Skip over navigation
Article by Lynne McClure
Primary teachers know that fluency in computation is important. Recent national initiatives have emphasised this - for example in the Numeracy Framework where mental calculation strategies were given new prominence. Most schools now have a calculation policy which includes both mental and written methods. So what's new in the new National Curriculum?
 Our KS1 tasks all focus on additive facts, skills and reasoning.
Our KS1 tasks all focus on additive facts, skills and reasoning.
Pairs of Numbers supports addition of single-digit numbers with an opportunity to generalise patterns and explain them.
We love Strike it Out which has lots of opportunities to recall addition and subtraction facts - many, many more than the children would do on a worksheet - and some conjecturing and convincing available to those who are already reasonably fluent.
Totality supports addition and subtraction of chains of single digits, but to be successful children need to look ahead and do some strategic thinking.
Or search by topic
Number and algebra
Geometry and measure
Probability and statistics
Working mathematically
Advanced mathematics
For younger learners
Age 5 to 11
Published 2014 Revised 2019
Developing Number Fluency - What, Why and How
Primary teachers know that fluency in computation is important. Recent national initiatives have emphasised this - for example in the Numeracy Framework where mental calculation strategies were given new prominence. Most schools now have a calculation policy which includes both mental and written methods. So what's new in the new National Curriculum?
Fluency in the new National Curriculum
One of the three aims of the new curriculum states that pupils (of all ages, not just primary children) will: become fluent in the fundamentals of mathematics, including through varied and frequent practice with increasingly complex problems over time, so that pupils develop conceptual understanding and the ability to recall and apply knowledge rapidly and
accurately.
The problem is that many schools are already interpreting this as practice, practice, practice of formal algorithms. That implies a pretty rigid and boring mathematical diet and is not what is intended in the aims. Let's take a closer look at what being fluent actually means.
The problem is that many schools are already interpreting this as practice, practice, practice of formal algorithms. That implies a pretty rigid and boring mathematical diet and is not what is intended in the aims. Let's take a closer look at what being fluent actually means.
What is fluency?
The first thing to say is that fluency is not only about number - there are other areas of the curriculum where fluency is important. However it's probably sensible to acknowledge that number is by far the largest part of the primary curriculum, so in this article we'll concentrate on that. We're not the only nation to take a recent interest in this - in the US the new
standards have quite a lot to say about being fluent:
Students exhibit computational fluency when they demonstrate flexibility in the computational methods they choose, understand and can explain these methods, and produce accurate answers efficiently.
Russell (2000) spells this out in more detail and suggests that fluency consists of three elements:
Efficiency - this implies that children do not get bogged down in too many steps or lose track of the logic of the strategy. An efficient strategy is one that the student can carry out easily, keeping track of sub-problems and making use of intermediate results to solve the problem.
Accuracy depends on several aspects of the problem-solving process, among them careful recording, knowledge of number facts and other important number relationships, and double-checking results.
Flexibility requires the knowledge of more than one approach to solving a particular kind of problem, such as two-digit multiplication. Students need to be flexible in order to choose an appropriate strategy for the numbers involved, and also be able to use one method to solve a problem and another method to check the results.
So fluency demands more of students than memorising a single procedure - they need to understand why they are doing what they are doing and know when it is appropriate to use different methods.
Students exhibit computational fluency when they demonstrate flexibility in the computational methods they choose, understand and can explain these methods, and produce accurate answers efficiently.
Russell (2000) spells this out in more detail and suggests that fluency consists of three elements:
Efficiency - this implies that children do not get bogged down in too many steps or lose track of the logic of the strategy. An efficient strategy is one that the student can carry out easily, keeping track of sub-problems and making use of intermediate results to solve the problem.
Accuracy depends on several aspects of the problem-solving process, among them careful recording, knowledge of number facts and other important number relationships, and double-checking results.
Flexibility requires the knowledge of more than one approach to solving a particular kind of problem, such as two-digit multiplication. Students need to be flexible in order to choose an appropriate strategy for the numbers involved, and also be able to use one method to solve a problem and another method to check the results.
So fluency demands more of students than memorising a single procedure - they need to understand why they are doing what they are doing and know when it is appropriate to use different methods.
Why do children need to be fluent?
To the person without number sense, arithmetic is a bewildering territory in which any deviation from the known path may rapidly lead to being totally lost. Dowker (1992)
The phrase 'number sense' is often used to mean conceptual fluency - understanding place value and the relationships between operations. Children need to be both procedurally and conceptually fluent - they need to know both how and why. Children who engage in a lot of practice without understanding what they are doing often forget, or remember incorrectly, those procedures. Further, there is growing evidence that once students have memorised and practised procedures without understanding, they have difficulty learning later to bring meaning to their work (Hiebert, 1999).
Russell describes two instances where children had a good idea about number relationships and operations but failed to use these successfully in practice. I'm sure you can think of similar examples that you have seen.
Child A knew, when asked verbally, what 112 and 40 were, and she had strategies to work out the answer which indicated that she understood place value - add 40 onto 110 and then add on the extra 2. But when asked to do it as a written calculation, she remembered an algorithm which was to do with lining up the numbers - and she remembered it incorrectly.

Similarly Child B could work out 57 x 4 mentally using the knowledge that 57 is 50 and 7 and breaking down the calculation into 50 x 4 and adding on 7 x 4. But he had remembered a written algorithm which was to do with carrying a digit over - and he remembered it incorrectly. (Can you see what he did? He added the 2 to the 5 before multiplying it by 4.) Both children knew their written answers were not correct but were convinced they had used the right method (and you might wonder what instructions they rehearsed in their heads which led them to believe that).
On the other hand, conceptual fluency without procedural fluency can make the problem-solving process tortuous - children lose track of their thinking because they have to divert their energies into calculations which should be quick but aren't.
The phrase 'number sense' is often used to mean conceptual fluency - understanding place value and the relationships between operations. Children need to be both procedurally and conceptually fluent - they need to know both how and why. Children who engage in a lot of practice without understanding what they are doing often forget, or remember incorrectly, those procedures. Further, there is growing evidence that once students have memorised and practised procedures without understanding, they have difficulty learning later to bring meaning to their work (Hiebert, 1999).
Russell describes two instances where children had a good idea about number relationships and operations but failed to use these successfully in practice. I'm sure you can think of similar examples that you have seen.
Child A knew, when asked verbally, what 112 and 40 were, and she had strategies to work out the answer which indicated that she understood place value - add 40 onto 110 and then add on the extra 2. But when asked to do it as a written calculation, she remembered an algorithm which was to do with lining up the numbers - and she remembered it incorrectly.

Similarly Child B could work out 57 x 4 mentally using the knowledge that 57 is 50 and 7 and breaking down the calculation into 50 x 4 and adding on 7 x 4. But he had remembered a written algorithm which was to do with carrying a digit over - and he remembered it incorrectly. (Can you see what he did? He added the 2 to the 5 before multiplying it by 4.) Both children knew their written answers were not correct but were convinced they had used the right method (and you might wonder what instructions they rehearsed in their heads which led them to believe that).
On the other hand, conceptual fluency without procedural fluency can make the problem-solving process tortuous - children lose track of their thinking because they have to divert their energies into calculations which should be quick but aren't.
How can we support children in becoming fluent?
As with much of mathematics, the key to fluency is in making connections, and making them at the right time in a child's learning.
Manipulatives
We learn by moving from the concrete to the abstract and structured apparatus such as Dienes can be helpful for learning about place value or number bonds. However the meaning isn't in the manipulatives themselves - it has to be constructed by children over a period of time, through playing around with them and connecting them directly to mental and recorded calculation.
We learn by moving from the concrete to the abstract and structured apparatus such as Dienes can be helpful for learning about place value or number bonds. However the meaning isn't in the manipulatives themselves - it has to be constructed by children over a period of time, through playing around with them and connecting them directly to mental and recorded calculation.
Talking about their work
At NRICH we often say you can't do maths unless you talk maths. But the quality of the talk is important. It is not simply children sharing how they did a particular calculation, but describing why and how it worked, and how their method is the same or different to those of others. In other words, giving children opportunities to use those higher-level skills of comparing, explaining and justifying. Russell says 'The reason that one problem can be solved in multiple ways is that mathematics does not consist of isolated rules, but connected ideas. Being able to solve a problem in more than one way, therefore, reveals the ability and the predilection to make connections between and among mathematical areas and topics'.
Consolidation in meaningful contexts
By offering children practice in context we help them to make links between the types of situations that a particular strategy might suit. Russell calls this mathematical memory, which is different from just memorising. She says that important mathematical procedures cannot be "forgotten over the summer" because they are based in a web of connected ideas about fundamental mathematical relationships.
At NRICH we often say you can't do maths unless you talk maths. But the quality of the talk is important. It is not simply children sharing how they did a particular calculation, but describing why and how it worked, and how their method is the same or different to those of others. In other words, giving children opportunities to use those higher-level skills of comparing, explaining and justifying. Russell says 'The reason that one problem can be solved in multiple ways is that mathematics does not consist of isolated rules, but connected ideas. Being able to solve a problem in more than one way, therefore, reveals the ability and the predilection to make connections between and among mathematical areas and topics'.
Consolidation in meaningful contexts
By offering children practice in context we help them to make links between the types of situations that a particular strategy might suit. Russell calls this mathematical memory, which is different from just memorising. She says that important mathematical procedures cannot be "forgotten over the summer" because they are based in a web of connected ideas about fundamental mathematical relationships.
The fluency tasks in this feature
We've chosen these tasks because they give lots of opportunities to practise core facts and skills, but in a much more interesting context than this usually happens. We like to think of this as 'thoughtful practice'. They are all Low Threshold High Ceiling tasks because pretty well all children will be able to take part, but for the higher attaining there are lots of
opportunities to do some mathematical reasoning too. A much better way than death by a thousand worksheets - and very little marking either! Four of the six tasks are games, which means they can be re-visited many times, whereas the remainder are challenges for one-off use.
Lower primary
 Our KS1 tasks all focus on additive facts, skills and reasoning.
Our KS1 tasks all focus on additive facts, skills and reasoning.Pairs of Numbers supports addition of single-digit numbers with an opportunity to generalise patterns and explain them.
We love Strike it Out which has lots of opportunities to recall addition and subtraction facts - many, many more than the children would do on a worksheet - and some conjecturing and convincing available to those who are already reasonably fluent.
Totality supports addition and subtraction of chains of single digits, but to be successful children need to look ahead and do some strategic thinking.
Upper primary
Our KS2 tasks all focus on multiplicative facts, skills and reasoning.
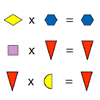 Shape Times Shape supports recall of multiplication facts but introduces some tricky ideas about 1 and 0 so that children need to do some logical thinking.
Shape Times Shape supports recall of multiplication facts but introduces some tricky ideas about 1 and 0 so that children need to do some logical thinking.
Mystery Matrix is useful because it focuses on working backwards with some mathematical reasoning needed, too.
Four Go also requires some backwards thinking - but to be successful and win the game children need to work strategically too.
We hope your pupils enjoy these challenges. Don't forget to encourage them to submit solutions to our live problems.
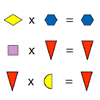 Shape Times Shape supports recall of multiplication facts but introduces some tricky ideas about 1 and 0 so that children need to do some logical thinking.
Shape Times Shape supports recall of multiplication facts but introduces some tricky ideas about 1 and 0 so that children need to do some logical thinking.Mystery Matrix is useful because it focuses on working backwards with some mathematical reasoning needed, too.
Four Go also requires some backwards thinking - but to be successful and win the game children need to work strategically too.
We hope your pupils enjoy these challenges. Don't forget to encourage them to submit solutions to our live problems.
Other NRICH articles to read
Low Threshold High Ceiling - An IntroductionCreating a Low Threshold High Ceiling Classroom
Early Number Sense
Place Value - The Ten-ness of Ten
Early Number Sense
Place Value - The Ten-ness of Ten
References
Dowker, A. (1992). Computational strategies of professional mathematicians. Journal for Research in Mathematics Education, 23 (1), 45-55.
Hiebert, J. (1999). Relationships between research and the NCTM Standards.Journal for Research in Mathematics Education, 30 (1), 3-19.
National Council of Teachers of Mathematics. (2000). Principles and Standards for School Mathematics. Reston, VA: NCTM.
Russell, Susan Jo. (May, 2000). Developing Computational Fluency with Whole Numbers in the Elementary Grades. In Ferrucci, Beverly J. and Heid, M. Kathleen (eds). Millenium Focus Issue: Perspectives on Principles and Standards. The New England Math Journal. Volume XXXII, Number 2. Keene, NH: Association of Teachers of Mathematics in New England. Pages 40-54.
Here is a PDF version of this article.
Hiebert, J. (1999). Relationships between research and the NCTM Standards.Journal for Research in Mathematics Education, 30 (1), 3-19.
National Council of Teachers of Mathematics. (2000). Principles and Standards for School Mathematics. Reston, VA: NCTM.
Russell, Susan Jo. (May, 2000). Developing Computational Fluency with Whole Numbers in the Elementary Grades. In Ferrucci, Beverly J. and Heid, M. Kathleen (eds). Millenium Focus Issue: Perspectives on Principles and Standards. The New England Math Journal. Volume XXXII, Number 2. Keene, NH: Association of Teachers of Mathematics in New England. Pages 40-54.
Here is a PDF version of this article.

