Skip over navigation

Or search by topic
Number and algebra
Geometry and measure
Probability and statistics
Working mathematically
Advanced mathematics
For younger learners
Networks and Nodes
Age 7 to 11
Challenge Level 





- Problem
- Getting Started
- Student Solutions
- Teachers' Resources
Why do this problem?
This problem makes a good whole class activity as it is suitable for all attainment levels but includes an element of logical thinking to challenge the highest attainers. It fits within the topic of topology which is not strictly within the curriulum but has lots of links to the language of shape and
space.
Possible approach
Ask a child to come to the board/whiteboard and draw a simple line picture or design without taking their pen off the surface. Change the colour of the pen and ask another child to come and copy, or go over, what the first child did. Ask a few simple questions such as "How did they know where to start?", "Did they remember or was there a clue in the way it was drawn?", "Would it work
starting at a different place (node)?".
Give the children a little time to work in pairs doing the same activity - one draws a network and the other has to find a route through it without taking their pen off the paper. Again, different coloured pens are helpful. Ask them the same sorts of questions to draw out any method they have found.
Offer the networks in the problem (you could print off this sheet of the diagrams) and ask the children to work by themselves or in pairs to find a unicursal (continuous) route for each one. Challenge them to find more than one route, marking each with arrows as indicated in the problem and showing at which node they started.
Now offer the challenge - can they draw a network that cannot be traced in one go? If they think they have, suggest that they draw it and post it somewhere central for others in the class to take up the challenge. That may be enough activity for most of the class, but the key questions to ask those who want to take it further are:
Is there a way of telling which networks can, and which can't, be traced?
Is there a way to tell which node is a starting node?
Key questions
If we can do it, does it matter where we start?
Is there a node we can start at that won't work?
How do you know?
Possible extension
Is there a way of telling which networks can, and which can't, be traced? Is there a way to tell which node is a starting node? Listen for explanations which describe going in and out of nodes. You might want to offer the well known The Bridges of Konigsberg problem as a further extension..
Possible support
All children will be able to try these activities. For those children who do need support, indicate the starting place.
You may also like
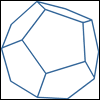
Redblue
Investigate the number of paths you can take from one vertex to another in these 3D shapes. Is it possible to take an odd number and an even number of paths to the same vertex?
Hamilton's Puzzle
I start my journey in Rio de Janeiro and visit all the cities as Hamilton described, passing through Canberra before Madrid, and then returning to Rio. What route could I have taken?
Sprouts Explained
This article invites you to get familiar with a strategic game called "sprouts". The game is simple enough for younger children to understand, and has also provided experienced mathematicians with significant food for thought.

