Skip over navigation
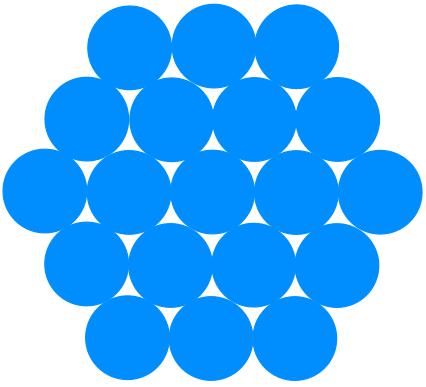
Here are some circles which are coins, counters, and rings. I used 10 coins, 19 counters, and 12 rings.
I wonder how many coins, counters, and rings I would need to make the next size up for each?
What is your idea of the 'next size up'?
My shapes are a triangle, a hexagon, and a rectangle.
What shapes could you make by putting circles together?
How many would you use?
How many would you need for the next size up?
This activity was initially developed for Wild Maths, our sister site, to encourage learners to be creative mathematicians. Mathematics is certainly a creative subject. It involves spotting patterns, making connections, finding new ways of looking at things and using what you already know in new contexts. Creative mathematicians play around with
examples, draw pictures, have the courage to experiment and ask good questions. (Wild Maths is aimed at individual learners, rather than teachers so the notes below only appear on NRICH.)
This activity may be used to encourage pupils to ask "I wonder what would happen it . . ." and use their curiosity to take things further. If you are interested in pursuing Curiosity further then see the Further Note at the bottom of this page.
This is really an adventure in arranging circles, counting and deciding what comes next.
Use some big circles (I used paper plates!) and start to make the triangle shape with all the children sitting around watching. After using about eight, ask a pupil to come and suggest where the next should go and then another pupil for the next etc. (You might need to consider the order in which you place the circles and whether this affects what suggestions the pupils have.)
You could then show learners the other two arrangements and pose the questions as in the problem.
What have you decided to do with your circles?
What would the shape before yours look like (bigger/smaller etc.)?
Encourage learners to use other shapes like equilateral triangles, regular hexagons, etc.
You may be interested in the following talks given by Professor Susan Engels, which focus on encouraging curiosity and are available on YouTube:
The Rise and Fall of Curiosity - the extract from 23.50 to 37.15 on adult encouragement and teacher behaviour is particularly worth viewing
The Hungry Mind: The Origins of Curiosity - the extract from 8.22 to 12.29 on children asking questions is especially useful.
Circles, Circles
Age 5 to 11
Challenge Level 
Circles,Circles
Here are some circles which are coins, counters, and rings. I used 10 coins, 19 counters, and 12 rings.
I wonder how many coins, counters, and rings I would need to make the next size up for each?
What is your idea of the 'next size up'?
My shapes are a triangle, a hexagon, and a rectangle.
What shapes could you make by putting circles together?
How many would you use?
How many would you need for the next size up?
Why do this problem?
This activity was initially developed for Wild Maths, our sister site, to encourage learners to be creative mathematicians. Mathematics is certainly a creative subject. It involves spotting patterns, making connections, finding new ways of looking at things and using what you already know in new contexts. Creative mathematicians play around with
examples, draw pictures, have the courage to experiment and ask good questions. (Wild Maths is aimed at individual learners, rather than teachers so the notes below only appear on NRICH.)This activity may be used to encourage pupils to ask "I wonder what would happen it . . ." and use their curiosity to take things further. If you are interested in pursuing Curiosity further then see the Further Note at the bottom of this page.
Possible approach
This is really an adventure in arranging circles, counting and deciding what comes next.Use some big circles (I used paper plates!) and start to make the triangle shape with all the children sitting around watching. After using about eight, ask a pupil to come and suggest where the next should go and then another pupil for the next etc. (You might need to consider the order in which you place the circles and whether this affects what suggestions the pupils have.)
You could then show learners the other two arrangements and pose the questions as in the problem.
Key questions
What have you decided to do with your circles?What would the shape before yours look like (bigger/smaller etc.)?
Possible extension
Encourage learners to use other shapes like equilateral triangles, regular hexagons, etc.
Further note
You may be interested in the following talks given by Professor Susan Engels, which focus on encouraging curiosity and are available on YouTube:The Rise and Fall of Curiosity - the extract from 23.50 to 37.15 on adult encouragement and teacher behaviour is particularly worth viewing
The Hungry Mind: The Origins of Curiosity - the extract from 8.22 to 12.29 on children asking questions is especially useful.
You may also like
Let's Investigate Triangles
Vincent and Tara are making triangles with the class construction set. They have a pile of strips of different lengths. How many different triangles can they make?
