Skip over navigation
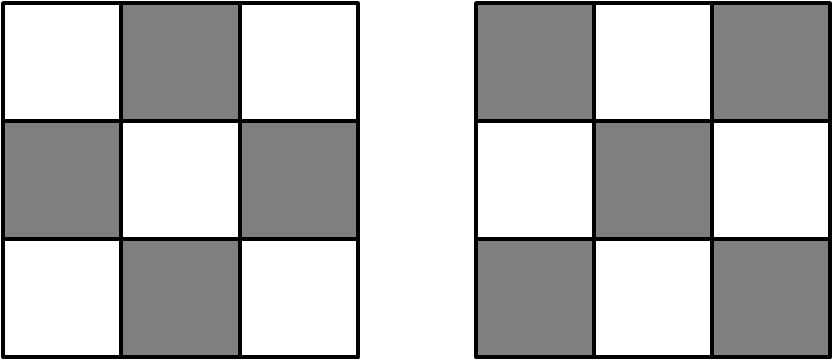Consider the different faces of this cuboid. The faces on the ends of the cuboid will look like these diagrams on the right. Between them they have $9$ black squares and $9$ white squares.
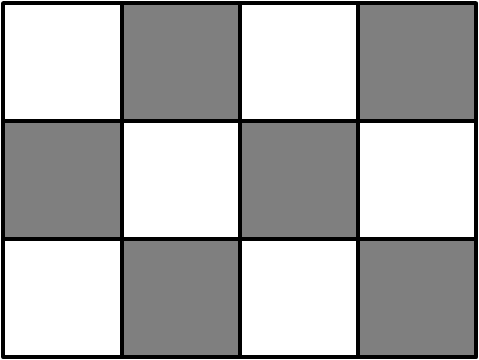The other four faces all look like the diagram to the right. This contains $6$ white squares and $6$ black squares.
Therefore, between the four faces there are $6 \times 4 = 24$ white squares and $6 \times 4 = 24$ black squares.
Therefore, overall there are $9+24=33$ white squares and $9+24=33$ black squares, so $\frac{1}{2}$ of the squares are coloured black.
Chequered Cuboid
Age 11 to 14
ShortChallenge Level 
Consider the different faces of this cuboid. The faces on the ends of the cuboid will look like these diagrams on the right. Between them they have $9$ black squares and $9$ white squares. The other four faces all look like the diagram to the right. This contains $6$ white squares and $6$ black squares.Therefore, between the four faces there are $6 \times 4 = 24$ white squares and $6 \times 4 = 24$ black squares.
Therefore, overall there are $9+24=33$ white squares and $9+24=33$ black squares, so $\frac{1}{2}$ of the squares are coloured black.
This problem is taken from the UKMT Mathematical Challenges.
You can find more short problems, arranged by curriculum topic, in our short problems collection.
You may also like
Consecutive Numbers
An investigation involving adding and subtracting sets of consecutive numbers. Lots to find out, lots to explore.
Days and Dates
Investigate how you can work out what day of the week your birthday will be on next year, and the year after...