Semicircle Distance

Let the length of the rectangle be $x$. Then by considering the area of the whole rectangle, we can find $x$.

The area of the whole rectangle can be found using length $\times$ width = $10x$, as shown below.
 

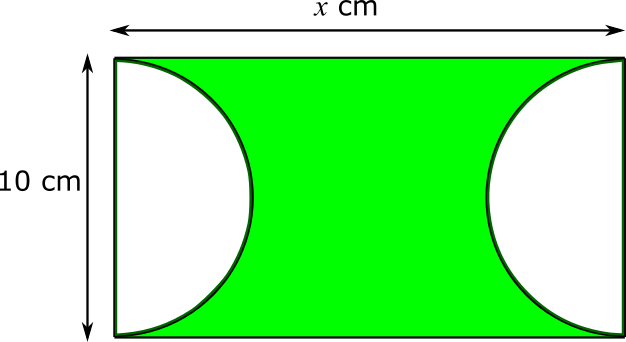
This must be equal to the shaded area added to the total area of the two semicircles. The radius of each semicircle must be half of 10 cm, which is 5 cm.
 

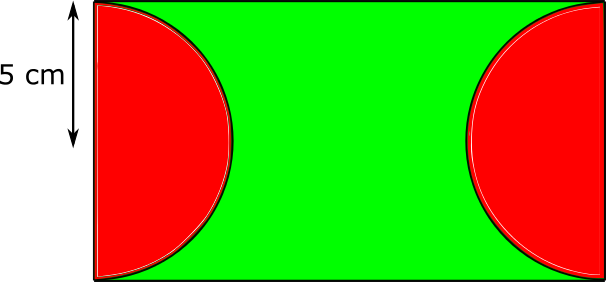
So the red area is equal to $\pi\times 5^2$ cm$^2=25\pi$ cm$^2$, because the two red semicircles could be stuck together to make a circle of radius 5 cm.

That means that the total area of the rectangle must be $125 + 25\pi$ cm$^2$, so $10x=125+25\pi$, so $x=\dfrac{125+25\pi}{10}=12.5+2.5\pi$.

Now, as shown below, the shortest distance can be found using $x$.

  

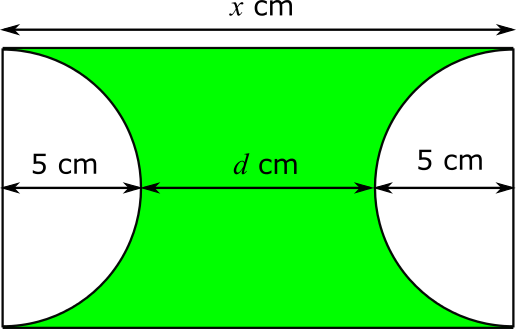
$d=x-5-5=x-10$, so $d=12.5+2.5\pi-10=2.5+2.5\pi$ cm.