Skip over navigation
Alternatively, we could use tiles that contain fractions of hexagons which are easy to identify, like the ones shown below. Underneath each tiling is the number of white and black hexagons per tile. In each case, the proportion of each tile that is black, given by $\dfrac{\text{number of black hexagons}}{\text{total number of hexagons}},$ is $\frac{1}{8}.$
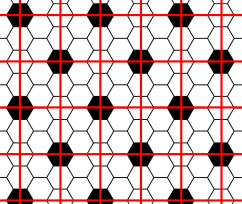
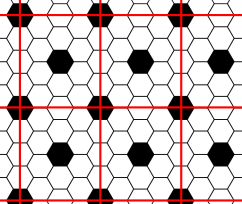
$2+ \frac{2}{2}+\frac{2}{4}=3\frac{1}{2}$ white hexagons and $10+\frac{8}{2}=14$ white hexagons and
$\frac{2}{4}=\frac{1}{2}$ black hexagon per tile $1+\frac{4}{4}=2$ black hexagons per tile
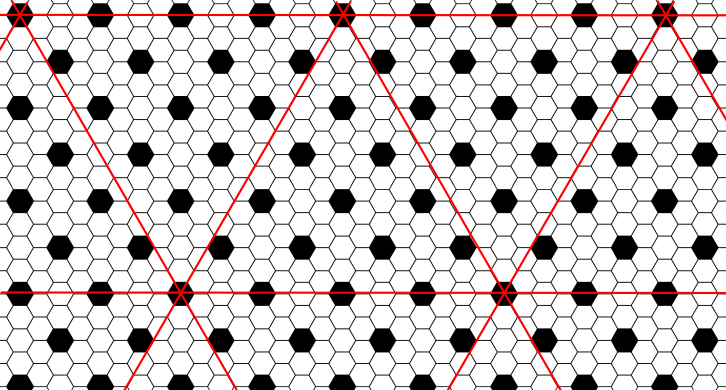
$75+\frac{18}{2}=84$ white hexagons and $10+\frac{3}{2}+\frac{3}{3}=12$ black hexagons per tile
Tricky Tessellations
Age 11 to 14
ShortChallenge Level 
If we can split the plane into a tesselation of identical tiles, then the proportion of each tile that is black will be the same as the proportion of the plane which is black. So we will count the proportion of each tile that is black.
We could use tiles that contain only whole hexagons, like the ones shown below. In each case, each tile contains 7 white hexagons and one black hexagon, so $\frac{1}{8}$ of each tile is black, so $\frac{1}{8}$ of the plane is black.
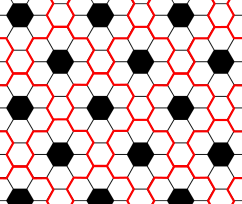
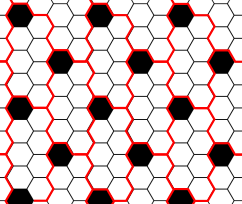
We could use tiles that contain only whole hexagons, like the ones shown below. In each case, each tile contains 7 white hexagons and one black hexagon, so $\frac{1}{8}$ of each tile is black, so $\frac{1}{8}$ of the plane is black.
Alternatively, we could use tiles that contain fractions of hexagons which are easy to identify, like the ones shown below. Underneath each tiling is the number of white and black hexagons per tile. In each case, the proportion of each tile that is black, given by $\dfrac{\text{number of black hexagons}}{\text{total number of hexagons}},$ is $\frac{1}{8}.$
$2+ \frac{2}{2}+\frac{2}{4}=3\frac{1}{2}$ white hexagons and $10+\frac{8}{2}=14$ white hexagons and
$\frac{2}{4}=\frac{1}{2}$ black hexagon per tile $1+\frac{4}{4}=2$ black hexagons per tile
$75+\frac{18}{2}=84$ white hexagons and $10+\frac{3}{2}+\frac{3}{3}=12$ black hexagons per tile
You can find more short problems, arranged by curriculum topic, in our short problems collection.
You may also like
Consecutive Numbers
An investigation involving adding and subtracting sets of consecutive numbers. Lots to find out, lots to explore.
Days and Dates
Investigate how you can work out what day of the week your birthday will be on next year, and the year after...