Skip over navigation
Answer: 35 km/h
Using a speed-time graph


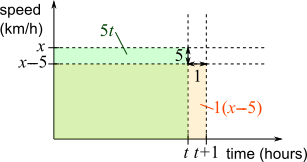
\therefore 5t = x-5 and xt=210
5t = x-5\Rightarrow t = \frac{x-5}5
xt = 210 \Rightarrow x\left(\frac{x-5}5\right)=210\\ \hspace{18mm}\Rightarrow x(x-5)=1050\\ \hspace{18mm}\Rightarrow x^2-5x-1050=0
The quadratic formula gives: \begin{align}x&=\frac{5\pm\sqrt{25--4200}}2\\ &=\frac{5\pm\sqrt{169\times25}}2\\ &=\frac{5\pm65}2\end{align}
Using time = distance \div speed
Average speed over 210 km is x km/h, then time is \dfrac{210}x hours.
Planned average speed is x-5 km/h, time at planned speed \dfrac{210}{x-5} hours.
1 hour earlier than planned, so \dfrac{210}x is 1 less than \dfrac{210}{x-5}, so \begin{align}&\frac{210}{x}=\frac{210}{x-5}-1\\ \hspace{1mm}\\ \Rightarrow&210=\frac{210x}{x-5}-x\\\hspace{1mm}\\ \Rightarrow&210(x-5)=210x-x(x-5)\\ \Rightarrow&210x-1050=210x-x^2+5x\\ \Rightarrow&x^2-5x-1050=0\\ \Rightarrow&(x+30)(x-35)=0\end{align}
Using distance = speed \times time
If Chris completed the journey in t hours, distance =x\times t.
Chris intended to take t+1 hours.
He intended to travel 5 km/h slower, so at an average speed of x-5 km/h.
Distance =(x-5)(t+1)=xt-5t+x-5
Distances are the same so xt = xt-5t+x-5\Rightarrow0=-5t+x-5\Rightarrow x=5t+5
And xt=210 so (5t+5)t=210\Rightarrow (t+1)t=42 so t, t+1 could be 6,7 (or -6, -7)
x=5\times6+5=35

Or search by topic
Number and algebra
Geometry and measure
Probability and statistics
Working mathematically
Advanced mathematics
For younger learners
Day Out
Age 14 to 16
ShortChallenge Level 





- Problem
- Solutions
Answer: 35 km/h
Using a speed-time graph



\therefore 5t = x-5 and xt=210
5t = x-5\Rightarrow t = \frac{x-5}5
xt = 210 \Rightarrow x\left(\frac{x-5}5\right)=210\\ \hspace{18mm}\Rightarrow x(x-5)=1050\\ \hspace{18mm}\Rightarrow x^2-5x-1050=0
The quadratic formula gives: \begin{align}x&=\frac{5\pm\sqrt{25--4200}}2\\ &=\frac{5\pm\sqrt{169\times25}}2\\ &=\frac{5\pm65}2\end{align}
So x=35
Using time = distance \div speed
Average speed over 210 km is x km/h, then time is \dfrac{210}x hours.
Planned average speed is x-5 km/h, time at planned speed \dfrac{210}{x-5} hours.
1 hour earlier than planned, so \dfrac{210}x is 1 less than \dfrac{210}{x-5}, so \begin{align}&\frac{210}{x}=\frac{210}{x-5}-1\\ \hspace{1mm}\\ \Rightarrow&210=\frac{210x}{x-5}-x\\\hspace{1mm}\\ \Rightarrow&210(x-5)=210x-x(x-5)\\ \Rightarrow&210x-1050=210x-x^2+5x\\ \Rightarrow&x^2-5x-1050=0\\ \Rightarrow&(x+30)(x-35)=0\end{align}
So x=-30 or x=35. Since x>0, x=35.
Using distance = speed \times time
If Chris completed the journey in t hours, distance =x\times t.
Chris intended to take t+1 hours.
He intended to travel 5 km/h slower, so at an average speed of x-5 km/h.
Distance =(x-5)(t+1)=xt-5t+x-5
Distances are the same so xt = xt-5t+x-5\Rightarrow0=-5t+x-5\Rightarrow x=5t+5
And xt=210 so (5t+5)t=210\Rightarrow (t+1)t=42 so t, t+1 could be 6,7 (or -6, -7)
x=5\times6+5=35
You can find more short problems, arranged by curriculum topic, in our short problems collection.
You may also like

Percentage Unchanged
If the base of a rectangle is increased by 10% and the area is unchanged, by what percentage is the width decreased by ?

