Skip over navigation
Answer: 1300
Using letters to represent the 'parts'
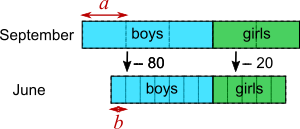
Boys: 3a-80=7b \times2 gives 6a-160=14b
Girls: 2a - 20 = 5b \times3 gives 6a-60=15b
Subtract: (6a-60)-(6a-160)=15b-14b
100=b
In June there were 7b + 5b = 12b = 1200 students
In September there were 1200 + 20 + 80 = 1300 students
Using a letter to represent the number of students in September
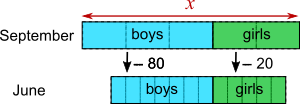
September: \frac35x boys out of x students
June: \frac35x-80 boys is \frac7{12} of x-100 students
\begin{align}\dfrac{\tfrac35x-80}{x-100}&=\dfrac7{12}\\ \Rightarrow 12\times\left(\tfrac35x-80\right)&=7\times\left(x-100\right)\\ \Rightarrow 12\times\left(3x-4000\right)&=35\times\left(x-100\right)\\ \Rightarrow 36x-4800&=35x-3500\\ \Rightarrow x&=1300\end{align}
Using letters to represent the numbers of boys and girls in September
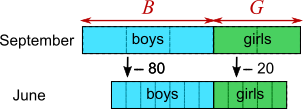
September: B:G is 3:2, and so \frac B3=\frac G2\Rightarrow B=\frac{3G}2
June: B-80:G-20 is 7:5, so \frac{B-80}7=\frac{G-20}5
\Rightarrow 5(B-80)=7(G-20)
\Rightarrow 5B-260=7G
Substitute B=\frac{3G}2: \begin{align}5\tfrac{3G}2-260&=7G\\ \Rightarrow 15G-520&=14G\\ \Rightarrow G &= 520\end{align}
So B=\frac{3G}2=780 and in September there were 520 + 780 =1300 students.

Or search by topic
Number and algebra
Geometry and measure
Probability and statistics
Working mathematically
Advanced mathematics
For younger learners
Boys and Girls
Age 14 to 16
ShortChallenge Level 





- Problem
- Solutions
Answer: 1300
Using letters to represent the 'parts'

Boys: 3a-80=7b \times2 gives 6a-160=14b
Girls: 2a - 20 = 5b \times3 gives 6a-60=15b
Subtract: (6a-60)-(6a-160)=15b-14b
100=b
In June there were 7b + 5b = 12b = 1200 students
In September there were 1200 + 20 + 80 = 1300 students
Using a letter to represent the number of students in September

September: \frac35x boys out of x students
June: \frac35x-80 boys is \frac7{12} of x-100 students
\begin{align}\dfrac{\tfrac35x-80}{x-100}&=\dfrac7{12}\\ \Rightarrow 12\times\left(\tfrac35x-80\right)&=7\times\left(x-100\right)\\ \Rightarrow 12\times\left(3x-4000\right)&=35\times\left(x-100\right)\\ \Rightarrow 36x-4800&=35x-3500\\ \Rightarrow x&=1300\end{align}
Using letters to represent the numbers of boys and girls in September

September: B:G is 3:2, and so \frac B3=\frac G2\Rightarrow B=\frac{3G}2
June: B-80:G-20 is 7:5, so \frac{B-80}7=\frac{G-20}5
\Rightarrow 5(B-80)=7(G-20)
\Rightarrow 5B-260=7G
Substitute B=\frac{3G}2: \begin{align}5\tfrac{3G}2-260&=7G\\ \Rightarrow 15G-520&=14G\\ \Rightarrow G &= 520\end{align}
So B=\frac{3G}2=780 and in September there were 520 + 780 =1300 students.
You can find more short problems, arranged by curriculum topic, in our short problems collection.
You may also like

Tummy Ache
Timmy, Tammy and Tommy all go to the doctors. In what order do they arrive at the surgery?

