Skip over navigation
Answer: On the hare's 13th lap
How far the tortoise goes for each hare lap using speed distance time
Hare 15 km in 1 hour
1 km in 4 minutes
250 m in 1 minute
300 m in 1\frac15 minutes
Tortoise 13.8 km in 1 hour
13800 m in 1 hour
13800\div60 m in 1 minute
230 m in 1 minute
230 + \frac{230}5 = 276 m in 1\frac15 minutes
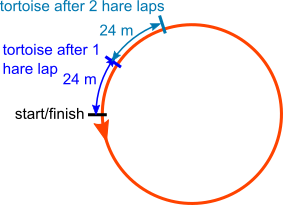
The tortoise loses 24 m every time the hare runs a lap
24 \times 12 = 288 \lt 300
24 \times 13 \gt 300

So the tortoise does not reach the starting point on the hare's 13th lap
So the hare passes the tortoise on the hare's 13th lap.
How far the tortoise goes for each hare lap using inverse proportion
hare speed : tortoise speed is 15 : 13.8
hare distance : tortoise distance is 13.8:15, which is equivalent to 23:25
So every time the hare runs a lap, the tortoise runs \frac{23}{35} of a lap

\frac2 {25}\times12 \lt 1
\frac2{25}\times13\gt1
So the tortoise does not reach the starting point on the hare's 13th lap
So the hare passes the tortoise on the hare's 13th lap.
After how long has the hare travelled 300 m further than the tortoise?
t time (in hours)
hare distance =15t
tortoise distance =13.8t
hare first passes tortoise when hare is 300 metres = 0.3 km ahead \begin{align} &15t-13.8t=0.3\\ \Rightarrow &1.2t = 0.3\\ \Rightarrow & t= \tfrac{0.3}{1.2}=\tfrac14\end{align}
3600 m = 12 laps
3900 m =13 laps
So the hare was on her thirteenth lap.
Working out how long it takes each runner to run k laps
Hare runs 15km in 1 hour
15000 m in 1 hour
300 m in \frac{1}{50} hours.
Tortoise runs 13.8 km in 1 hour
13800 m in 1 hour
300 m in \frac{1}{46} hours.
Hare runs k laps in \frac{1}{50}\times k hours.
When the hare passes the tortoise, the tortoise has only run k-1 laps.
Tortoise runs k-1 laps in \frac{1}{46}\times(k-1) hours. \begin{align}&\tfrac{1}{50}k=\tfrac{1}{46}(k-1)\\ \Rightarrow&46k=50(k-1)\\ \Rightarrow&46k=50k-50\\ \Rightarrow &40=4k\\ \Rightarrow &12.5=k\end{align}

Or search by topic
Number and algebra
Geometry and measure
Probability and statistics
Working mathematically
Advanced mathematics
For younger learners
Laps
Age 14 to 16
ShortChallenge Level 





- Problem
- Solutions
Answer: On the hare's 13th lap
How far the tortoise goes for each hare lap using speed distance time
Hare 15 km in 1 hour
1 km in 4 minutes
250 m in 1 minute
300 m in 1\frac15 minutes
Tortoise 13.8 km in 1 hour
13800 m in 1 hour
13800\div60 m in 1 minute
230 m in 1 minute
230 + \frac{230}5 = 276 m in 1\frac15 minutes
The tortoise loses 24 m every time the hare runs a lap

24 \times 12 = 288 \lt 300
24 \times 13 \gt 300

So the tortoise does not reach the starting point on the hare's 13th lap
So the hare passes the tortoise on the hare's 13th lap.
How far the tortoise goes for each hare lap using inverse proportion
hare speed : tortoise speed is 15 : 13.8
hare distance : tortoise distance is 13.8:15, which is equivalent to 23:25
So every time the hare runs a lap, the tortoise runs \frac{23}{35} of a lap

\frac2 {25}\times12 \lt 1
\frac2{25}\times13\gt1

So the tortoise does not reach the starting point on the hare's 13th lap
So the hare passes the tortoise on the hare's 13th lap.
After how long has the hare travelled 300 m further than the tortoise?
t time (in hours)
hare distance =15t
tortoise distance =13.8t
hare first passes tortoise when hare is 300 metres = 0.3 km ahead \begin{align} &15t-13.8t=0.3\\ \Rightarrow &1.2t = 0.3\\ \Rightarrow & t= \tfrac{0.3}{1.2}=\tfrac14\end{align}
When t=\frac14 the hare has run 15\times\frac14 = 3\frac34 km or 3750 metres
3600 m = 12 laps
3900 m =13 laps
So the hare was on her thirteenth lap.
Working out how long it takes each runner to run k laps
Hare runs 15km in 1 hour
15000 m in 1 hour
300 m in \frac{1}{50} hours.
Tortoise runs 13.8 km in 1 hour
13800 m in 1 hour
300 m in \frac{1}{46} hours.
Hare runs k laps in \frac{1}{50}\times k hours.
When the hare passes the tortoise, the tortoise has only run k-1 laps.
Tortoise runs k-1 laps in \frac{1}{46}\times(k-1) hours. \begin{align}&\tfrac{1}{50}k=\tfrac{1}{46}(k-1)\\ \Rightarrow&46k=50(k-1)\\ \Rightarrow&46k=50k-50\\ \Rightarrow &40=4k\\ \Rightarrow &12.5=k\end{align}
So the hare has run 12.5 laps and is on her thirteenth when she passes the tortoise.
You can find more short problems, arranged by curriculum topic, in our short problems collection.
You may also like

Percentage Unchanged
If the base of a rectangle is increased by 10% and the area is unchanged, by what percentage is the width decreased by ?

