Skip over navigation
Using a speed-time graph
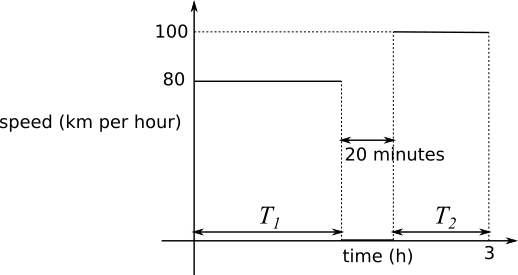
time: T_{1}+T_{2}=3 hours-20 minutes =2\frac23 hours
Distance on a speed-time graph is given by the area under the graph, so: \begin{align} 80T_{1}+100T_{2}&=250\\ \Rightarrow 80(T_1+T_2) + 20T_2&=250\\ \Rightarrow 80\times 2\tfrac23+20T_2&=250\\ \Rightarrow 8\times \tfrac83 + 2T_2 &=25\\ \Rightarrow 2T_2&= \tfrac{75}3-\tfrac{64}3\\ \Rightarrow T_2 &= \tfrac{11}6\end{align}
Using the speed-distance-time relationship
Distance = speed \times time
Distance travelled before the petrol stop is 80\times T_{1}
Distance travelled after the petrol stop is 100\times T_{2}
Total distance is 250 km so 80T_{1}+100T_{2}=250.
Total time is 3 hours. 20 minutes =\frac13 hour, so T_{1}+T_{2}=2\frac23.
T_{1}+T_{2}=2\frac23\Rightarrow T_1 = \frac83 - T_2
Substitute into other equation: \begin{align} 80 \left(\tfrac83 - T_2\right) +100T_2 &= 250\\ \Rightarrow \tfrac {64}3 -8T_2 +10T_2 &=25\\ \Rightarrow \tfrac{64}3 + 2T_2&=\tfrac{75}3\\ \Rightarrow T_2&=\tfrac{11}{6}\end{align}

Or search by topic
Number and algebra
Geometry and measure
Probability and statistics
Working mathematically
Advanced mathematics
For younger learners
Petrol Stop
Age 14 to 16
ShortChallenge Level 





- Problem
- Solutions
Answer: 1 hour and 10 minutes
Using a distance-time graph

Gradient represents speed so gradients are 80 and 100
So vertical distances are 80T_1 and 100T_2
time: T_{1}+T_{2}=3 hours-20 minutes =2\frac23 hours
distance: 80T_{1}+100T_{2}=250\Rightarrow 8T_1+10T_2=25
T_1+T_2 = 2\frac23 \times8 gives 8T_1+8T_2=16\tfrac{16}3=21\frac13
Subtracting, \begin{align}(8T_1+10T_2) - (8T_1+8T_2)&=25 - 21\tfrac13\\
2T_2&=3\tfrac23=\tfrac{11}3\\
T_2&=\tfrac{11}6\end{align}
\frac{11}6 hours =1 hour and 50 minutes.
Using a speed-time graph

time: T_{1}+T_{2}=3 hours-20 minutes =2\frac23 hours
Distance on a speed-time graph is given by the area under the graph, so: \begin{align} 80T_{1}+100T_{2}&=250\\ \Rightarrow 80(T_1+T_2) + 20T_2&=250\\ \Rightarrow 80\times 2\tfrac23+20T_2&=250\\ \Rightarrow 8\times \tfrac83 + 2T_2 &=25\\ \Rightarrow 2T_2&= \tfrac{75}3-\tfrac{64}3\\ \Rightarrow T_2 &= \tfrac{11}6\end{align}
\frac{11}6 hours =1 hour and 50 minutes.
Using the speed-distance-time relationship
Distance = speed \times time
Distance travelled before the petrol stop is 80\times T_{1}
Distance travelled after the petrol stop is 100\times T_{2}
Total distance is 250 km so 80T_{1}+100T_{2}=250.
Total time is 3 hours. 20 minutes =\frac13 hour, so T_{1}+T_{2}=2\frac23.
T_{1}+T_{2}=2\frac23\Rightarrow T_1 = \frac83 - T_2
Substitute into other equation: \begin{align} 80 \left(\tfrac83 - T_2\right) +100T_2 &= 250\\ \Rightarrow \tfrac {64}3 -8T_2 +10T_2 &=25\\ \Rightarrow \tfrac{64}3 + 2T_2&=\tfrac{75}3\\ \Rightarrow T_2&=\tfrac{11}{6}\end{align}
\frac{11}6 hours =1 hour and 50 minutes.
You can find more short problems, arranged by curriculum topic, in our short problems collection.
You may also like

Tummy Ache
Timmy, Tammy and Tommy all go to the doctors. In what order do they arrive at the surgery?

