Two Triangles

Using the isosceles triangle and the right-angled triangle


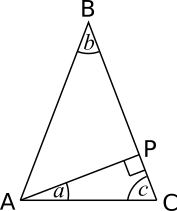
”‹From the right-angled triangle, $a+c+90=180$, so $a=90-c$.
From the isosceles triangle, $2c+b=180$, so $b=180-2c$.
But $180-2c=2(90-c)$, so $b=2a$.

Using this pair of triangles can also lead to simple simultaneous equations.


Using the two right-angled triangles
 
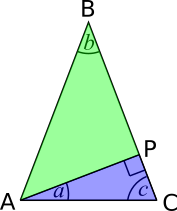
From the blue right-angled triangle, $a+c+90=180$, so $c=90-a$.
Similarly, the missing angle in the green right-angled triangle is $90-b$.
But the missing angle in the green triangle added to $a$ makes $c$.
So $a+90-b=90-a$, which rearranges to give $2a=b$.