Making Pathways

We have some blue, green and red paving slabs - twelve paving slabs of each colour.

There are three different sizes of slabs for each colour. All paving slabs are the same width. 

The blue paving slabs are: four of length 1, four of length 9 and four of length 24.

The green paving slabs are: four of length 1, four of length 7 and four of length 25.

The red paving slabs are: four of length 1, four of length 5 and four of length 29.

 

The paving slabs cannot be broken into smaller pieces. Every path in this challenge must be made out of only one colour of paving slab - we cannot have a mixture of colours in a path.

Challenge 1

If we want to make a path of length 18 in each colour, we might have:

Blue:   

9+9  

Green:

7+7+1+1+1+1

(which we count as the same solution as e.g. 7+1+1+7+1+1 )

Red:

5+5+5+1+1+1

(which we count as the same solution as e.g. 5+1+5+1+5+1 )

Can you make three paths of length 22, one in each of the three colours?
Next, try making three paths of length 40, one in each of the three colours.
Lastly, try making three paths of length 64, one in each of the three colours.

Challenge 2

In how many different ways can you make paths of length 75, using only one colour in each path?

Challenge 3

We would like to find eight consecutive lengths of path that can be made out of the blue, green and red paving slabs, where each of the eight lengths can be made out of each colour.

It would look something like this picture for the consecutive lengths 59, 60, 61, 62, 63, 64, 65 and 66:

 

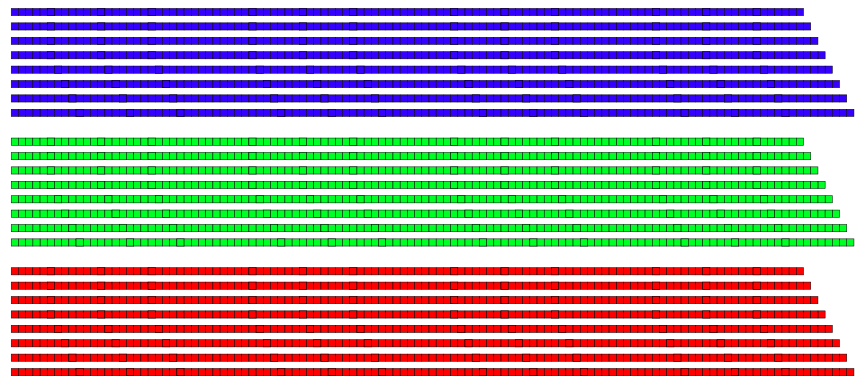
Unfortunately this is not a solution as it cannot be made without breaking some paving slabs up into smaller pieces.

Your challenge is to find eight consecutive lengths of path that can be made out of each colour separately.