Skip over navigation
In how many different ways can you halve an A4 sheet of paper?
You might start off with something like these two, for example:


Have a good explore and see what different ways you can find. Don't be afraid to be creative!
How will you know if they are halves?
This activity offers free exploration that can help youngsters with their development of the concepts associated with fractions. It provides a chance for meaningful mathematical discussion and sharing of current understanding, in addition to offering opportunities for challenging misconceptions.
This task is best introduced as succinctly as possible so that learners have the freedom to work in any way they wish. Make sure that pupils know that they have a choice in how they fold/cut/draw to show halves - the key is that they can justify that the two parts really are halves.
Here are some ways of halving that sometimes occur:
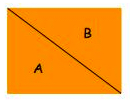
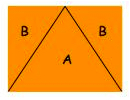
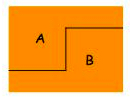
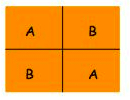

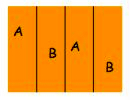
(Please note that the labelling of A and B is ours, not the children's.)
Some learners may be adventurous and use curves such as these, which produce interesting justifications as to how they are halves:
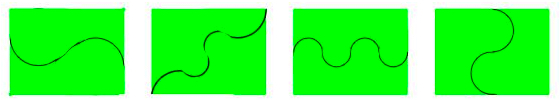
Talk is key for this task. Asking children to explain their ideas will help to reveal their understanding even if their constructions are not quite accurate. You can facilitate discussion about not all halves being symmetrical and it would also be fruitful to ask how learners think the task would have been affected if they had been given squared paper rather than plain.
Tell me about your halves.
How do you know this is a half?
Can you find some other ways of halving the paper?

Or search by topic
Number and algebra
Geometry and measure
Probability and statistics
Working mathematically
Advanced mathematics
For younger learners
Paper Halving
Age 5 to 11
Challenge Level 





- Problem
- Student Solutions
- Teachers' Resources
Paper Halving
In how many different ways can you halve an A4 sheet of paper?
You might start off with something like these two, for example:


Have a good explore and see what different ways you can find. Don't be afraid to be creative!
How will you know if they are halves?
Why do this problem?
This activity offers free exploration that can help youngsters with their development of the concepts associated with fractions. It provides a chance for meaningful mathematical discussion and sharing of current understanding, in addition to offering opportunities for challenging misconceptions.
Possible approach
This task is best introduced as succinctly as possible so that learners have the freedom to work in any way they wish. Make sure that pupils know that they have a choice in how they fold/cut/draw to show halves - the key is that they can justify that the two parts really are halves. Here are some ways of halving that sometimes occur:






(Please note that the labelling of A and B is ours, not the children's.)
Some learners may be adventurous and use curves such as these, which produce interesting justifications as to how they are halves:

Talk is key for this task. Asking children to explain their ideas will help to reveal their understanding even if their constructions are not quite accurate. You can facilitate discussion about not all halves being symmetrical and it would also be fruitful to ask how learners think the task would have been affected if they had been given squared paper rather than plain.
Key questions
Tell me about your halves.How do you know this is a half?
Can you find some other ways of halving the paper?
Possible extension
Repeat the same for thirds. (Yes thirds before quarters!)Possible support
There may be some pupils who need support folding, cutting and/or drawing as well as any measuring. It might be appropriate to pair children with different skills in this regard.You may also like
A Bowl of Fruit
Can you work out how many apples there are in this fruit bowl if you know what fraction there are?

