Parallelogram It

Adrian from the British School Al Khubairat in Syria described a strategy for beating the computer:

So, this bot was actually pretty easy to beat, as you could just start by placing your first triangle in the top left square, then the bot will go middle, then you could leave two spaces to the right and click the next square, then the bot will go just under the middle, then you could click the square right below the previous one, then it will go somewhere random, and finally you could go under the first square you clicked.
 

Ci Hui Minh Ngoc from Kelvin Grove State College (Brisbane) in Australia also described one way of winning the game:

1st player (Human) started with point at centre point. Then put 2nd point on either side of this centre point. Then these first two points are on one line and it forms one of the parallel sides of the Parallelogram.

The other two vertices, 3rd and 4th of parallelogram can be located on either sides (up or down) of 1st and 2nd points, also on the same line

Also, the 3rd and 4th points have the option to be on the left of 1st point or on the right side of the 2nd point, hence when computer (2nd player) block one of the option, 1st player can choose the other option and complete the parallelogram first.
 

Olivia from McCauley Catholic High Scool in England had a broader strategy:

I set up my next paralelogram within my first 3 moves so that if my next move was covered by the computer I could go somewhere else and win that way.
 

Dylan from Chesterton Community College in the UK had a strategy that adapted based on what the computer did:

The method I found does not work on a 3 by 3 grid as it is too small. It works on all larger grids. Using this method the player who starts will always win against the computer. Put your 1st dot on the centre point of an odd-sized grid or on one of the 4 centre points of an even-sized grid. Then put your 2nd dot on a vertical or horizontal line from your 1st dot and adjacent to the computer’s dot forming a right angle triangle between the 3 dots. Turn your screen so the computer’s next dot is at the bottom. Next place your 3rd dot two places vertically above your first dot. You are now in a winning position as you can make two different parallelograms with your next dot.
 

So is the game easier to win than Square It? Rishik explained why there are more winning shapes.

Although this game asks the player to form a parallelogram, the player has the option to also form a rectangle, square or rhombus as these shapes also share the exact same properties as a parallelogram and they may not appear as the same shape, but they are ‘technically’ a parallelogram. 
 

Ci Hui Minh Ngoc thought about who goes first:

1st Move Advantage: The 1st player can get into the centre point of the board and improve the chance of winning.
If computer is the 1st player, the computer would always choose the centre point and will always win.
If the 1st player is a human, it will depend on human's strategy. 
 

Rishik had some more general strategies and tips:

1. Similarly with the previous Square It game, the player should try to go first if given a choice as it allows them more opportunities to win and capturing the middle point is quite useful whilst playing. When, I played against the computer and placed my counter elsewhere, the computer always irrespective of where I placed my counter, would capture the centre spot if vacant.
    
2. One formation which the player should try to form is a triangle, in any size and in any rotation. It is also important to note that the triangle can have any side lengths as your opponent will be able to easily recognise simple triangles, so you should aim to form a triangle discreetly. The triangle allows two chances for you to win, either by making a square /rectangle or a parallelogram. Your opponent can only place one counter at a time, so your victory is determined as you always have the other option to win as well. In some cases, it is even possible for the player to form another parallelogram by placing their counter above the triangle if the space is vacant, but you may not always have this additional opportunity.
   
   
    
3. Besides this, we can also use the arrow formation which can also be used in the Square It game, as this will enable you victory when forming a square. The arrow formation can be used in any rotation or size. 
   
   
    
4. Another benefit of the arrowhead formation pictured above is that, besides forming a square or rectangle you can also form a parallelogram and this formation is one of the most efficient which should be used when tackling this game. From this image, the red circles indicate 4 possible places to win. Even if your opponent manages to recognise your arrowhead shape and prevent you from winning, you will still be able to form two parallelograms behind it. 
   
   

Clare thought about it a different way:

I noticed that, once you have three points on the board, there are always three different parallelograms you can make (assuming your opponent hasn't blocked those points). For example, in the diagram below, can you find the three ways that the blue triangle player could complete a parallelogram? What about the three ways the red square player could?

Click to see the possible points.

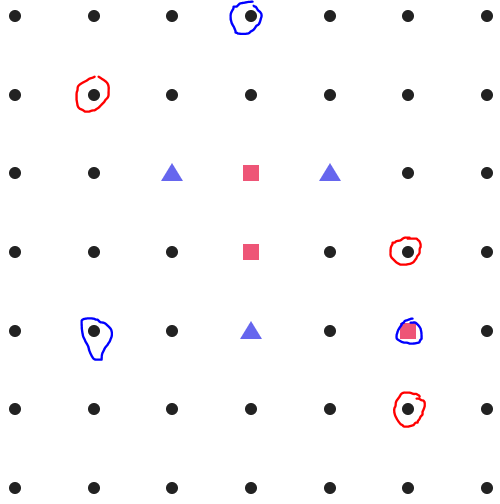
If you go first, then think about what happens when you place your third point. Three points can make three parallelograms, so if your opponent hadn't placed any points, you'd have three choices of parallelogram to make. But your opponent has already placed two points, so the worst way to choose third point would be if two of the possible parallelograms are already blocked by your opponent's points. As long as you don't make that choice for your third point, you can always win.