Skip over navigation
The Public Key
Age 16 to 18
Challenge Level 
This problem is intended to be tackled in conjunction with reading
the article on Public Key Cryptography though it is not essential
to understand the details of Public Key Cryptography in order to do
the problem. In itself it is a challenge to systematically reduce
the large number $180^{59}$ (using modulus arithmetic) to one which
the calculator can handle and finally to its equivalent modulo 391.
You may also like
Pythagorean Golden Means
Show that the arithmetic mean, geometric mean and harmonic mean of a and b can be the lengths of the sides of a right-angles triangle if and only if a = bx^3, where x is the Golden Ratio.
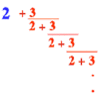
There's a Limit
Explore the continued fraction: 2+3/(2+3/(2+3/2+...)) What do you notice when successive terms are taken? What happens to the terms if the fraction goes on indefinitely?
