History of Measurement
Early man soon learned that joining forces with other people by living in communities was a way to keep safe. By pooling resources, such as their strength to do heavy jobs and their weapons, they could get more food, as well as wood for fires and for building, which they could share .
But to get
jobs done most effectively and to make sure that goods were shared
fairly, tools were needed. Some of the first mathematical tools man
developed where to help them measure. They had to measure the
passing of time, find ways to divide up pieces of land, develop a
system to help keep track of the number of animals they owned and
ways to measure grain so that they could exchange goods with each
other.
Over time,
foresters, builders, surveyors, map makers and scientists have
created special tools to help them measure the height of trees,
buildings and other tall structures very accurately. One of the
tools is called a theodolite, the illustration shows a surveyor
using one. You may have seen one on a building site. It is still
important to measure tall trees - which are in fact the largest and
oldest living organisms. Did you know that the tallest tree in the
world is in Redwood National Park in California? It's as tall as a
30 storey building! That's about 360 feet!!
Some of the early ways of measuring the height of tall structures are still in use today. They require very little equipment and can be used out in forests where special equipment is hard to come by. Why not try some of these methods for yourself? If you try more than one way you can then compare your results to discover if the answers you get are close - they should be. After all, the building or tree doesn't change its height because you change your measurement method! You might be surprised just how accurate you can be.
Native Americans had a very interesting and unusual way of seeing how high a tree was. They would bend over and look through their legs!
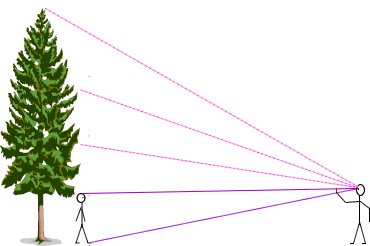
You need a partner who stands at the base of the tree. Move a distance away from the tree then, holding your pencil at arms length, between your thumb and forefinger so that it brackets the height of your partner (the top and bottom of the pencil coincide with the top of the head and the bottom of the feet of your partner). Now, use this length to step out the height of the tree in "pencil lengths". You can now measure the height of your partner and multiply this by the number of pencils high the tree is. This is worth doing several times from different distances and working out an average to improve the accuracy.
This is quite easy, once you have the knack. Describing the method makes it sound way more complicated than it is. After a couple of tries, you'll be able to apply for a lumberjack's job!
Fancy trying a third method? Don't forget to be comparing the sizes that you are getting for the tree/building height to see how they match up.
You will need a partner again, and another thing that you will need is a sunny day. You might have to pick your time for this experiment.
We'll call this method Measuring Shadows. Wait until the tree casts a shadow then measure the length of the shadow. Stand next to the tree and have a friend or parent measure your shadow. Be sure to do it as soon as you can after you measure the tree shadow! Measure your height to find out how tall you are. Now for the good part - get those calculators out! You will need the 3 measurements:
- the length of the tree's shadow
- the length of your shadow
- your height
Can you see why this works? Why not have a go?
You may also like
The Secret World of Codes and Code Breaking
When you think of spies and secret agents, you probably wouldn’t think of mathematics. Some of the most famous code breakers in history have been mathematicians.
History of Morse
This short article gives an outline of the origins of Morse code and its inventor and how the frequency of letters is reflected in the code they were given.