Skip over navigation
This solution was written by Andrei from Tudor Vianu National College, Bucharest, Romania.
I calculate the values of f(x)= x(x+|x|) for x< 0 and x\geq 0:
\eqalign{ f(x) &= x(x-x)=0\ for \ x < 0 \cr &=x(x+x)= 2x^2 \ for \ x\geq 0.}
Its graph is represented below: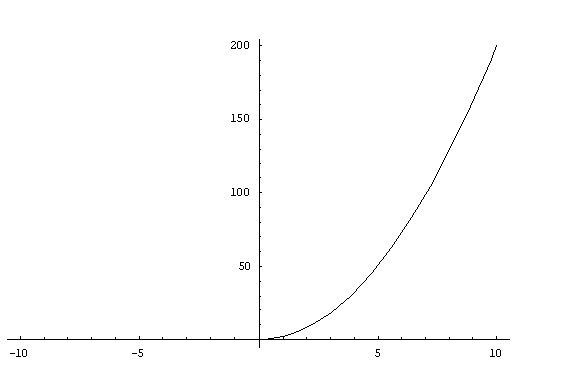

Or search by topic
Number and algebra
Geometry and measure
Probability and statistics
Working mathematically
Advanced mathematics
For younger learners
Slide
Age 16 to 18
Challenge Level 





- Problem
- Getting Started
- Student Solutions
- Teachers' Resources
This solution was written by Andrei from Tudor Vianu National College, Bucharest, Romania.
I calculate the values of f(x)= x(x+|x|) for x< 0 and x\geq 0:
\eqalign{ f(x) &= x(x-x)=0\ for \ x < 0 \cr &=x(x+x)= 2x^2 \ for \ x\geq 0.}
Its graph is represented below:
Now, I find the first derivative of f(x):
\eqalign{ f'(x) &= 0\ {\rm for}\ x < 0 \cr &= 4x
\ {\rm for}\ x\geq 0}
I observe that for x = 0, f'(x) is 0 both from the first
and from the second form of f(x), that is on both sides of the
origin. So the first derivative f'(0)=0 exists at x=0. Hence
the graph of f(x) has the tangent at y=0 at the origin.
Now, I calculate the second derivative:
\eqalign{ f''(x) &= 0\ for\ x < 0 \cr &= 4\ for \
x> 0.}
Hence the second derivative does not exist at the origin
because on the left the limiting value of f''(x) as x\to 0 is
0 whereas on the right the limiting value of f''(x) as x\to 0
is 4. So there isn't a unique tangent to the graph of f'(x) at
x = 0.
You may also like
Power Up
Show without recourse to any calculating aid that 7^{1/2} + 7^{1/3} + 7^{1/4} < 7 and 4^{1/2} + 4^{1/3} + 4^{1/4} > 4 . Sketch the graph of f(x) = x^{1/2} + x^{1/3} + x^{1/4} -x

