Chairs and Tables

Chairs and Tables

I made a chair from interlocking cubes. It looked a bit like this:

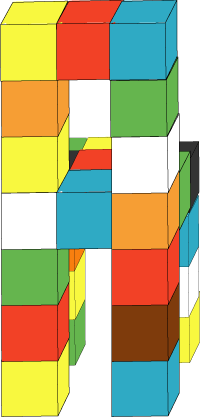
Can you make a chair too? It need not be the same as mine.
What are you thinking about as you make your chair?

Try making a table to go with your chair.
How are you going about making your table? What are you thinking about?

You could try making chairs for the Three Bears: a big chair, a middle-sized chair and a small one.

Please send us photos of your chairs and tables if you can, and we would love to know how you went about making them.

Why do this problem?

Although this problem sounds straightforward, some children may find the matter of scaling the table to match the chair quite a challenge. Tackling this will help to develop children's spatial awareness. It is also a great opportunity to encourage discussion among the class or group.

Possible approach

A hands-on approach is absolutely necessary for this problem, and you may like to read the story of Goldilocks and the Three Bears with the class to set the scene for this task.

You could start by making a chair from interlocking cubes (or Duplo/Lego) yourself (or show the picture in the problem) and then invite learners to share what they see, what they notice and any questions they have. Use the ensuing discussion to challenge them to make a chair of their own. You might like to put your chair somewhere it can be seen easily so children can refer to it as they build their own. Alternatively, you may decide to hide it at first to see how learners get on.

Children could then work in pairs or small groups to make a chair and a table under which the chair will fit. Before they have completed their models, stop them for a short time, and ask them what they are thinking about as they build. This is a fantastic opportunity to share some spatial reasoning - listen out for those children who have a sense of 'scale', however informal. Explicitly talking about what children are attending to will help all learners progress in the task.

At the end the whole group could come together to see each other's handiwork. If you have enough resources, the resulting creations would make a lovely table display, even if only for a short period of time!

Key questions

Possible support

Try to talk with those who appear to be struggling to find out what exactly it is that they are finding difficult. It might be that they would like help sequencing the construction e.g. legs, then seat, then back of the chair. Or it could be that it is the relative sizing that is tricky, in which case you may need to help them count cubes.

Possible extension

Encouraging children to articulate how they have made their models is key. What advice would they offer to children in another class who hadn't yet had a go at this task?