Skip over navigation
Congratulations to Ang Zhi Ping from River Valley High School, Singapore for this solution.
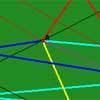
Take the vertex of the cube at the origin (0,0,0) as shown in the diagram. The tetrahedron has vertices O,X,Y,Z, where the three centres X, Y and Z are as follows: X=\left(1,\frac{1}{2},\frac{1}{2}\right), Y=\left(\frac{1}{2},1,\frac{1}{2}\right), Z=\left(\frac{1}{2},\frac{1}{2},1\right).
Or search by topic
Number and algebra
Geometry and measure
Probability and statistics
Working mathematically
Advanced mathematics
For younger learners
Four Points on a Cube
Age 16 to 18
Challenge Level 





- Problem
- Getting Started
- Student Solutions
- Teachers' Resources
Congratulations to Ang Zhi Ping from River Valley High School, Singapore for this solution.
Take the vertex of the cube at the origin (0,0,0) as shown in the diagram. The tetrahedron has vertices O,X,Y,Z, where the three centres X, Y and Z are as follows: X=\left(1,\frac{1}{2},\frac{1}{2}\right), Y=\left(\frac{1}{2},1,\frac{1}{2}\right), Z=\left(\frac{1}{2},\frac{1}{2},1\right).
The area required is the sum of the areas of the triangles XYZ (which is equilateral), and OXY, OYZ, OZX (which are congruent to each other and isosceles).
Now XY= 1/\sqrt{2} so that area(XYZ) = \frac{1}{2} \left(\frac{1}{\sqrt{2}}\right)^2 \sin 60^\circ = \frac{\sqrt{3}}{8}
Next, OX = OY = OZ = \sqrt{\frac{3}{2}}.
Thus OX = OY = \sqrt{\frac{3}{2}}, XY = \frac{1}{\sqrt{2}}.
This gives the area of OXY as \sqrt{11}/8. Thus the surface
area of the tetrahedron is \frac{\sqrt{3}}{8} +
\frac{3\sqrt{11}}{8}.
You may also like
Cushion Ball
The shortest path between any two points on a snooker table is the straight line between them but what if the ball must bounce off one wall, or 2 walls, or 3 walls?
Napoleon's Hat
Three equilateral triangles ABC, AYX and XZB are drawn with the point X a moveable point on AB. The points P, Q and R are the centres of the three triangles. What can you say about triangle PQR?

