Skip over navigation
We start by placing the two halves on the table so that the whole table is covered. Then we move them to overlap as in the question.
The area of the table covered by the paper has decreased by the two uncovered areas. The area covered by the paper has decreased by the amount covered twice. These two areas must both be the same, so the ratio must be 1:1
Alternatively:
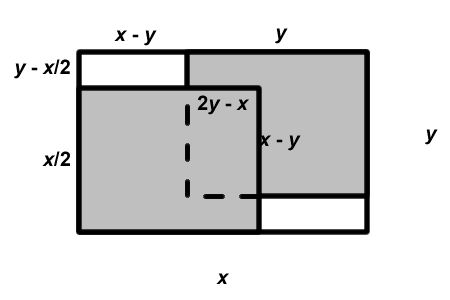
Let the sheet of paper have length x and width y. Then the uncovered area consists of two congruent rectangles of length x-y and width y-\frac{1}{2}x. So the uncovered area is 2(x-y)(y-\frac{1}{2}x), that is, (x-y)(2y-x).
The area covered twice is a rectangle of length y-(x-y), that is, 2y-x, and width \frac{1}{2}x-(y-\frac{1}{2}x), that is, (x-y). So the area covered twice is also (x-y)(2y-x).

Or search by topic
Number and algebra
Geometry and measure
Probability and statistics
Working mathematically
Advanced mathematics
For younger learners
Double Cover
Age 11 to 14
ShortChallenge Level 





- Problem
- Solutions
We start by placing the two halves on the table so that the whole table is covered. Then we move them to overlap as in the question.
The area of the table covered by the paper has decreased by the two uncovered areas. The area covered by the paper has decreased by the amount covered twice. These two areas must both be the same, so the ratio must be 1:1
Alternatively:

Let the sheet of paper have length x and width y. Then the uncovered area consists of two congruent rectangles of length x-y and width y-\frac{1}{2}x. So the uncovered area is 2(x-y)(y-\frac{1}{2}x), that is, (x-y)(2y-x).
The area covered twice is a rectangle of length y-(x-y), that is, 2y-x, and width \frac{1}{2}x-(y-\frac{1}{2}x), that is, (x-y). So the area covered twice is also (x-y)(2y-x).
This problem is taken from the UKMT Mathematical Challenges.
You can find more short problems, arranged by curriculum topic, in our short problems collection.
You may also like
Rule of Three
If it takes four men one day to build a wall, how long does it take 60,000 men to build a similar wall?
How Big?
If the sides of the triangle in the diagram are 3, 4 and 5, what is the area of the shaded square?

