Sums of Powers - A Festive Story

On the twelfth day of Christmas, my true love gave to me.. .

How many gifts?
But that's easy; all you have to do is add up the numbers from one to twelve.

That sounds easy, but what if the last line had been... fifty drummers drumming?
Isn't there a better way than huddling over your calculator?

And a partridge in a pear tree...

On the fifth day $1+2+3+4+5=15$ gifts are given. We can visualize this as $15$ squares arranged into the shape of a staircase; $1$ square on top of $2$ squares on top of $3$ squares etc.



 

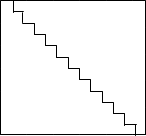
For the twelfth day we can repeat the process and end up with a new rectangle, as shown on the right. The rectangle is $12$ by $12+1=13$. We can now say: $$1+2+3+ ... +12 = \frac{12 \times (12+1)}{2} = 78$$

So the true love gets $78$ gifts on the twelfth day of Christmas.


How many gifts arrive on the $n$th day?

The same argument applies and we would end up drawing a rectangle that was $n$ squares high and $n+1$ squares wide. We would end up with: $$1+2+3+ ... +n = \frac{n \times (n+1)}{2}$$

That's all well and good but what if the true love went overboard on the whole gift front?

Instead of two turtle doves, he gave four;

instead of three French hens he gave nine ...

More precisely, if instead of giving $n$ gifts on the $n$th day, $n \times n$
(normally written as $n^2$) gifts are given, then what?
 

Now on the twelfth day there would be $1+4+9+25+\ldots + 144$ gifts.

Is it time to huddle over our calculator now?

Not quite yet, we can visualize the number of gifts on the third day, for example as $1$ cube on top of $4$ cubes on top of $9$ cubes arranged as in Figure A.


 

Now treat the object in A as a single building block. If you put two of these building blocks together you get the solid in Figure B.




 

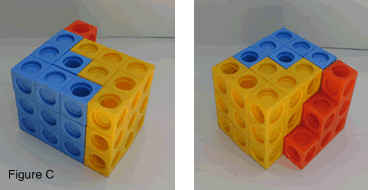
Adding another building block you get the solid on the left in Figure C. The picture on the right is just a different view of the solid shown on the left.






 

Figures D and E show two copies of the solid made from three building blocks separately (D) and then placed together (E).

Now what is the point of all this?

Well the task is to work out how many cubes are inside our building block, we can do it two ways.

By direct counting we get:

$1 + 4 + 9 = 14$.

But we also have shown that six of our building blocks can be arranged into the solid cuboid in Figure E. So, how many cubes are there in Figure E?

Well the cuboid is $3$ cubes high, $4$ cubes wide and $7$ cubes long and the cuboid contains $6$ of our building blocks.

So the volume of our building block is: $$1+4+9 = \frac{3 \times 4 \times 7}{6} = 14$$ Now here's a question:

Would the construction have worked if our building block had more layers, e.g. $1$ cube on top of $4$ cubes on top of $9$ cubes on top of $16$ cubes?

Twenty-seven French hens ...

A very pleasing result which means that ...

On the twelfth day our exhausted distributor of gifts would have dispensed:

For the rest of you intrepid explorers who want to carry on to the summit, for a whole number $n$ and $m$, go to the notes for more and more and more...