...on the Wall
...on the Wall printable sheet
This problem follows on from Mirror, Mirror...
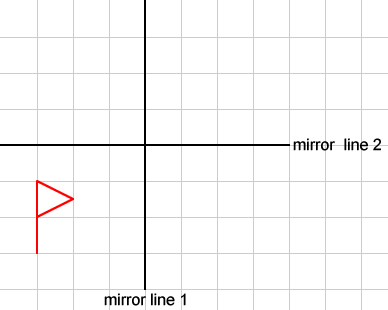
You might find it helpful to copy this diagram onto squared paper .
Reflect the flag in one of the lines.
Reflect the resulting image in the other line.
Can you describe the single transformation that takes the first flag to the last flag?
Does it matter in which line you reflect first?
Try this with the flag in other positions.
In each case, what is the single transformation that takes the first flag to the last flag?
Now try it with lines that meet at $45^{\circ}$ and at $60^{\circ}$ (you might find it helpful to use isometric paper for the $60^{\circ}$ case).
Again, try it with the flag in different positions
Can you describe the single transformation that takes the first flag to the last flag when the lines meet at $\theta^{\circ}$ (theta degrees)?
You may also like
Frieze Patterns in Cast Iron
A gallery of beautiful photos of cast ironwork friezes in Australia with a mathematical discussion of the classification of frieze patterns.
The Frieze Tree
Patterns that repeat in a line are strangely interesting. How many types are there and how do you tell one type from another?
Friezes
Some local pupils lost a geometric opportunity recently as they surveyed the cars in the car park. Did you know that car tyres, and the wheels that they on, are a rich source of geometry?