Magic Matrix

Several of you noticed that the numbers you end up with in the matrix always add up to 10 - to fit in with NRICH's 10th anniversary! A whole group of pupils from Gorseland Primary School worked hard on the first matrix in pairs, discovering how it works, making their own and solving this problem. They found this solution for the first matrix:

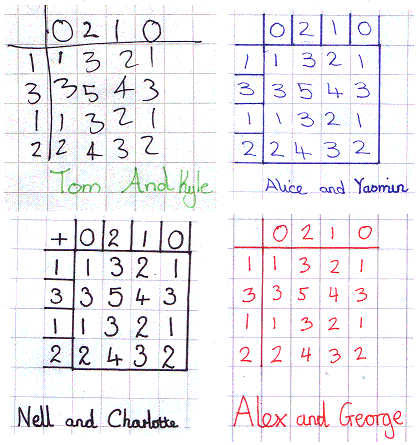
George from Greenwich solved the second and third matrices as well. For the second one, he says:

The numbers on the sides are the eight magic numbers, they are 1.7, 0.3, 0.1, 2.6, 0.2, 1.7, 1 and 2.4. The eight numbers add up to 10 and they fit in place:

	0.2	1.7	1	2.4
1.7	1.9	3.4	2.7	4.1
0.3	0.5	2	1.3	2.7
0.1	0.3	1.8	1.1	2.5
2.6	2.8	4.3	3.6	5

For the third matrix, George says:

What you have to do in the first step is convert all the fractions to a common denominator to make it easier. The common number is 12. This is the solved grid below. As you can see, the eight numbers are 8/12, 6/12, 19/12, 27/12, 5/12, 9/12, 30/12 and 16/12. They add up to 10 as well.

	6/12	19/12	27/12	5/12
8/12	14/12	27/12	35/12	13/12
9/12	15/12	28/12	36/12	14/12
30/12	36/12	49/12	57/12	35/12
16/12	22/12	35/12	43/12	21/12

You can check that this is the same as the matrix in the problem! Excellent George, thank you for sending in your solutions.