Article by Jennifer Piggott

Published 2011

Developing a Framework for Mathematical Enrichment

Abstract

"In mathematics the ability to solve problems is not just knowing some straightforward rules"

Polya (1957)

The NRICH Project (www.nrich.maths.org) has been in operation since 1996. Since this time the resources on the web site have grown and the project has developed a reputation for creative thinking in the area of mathematics enrichment.

The most recent work of the project has centred on making more effective use of the wealth of resources we now have available to us, both in terms of access to the enormous archive and in creating meaningful frameworks within which selections of the material can be placed (enrichment trails). As the new site and trails have developed we have questioned our understanding of mathematics enrichment and how it might be represented in classroom practice and through the NRICH site itself. The reflection and early research findings have resulted in two key outcomes that are having a fundamental impact on our work:

the resources have something to offer pupils of nearly all abilities. This has resulted in the structuring of the site and creation of the trails to facilitate a "free flow" of resources across age and ability boundaries.
enrichment is not only an issue of content but a teaching approach that offers opportunities for exploration, discovery and communication,
effective mediation offers a key with which to unlock the barriers to engagement and learning.

We are attempting to address the issues of the nature of enrichment, accessibility, mediation and the philosophies of learning and teaching that underpin our work both through the structure and content of the site, our work on enrichment trails and our face to face work with pupils and teachers.

This paper considers the key aspects of mathematics enrichment and how the content and design of trails (as well as the NRICH site itself) has been influenced by, and built upon, these philosophies.

Background and Rationale

The wider context

The United Kingdom Numeracy Framework offers guidance and exemplification of the mathematics curriculum giving content, structure and guidance on its implementation and delivery. However, although there has been an overall improvement in performance in national tests, there are areas where concerns still exist in terms of performance, teaching and attitudes to mathematics:

Concerns exist over pupil performance in algebra, geometry and problem solving (Brown, Millett et al. 2000). These concerns have most recently resulted in changes to the national mathematics attainment tests, which will now include a problem solving section.
Most commonly, the needs of most able pupils are met through courses of acceleration. Pupils undertaking such courses are often taught independently (and separately) from their peers, older pupils often having to go to other schools for their lessons. These models of acceleration pose medium to long term problems of sustainability and there is no evidence of long-term benefits. Ability grouping with "fast track" top sets has also been shown to cause problems in the long term (Boaler, Wiliam et al. 2000).
Fewer pupils are choosing to study mathematics and mathematics related subjects beyond the age of 16 (Nardi and Steward 2002), (Nardi and Steward 2002) (Nardi and Stewart 2003 forthcoming).
Evidence of lack of motivation and consequent dips in performance across KS3 is available and indicates pupils are being "turned off" mathematics. (Watson, 2001). Results in 2003 show a slight decline in performance over previous years resulting in the government adjusting long term performance targets.

Enrichment can be used:

to support the most able alongside all children in the class; often offering differentiation by outcome,
to promote mathematical reasoning and thinking skills, preparing pupils through breadth and experience to tackle higher level mathematics with confidence and a sense of pattern and place.

Mathematics Enrichment Materials on the NRICH Website

There have been a wealth of resources that support mathematical enrichment, most notably the NRICH online mathematics project (www.nrich.maths.org). The resources on the NRICH site have been in "loose leaf" format; being stored with few pointers to their curriculum context and relevance. This has left the user with issues of access to appropriate material and knowledge of the potential of, and means by which, the material can be used to support the development of high level mathematical reasoning (and other) skills.

From these points come the foci of our recent work:

identification of key aspects of an enrichment curriculum for mathematics that makes links between content, the national frameworks, and practice explicit;
effective presentation and structuring of resources on the NRICH site such that they will underpin an enrichment framework by offering exemplars of content and supporting material.

It is through examining the theories underpinning the development of structured content (trails) and views of teachers as users of the trails, the nature of mathematical enrichment and how it can be represented is being implemented.

Defining a Framework

Terms such as "mathematical thinking", "mathematical problem solving" and "enrichment" are variously described in current literature. Our work has therefore involved us in clarifying definitions of these terms. Establishing meanings has involved a literature review, interviews with colleagues and teachers and the analysis of NRICH team discussions. In addition, the process of site and trail development has involved multiple iterations which have themselves informed the definitions. These definitions are therefore constantly being reviewed and refined as we trial and test materials and build the framework within which our work is set. What is presented is our current view of these terms as they relate to our work.

Enrichment

In current literature, "enrichment" is almost exclusively used in the context of provision for the mathematically most able. However, there is strong evidence from the use of the NRICH site, and our own experience working with teachers and pupils, that this fails to address the value of an enrichment approach to teaching mathematics generally. Problems which offer suitable entry points can be used with pupils of a wide range of ability and therefore can be used within the "ordinary" classroom. The teacher or mentor can use such materials in flexible ways that respond to the needs (and experience) of the learner. We see enrichment as an approach to teaching and learning mathematics that is appropriate for all not simply the most able. NRICH resources therefore continue to support the most able but this is within the context of a broad interpretation and view of enrichment not within a context of provision simply targeting the most able. Good enrichment education is good education for all. Good mathematics education should incorporate an approach that is an enriching and stimulating experience for all pupils. 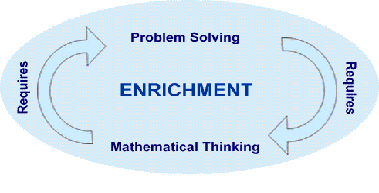The construction of enrichment we are adopting thus builds on two main threads:

Content

This thread describes an enrichment curriculum, which has the following components:

Content opportunities designed to:
- develop and use problem solving strategies,*
- encourage mathematical thinking,*
- include historical cultural contexts,
- offer opportunities for mathematical extension.

* These two strands form the focus of the content discussion in this paper

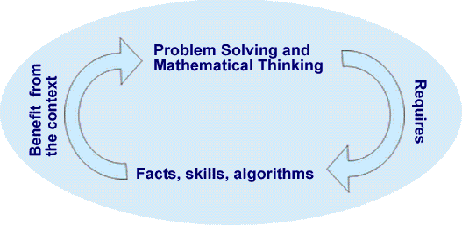
Enrichment is not simply learning facts and demonstrating skills. Mathematical skills and knowledge can be a precursors to, and also outcomes of, an enrichment curriculum (needs driven learning). The aim of an enrichment curriculum is to support:

a problem solving approach
improving pupil attitudes
a growing appreciation of mathematics
the development of conceptual structures

based on Ernest (2000)

Enrichment therefore represents an open and flexible approach to teaching mathematics which encourages experimentation and communication.

Teaching approach

This places an emphasis on teaching that reflects a constructivist view of learning and which stresses:

non-assertive mediation,
group work, discussion, communicating...,
varied solutions and different approaches being valued and utilised,
exploration, making mathematical connections, extending boundaries, celebrating ideas not simply answers, flexibility...,
acknowledgment that maths is hard but success is all the more enjoyable when a hurdle is overcome.

Problem Solving and Mathematical Thinking

A range of literature exists in the areas of Problem solving and Mathematical thinking, the two terms often being used synonymously or with a lack of clarity in their inter-relationship. As part of our framework for development we have been able to identify two distinct threads that appear in the use of the two terms and which are worthy of articulation and distinction. These threads pull together ideas drawn from current theory (Mayer (2002); Koshy (2001); Mason, Burton and Stacey (1985); Ernest (2000), Shoenfeld (1994), Polya (1957), Lester (1994), Cobb et al (1991), Van Zoest et al (1994), and our own work in the field.

We are taking "mathematical thinking" to mean particular mathematical strategies that are employed in solving problems of different types. Some exemplars of these strategies are given below. The aim is to identify problems where such strategies are useful and create a curriculum thread that encourages pupils to develop each strategy and identify the type of context and the ways is which such strategies can be employed.

Problem solving is reserved for the structural approach to solving problems - the overview, or steps on the journey from meeting a problem for the first time to its solution. Problem solving identifies and developments competence in utilising the stages on the route through solving a problem. Problem solving underpins the vast majority of NRICHs resources.

Thus mathematical thinking strategies are needed to tackle problems and will be used within the problem solving process.

Mathematical Thinking Strategies:

Some of the mathematical thinking strategies we have identified include:

Conjecturing/theorising;
Being systematic;*
Identifying common structures (isomorphisms);*
Introducing variables;
Generalising;*
Specialising/clarifying/looking for specific examples;
Considering a special case (the particular);
Solving simpler related problems;
Reflecting on experience - have you met something like this before?
Multiple representations;
Working backwards;
Identifying and describing patterns;
Representing information - diagram, table
Testing ideas - guessing and testing (hypothesizing).

*We have begun to develop curriculum resources that illustrate and support these aspects of mathematical thinking, in the form of trails.

There is still some work to do in identifying different aspects of mathematical thinking .Not all these strategies have a similar feel to them. Currently it seems easier to implement a developmental schema for some than for others.

Problem Solving Process

There are a number of descriptions of what constitutes problem solving within the literature (Mason et al (1985), Mayer (2002), Ernest (2000), Polya (1957)). These references have many common threads and have models of the process that are broken down into a varied number of stages. The process outlined below combines a number of the features of these existing models with our own research findings.

The C.A.P.E. model

Comprehension
- Making sense of the problem/retelling/creating a mental image,
- Applying a model to the problem;
Analysis and synthesis
- Identifying and accessing required pre-requisite knowledge,
- Applying facts and skills, including those listed in mathematical thinking (above),
- Conjecturing and hypothesising (what if);
Planning and execution
- Considering novel approaches and/or solutions
- Identifying possible mathematical knowledge and skills gaps that may need addressing,
- Planning the solution/mental or diagrammatic model,
- Execute;
Evaluation
- Reflection and review of the solution,
- Self assessment about ones own learning and mathematical tools employed,
- Communicating results.

Despite its representation, this is not a simple linear model -sometimes it is necessary to revisit and review several times -one can think of the problem solving process as a spiralling inward towards a satisfactory conclusion.

Implications for teaching for enrichment

I have discussed above the curriculum content associated with mathematical enrichment in terms of the two aspects of mathematical thinking and problem solving. For this content to have meaning, the learning (and teaching) environment needs to encourage effective use of the resources so that pupils develop the necessary skills, strategies and competence to tackle problems and use underpinning thinking skills effectively. This has implications for the second thread of mathematical enrichment '?? that of the teaching approach adopted. There are a number of features of such a teaching approach, building on the work of Lerman (1999), Romberg (1993) and Ruthven (1989) and takes a view of pupils constructing their own learning in a social context, where communication and sharing are central to mathematical growth and understanding. Aspects of such an approach include:

The use of problems which encourage a problem solving approach that in turn supports mathematical thinking and the contextualising of the relevance of mathematical skills and facts (known or to learn).
Employing the use of low threshold -high ceiling tasks
Giving pupils time to engage with the problem before moving towards a solution (exploration)
Focus on "doing mathematics" -pupils taking responsibility for tasks and identifying possible routes to and requirements of solutions rather than being led by the teacher.
Appropriately targeted mediation that supports entry into problems and development of solutions without leading. Building on pupil discovery and knowledge and making connections (codification)
Transfer of knowledge which is dependent upon individuals internalising schema with the teacher identifying opportunities.

Mathematical enrichment trails

The trails are a new concept of resource management that are being developed by the NRICH team, practising teachers and mathematics educators. They aim to combine related resources (problems, activities, games, articles, other sites) into a coherent programme of activities that have problem solving at their centre and which describe a strand of an enrichment curriculum aimed at either a particular aspect of mathematical thinking, or a particular aspect of the curriculum tackled through a problem solving approach. They also reflect the view of teaching and learning mathematics outlined above and are being described in terms of:

their mathematical content (standard curriculum facts and skills as well as mathematical thinking skills);
a recommended pathway, or pathways, through the items
prerequisite knowledge;
anticipated learning outcomes;
guidance notes for teachers which reflect the enrichment approach to teaching tha underpins our work
guidance notes and hints for pupils;
formative self-assessment mechanisms which will enable medium to long term planning and evaluation.

A trail, for example, might develop and support the work on number and problem solving through investigating Magic Squares. For the most able students the work might lead to investigating the idea of isomorphisms and the underlying structure of some mathematical problems (looking for pattern and familiarity in problem solving contexts - "have I seen something like this before"?). Brighter pupils may also be encouraged to consider algebraic properties and relationships in this context. A very able student may begin to generalise and look at "higher order" mathematics, looking at articles on the subject written by established mathematicians. Whilst students struggling with identifying patterns and relationships more generally may benefit from generalising their findings when working from one magic square context to another.

A trail on "being systematic" can offer opportunities in a range of mathematical contexts (number, geometry etc) to take a systematic approach to solving the problem. Whilst other proof, or algebra based methods may be just as appropriate in any particular context, the aim is to use a range of systematic strategies to access, engage in, and eventually solve, a problem. Work on the trail may extend over weeks or months or several academic years but in every case the aim is to give some structure to the development of the related skills.

The structure of a trail will enable choices concerning the routes into the resources to reflect the needs of the pupil and underlying learning theories. Trails aim to "unpick" the opportunities being offered to pupils to use and develop their problem solving and other higher order mathematical skills in terms of content, learning theories and associated teaching styles.

Implications for Implementation

Through the intertwining of the research and development of the NRICH site, and particularly the trails, the value of this curriculum innovation is being constantly assessed. All the work is grounded in appropriate theories as well as research and classroom experience that not only clarifies and informs the development itself but throws light on current views and practice with respect to the role, content and implementation of mathematics enrichment more generally. As materials are developed and tested this in turn informs our theoretical framework.

Mediation

An emerging area of interest is the nature and role of mediation and how mediation can take place, or underpinning learning theory be reflected, in the materials we produce. Current small-scale research by members of the NRICH team identifies the view of problems as rivers to be crossed rather than to be studied (the process is simply about finding the answer rather that mathematical discovery). This view acts as a barrier to encouraging problem solving and mathematical thinking skills. We are currently undertaking research into the role of mediation and how we can offer relevant mediation at a distance (Back, J., et al. 2004, forthcoming).

Conclusion

The clarification of the terms enrichment, mathematical thinking and problem solving have all led to a clearer understanding of the potential of NRICH to support mathematical enrichment more generally, being a vehicle for the many not simply the few.

Key outcomes:

establishing a view of enrichment/problem solving /mathematical thinking and reflecting this view within the resources we produce.
Placing the role of factual knowledge and skills within an enrichment framework both as a precursor and a consequence
the identification of mediation in a "remote" environment as a key area for our future research
continuing to reflect the importance of the social role in the construction of knowledge within an online and remote resource
that issues related to seeing the process and/or solution as the goal rather than the answer is key to our mediation and support work
that there is a role for assessment and that self and/or peer assessment is an area we need to investigate further.

Impact on the development of the NRICH site

The NRICH had the first phase of its relaunch in January 2004. The key features of the new site that have been driven by our research findings are:

Transparency between levels
Range of levels and difficulty (challenge level)
Monthly themes
Problems also include hints and notes
Integration of the thesaurus
Integration of the discussion boards
Easier access to related material within the archive.

Impact on the development of Trails

Clear rationale for each trail
Structure and accompanying documentation that supports learning theories and associated teaching approaches,
Picking particular mathematical thinking and problem solving schemes as focus for each trail
Developmental not ad-hoc organisation of resources
Consideration of the role of mediation and developing mediation strategies.
The choice of self-assessment as the core assessment strategy.

Bibliography

1. Back, J., Gilderdale, C., Piggott, J., 2004, forthcoming.

2. Boaler, J., Wiliam, D. et al., 2000, "Students' experiences of Ability Grouping - disaffection, polarisation and the construction of failure." British Educational Research Journal 26( 5): 631 - 648.

3. Brown, M., Millett, A. et al., 2000, "Turning our attention from the what to the how: the National Numeracy Strategy." British Educational Research Journal 26( 4): 457 -471.

4. Cobb, P., Wood, T. and Yackel, E. (1991). "A constructivist approach to second grade mathematics". In von Glaserfield, E. (Ed.), Radical Constructivism in Mathematics Education , pp. 157-176. Dordrecht, The Netherlands: Kluwer Academic Publishers.

5. Ernest, P., 2000, "Teaching and Learning Mathematics", in Koshy, V. et al, Mathematics for Primary Teachers . London Routledge.

6. Koshy, V.,2001, Teaching mathematics to able children , David Fulton.

7. Lerman, S., 1999, "Culturally Situated Knowledge and the Problem of Transfer in the Learning of Mathematics", in Learning Mathematics , Burton, L., (Ed), Studies in Mathematics Education Series, Falmer Press.

8. Lester, F.K.Jr., Masingila, J.O., Mau, S.T., Lambdin, D.V., dos Santon, V.M. and Raymond, A.M., 1994. "Learning how to teach via problem solving". in Aichele, D. and Coxford, A. (Eds.) Professional Development for Teachers of Mathematics , pp. 152-166. Reston, Virginia: NCTM.

9. Mason, J., Burton, L., Stacey, K.,1985, Thinking Mathematically , Prentice Hall

10. Mayer, R 2002, Mathematical Problem solving, Mathematical Cognition, 69-72

11. Nardi, E. and Steward, S., 2002, "Part 1: 'I could be the best mathematician in the world... if I actually enjoyed it'." Mathematics Teaching 179.

12. Nardi, E. and Steward, S., 2002, "Part 2: 'I'm 14, and I know that! Why can't some adults work it out?'." Mathematics Teaching 180.

13. Nardi, E. and S. Stewart (2003 forthcoming). "Is Mathematics T.I.R.E.D.? A profile of quiet disaffection in the secondary mathmatics classroom." British Educational Research Journal 28( 2).

14. Polya, G., 1957, How to Solve it , Princeton Paperbacks.

15. Romberg, T., A, 1994, "Classroom instruction that fosters mathematical thinking and problem solving: Connections between theory and practice." In A. H. Schoenfeld (Ed.), Mathematical thinking and problem solving (pp. 287-304). Hillsdale, NJ: Lawrence Erlbaum Associates.

16. Schoenfeld, A., 1994. "Reflections on doing and teaching mathematics." In A. Schoenfeld (Ed.). Mathematical Thinking and Problem Solving . (pp. 53-69). Hillsdale, NJ: Lawrence Erlbaum Associates.

17. Van Zoest, L., Jones, G. and Thornton, C. (1994). "Beliefs about mathematics teaching held by pre-service teachers involved in a first grade mentorship program". Mathematics Education Research Journal . 6 (1): 37-55.

18. Watson, A., 2001, Changes in mathematical performance of year 7 pupils who were 'boosted' for KS2 SATs. British Educational Research Association, Leeds, Education-

Number and algebra

Geometry and measure

Probability and statistics

Working mathematically

Advanced mathematics

For younger learners