Skip over navigation
What colour(s) could be on a marble that -
What colours would you find on a cold, sparkly, dark and rough marble?
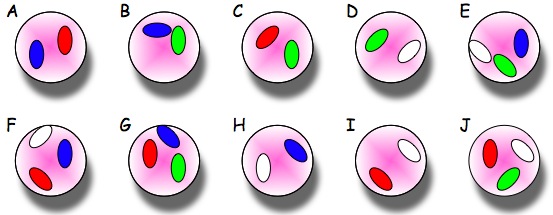
A Bag of Marbles
Age 5 to 7
Challenge Level 
A Bag of Marbles
Suppose that in a bag of marbles, all the ones that -
are rough to feel, have a blue colour on them;
sparkle when rolling, have a red colour on them;
are cold to touch, have a white colour on them;
are dark inside, have a green colour on them.
What can you say about each of the marbles A - J?
Imagine you are making marbles. What colours must be put on marbles that -
sparkle when rolling but are dark inside?
are cold to touch and feel rough?
are cold, but sparkle when rolling?
What colour(s) could be on a marble that -
is cold?
sparkles when rolling?
What colours would you find on a cold, sparkly, dark and rough marble?
Why do this problem?
This problem builds on simple sorting skills and gives children the experience of using logical reasoning.
Possible approach
It would be helpful if pupils had a sheet of marbles to cut out so that they could physically move or group them. (You might find it useful to print off this sheet which depicts four sets of the marbles.) In addition, some children might benefit from having coloured
pens to use so that they can record different marbles easily.
The first part of the problem, describing each marble, could be done as a whole group so that everyone become more familiar with the different attributes. You could then ask pairs of pupils to work on the second and third parts of the problem, emphasising that you will be interested in how they arrived at their solutions. You may like to draw attention to efficient ways of approaching the
problem that you see, or to clear ways of recording their solutions. It might be appropriate to stop them briefly after five minutes or so, once they have had a chance to look at the first question in the third part "What colour(s) could be on a marble that is cold?". The idea here is for the children to appreciate that there is not just one right answer - there are in fact several possibilities.
You could challenge the class to find all the possible marbles which fit the criteria, perhaps by having a section of a display board devoted to collecting examples over the week to come.
Key questions
How do you know that the marbles you've chosen are correct?
What other colours could be on a marble which is cold/sparkles when rolling?Possible extension
Children could be challenged to look at how marbles could be coloured if they have exactly two attributes, or three attributes or all attributes, and to record the number of possibilties each time. Do they have a system for making sure they don't leave out any possibilities each time?
Possible support
Having circles of paper cut out to represent marbles, and coloured pens to mark each with, may help children access this problem.
You may also like
Strike it Out
Use your addition and subtraction skills, combined with some strategic thinking, to beat your partner at this game.
Two Numbers Under the Microscope
This investigates one particular property of number by looking closely at an example of adding two odd numbers together.
Odd Times Even
This problem looks at how one example of your choice can show something about the general structure of multiplication.