Skip over navigation
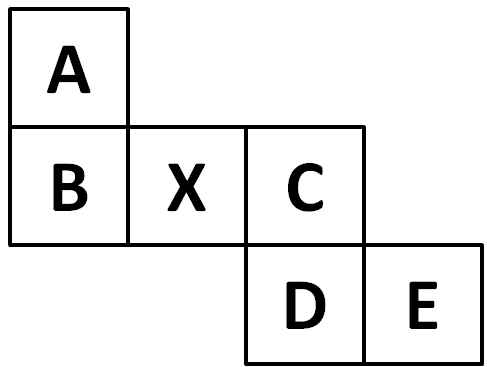
The net shown here is cut out and folded to form a cube.
Which face will be opposite the face marked $X$?
Net Result
Age 11 to 14
ShortChallenge Level 
The net shown here is cut out and folded to form a cube.
Which face will be opposite the face marked $X$?
If you liked this problem, here is an NRICH task which challenges you to use similar mathematical ideas.
This problem is taken from the UKMT Mathematical Challenges.
You can find more short problems, arranged by curriculum topic, in our short problems collection.
You may also like
Rhombicubocts
Each of these solids is made up with 3 squares and a triangle around each vertex. Each has a total of 18 square faces and 8 faces that are equilateral triangles. How many faces, edges and vertices does each solid have?
Cutting a Cube
A half-cube is cut into two pieces by a plane through the long diagonal and at right angles to it. Can you draw a net of these pieces? Are they identical?
