Skip over navigation
Two of our best solvers, Alex from Stoke on Trent Sixth Form College and Patrick from Woodbridge School thought about this implicit equation.
r^2X^2-rX-r+1=0
Patrick looked at the first parts using the quadratic equation formula as follows:
Part 1:
If r=1 then X^2-X = 0, so X(X-1) = 0 and X = 0 or 1.
If r = 100 then 100^2X^2 - 100X - 100 + 1 = 0 so 100^2X^2-100X-99 = 0 so
X = \frac{100\pm \sqrt{100 ²+4\times 100 ²\times 99}}{ 2\times 100^2}
This is real as we neither divide by 0 nor take a negative square root.
Part 2:
If r = 0 then we find the equation gives 1=0, so r cannot equal 0.
Substituting r^2 for a, -r for b and -(r-1) for c in the usual quadratic equation formula we get
X = \frac{r\pm\sqrt{r ²+4r ²(r-1)}}{2r^2}=\frac{1\pm\sqrt{1+4r-4}}{2r} = \frac{1\pm\sqrt{4r-3}}{2r}
So this takes real values if and only if 4r - 3 \geq 0.
Alex realised that the key feature of this problem was the discriminant of the quadratic equations in X, and looked at that object directly:
The discriminant determines whether a quadratic has real roots, therefore X(r) will have real values if and only if the discriminant D of the quadratic is zero or positive.
\begin{eqnarray} D&\geq 0&\cr \Rightarrow (-r)^2 - 4(r^2)(1-r) &\geq& 0 \cr \Rightarrow r^2 - 4r^2 + 4r^3 &\geq& 0\cr \Rightarrow 4r^3 &\geq& 3r^2\cr \Rightarrow r &\geq& 3/4 \end{eqnarray}
So, X(r) has real values for r\geq 3/4.
This means that X(1) and X(100) have real values, but X(-1) does not. X(0) is undefined because the corresponding equation (1=0) has no solutions, it is a contradiction.
Alex also correctly deduced the maximum and minimum values of X(r)
The maximum value of X(r) is X(1) = 1. The minimum value of X(r) is X(3) = -1/3. The graph has an asymptote r = 0.
Alex submitted a plot of the associated curve, which shows two distinct branches.

Steve solved this problem in the following way:
X = \frac{-b \pm \sqrt{b^2 - 4ac}} {2a}
X = \frac {1 \pm \sqrt{4r - 3}}{2r}
X is therefore real for all r greater than or equal to \frac{3}{4}
Hence r = 0 and -1 give complex roots of X but 1 and 100 give real roots.
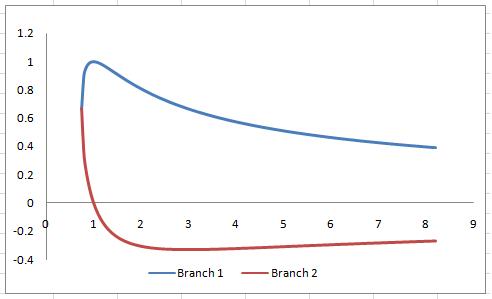
Asymptote at r = 0
As r \to \infty, X \to 0
\frac{\mathrm{d}X}{\mathrm{d}r} = \frac{\mathrm{d}}{\mathrm{d}r} \left[ \frac{1}{2r} \left(1 \pm \sqrt{4r - 3} \right) \right] = \frac{-2 \left( \sqrt{4r-3} \right) +4r-6} {r^2 \sqrt{4r-3}}
Setting \displaystyle \frac{\mathrm{d}X}{\mathrm{d}r} = 0 we find r = 1,\ 3
Or search by topic
Number and algebra
Geometry and measure
Probability and statistics
Working mathematically
Advanced mathematics
For younger learners
Implicitly
Age 16 to 18
Challenge Level 





- Problem
- Getting Started
- Student Solutions
- Teachers' Resources
Two of our best solvers, Alex from Stoke on Trent Sixth Form College and Patrick from Woodbridge School thought about this implicit equation.
r^2X^2-rX-r+1=0
Patrick looked at the first parts using the quadratic equation formula as follows:
Part 1:
If r=1 then X^2-X = 0, so X(X-1) = 0 and X = 0 or 1.
If r = 100 then 100^2X^2 - 100X - 100 + 1 = 0 so 100^2X^2-100X-99 = 0 so
X = \frac{100\pm \sqrt{100 ²+4\times 100 ²\times 99}}{ 2\times 100^2}
This is real as we neither divide by 0 nor take a negative square root.
Part 2:
If r = 0 then we find the equation gives 1=0, so r cannot equal 0.
Substituting r^2 for a, -r for b and -(r-1) for c in the usual quadratic equation formula we get
X = \frac{r\pm\sqrt{r ²+4r ²(r-1)}}{2r^2}=\frac{1\pm\sqrt{1+4r-4}}{2r} = \frac{1\pm\sqrt{4r-3}}{2r}
So this takes real values if and only if 4r - 3 \geq 0.
Alex realised that the key feature of this problem was the discriminant of the quadratic equations in X, and looked at that object directly:
The discriminant determines whether a quadratic has real roots, therefore X(r) will have real values if and only if the discriminant D of the quadratic is zero or positive.
\begin{eqnarray} D&\geq 0&\cr \Rightarrow (-r)^2 - 4(r^2)(1-r) &\geq& 0 \cr \Rightarrow r^2 - 4r^2 + 4r^3 &\geq& 0\cr \Rightarrow 4r^3 &\geq& 3r^2\cr \Rightarrow r &\geq& 3/4 \end{eqnarray}
So, X(r) has real values for r\geq 3/4.
This means that X(1) and X(100) have real values, but X(-1) does not. X(0) is undefined because the corresponding equation (1=0) has no solutions, it is a contradiction.
Alex also correctly deduced the maximum and minimum values of X(r)
The maximum value of X(r) is X(1) = 1. The minimum value of X(r) is X(3) = -1/3. The graph has an asymptote r = 0.
Alex submitted a plot of the associated curve, which shows two distinct branches.

Steve solved this problem in the following way:
Part 1:
X = \frac{-b \pm \sqrt{b^2 - 4ac}} {2a}
X = \frac {1 \pm \sqrt{4r - 3}}{2r}
X is therefore real for all r greater than or equal to \frac{3}{4}
Hence r = 0 and -1 give complex roots of X but 1 and 100 give real roots.
Part 2:

Asymptote at r = 0
As r \to \infty, X \to 0
Part 3:
\frac{\mathrm{d}X}{\mathrm{d}r} = \frac{\mathrm{d}}{\mathrm{d}r} \left[ \frac{1}{2r} \left(1 \pm \sqrt{4r - 3} \right) \right] = \frac{-2 \left( \sqrt{4r-3} \right) +4r-6} {r^2 \sqrt{4r-3}}
Setting \displaystyle \frac{\mathrm{d}X}{\mathrm{d}r} = 0 we find r = 1,\ 3

