Skip over navigation
We had some good solutions sent in for this group activity, first from Oliver and Daniel from S.U.N. School:
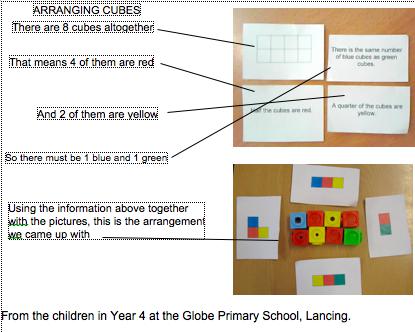
First, we read the statements out and found out that half of the cubes were red, a quarter were yellow and there is the same number of blue cubes as green cubes.
Secondly, Oliver said that, on the picture, there are four cubes across and two down.
Then, Daniel said that one of the lines along was: green, red, yellow.
After that, we discovered that inside the green, red, yellow sequence was the green, red combination so we didn't need to do that card.
Then Daniel found on one of his cards was a corner piece with a red block and a yellow and blue block either side so we added that in.
Then we found there was one piece left, the yellow, red, blue combination and all of the
things were somewhere in our rectangle.
This is what it looked like in a drawing.
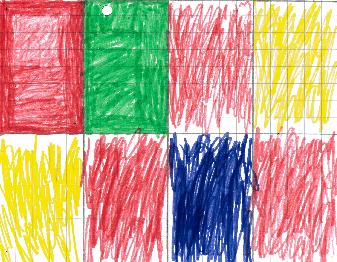
Then a well-presented solution came in from pupils at The Globe School in Lancing. Here it is!
Then from Mia, Jamie-Lee, Shaneece and Spencer from Elphinstone School we had this good explanation. Thank you so much for the written details:
We went around the circle and listened to each other's explanations.
First we found out the shape and that there were eight squares (this was the most important card).
Secondly, we found that half of the squares were red, so we knew there had to be four red squares.
Then we found out that there were the same amount of blues as greens. At first we thought there were two blues and two greens, but when we found out there were a quarter yellows (this had to be two yellows because that is a quarter of eight), we realised there had to be one green and one blue.
Then we found we needed an L shape yellow, red and blue, then a line of yellow, red and blue.
Finally we added the red and blue. Finished. It looks like this:
YRBR RBRY
RYRG or GRYR
Two groups with exciting names from St. James School sent in these pictures of their work. First we have the Flying Dolphins:

Then we have the Golden Terrifying Tigers who sent in this picture:

Thank you for all these solutions. Maybe others will be encouraged to try the same activity.

Or search by topic
Number and algebra
Geometry and measure
Probability and statistics
Working mathematically
Advanced mathematics
For younger learners
Arranging Cubes
Age 7 to 11
Challenge Level 





- Problem
- Student Solutions
- Teachers' Resources
We had some good solutions sent in for this group activity, first from Oliver and Daniel from S.U.N. School:
First, we read the statements out and found out that half of the cubes were red, a quarter were yellow and there is the same number of blue cubes as green cubes.
Secondly, Oliver said that, on the picture, there are four cubes across and two down.
Then, Daniel said that one of the lines along was: green, red, yellow.
After that, we discovered that inside the green, red, yellow sequence was the green, red combination so we didn't need to do that card.
Then Daniel found on one of his cards was a corner piece with a red block and a yellow and blue block either side so we added that in.
Then we found there was one piece left, the yellow, red, blue combination and all of the
things were somewhere in our rectangle.
This is what it looked like in a drawing.

Then a well-presented solution came in from pupils at The Globe School in Lancing. Here it is!

Then from Mia, Jamie-Lee, Shaneece and Spencer from Elphinstone School we had this good explanation. Thank you so much for the written details:
We went around the circle and listened to each other's explanations.
First we found out the shape and that there were eight squares (this was the most important card).
Secondly, we found that half of the squares were red, so we knew there had to be four red squares.
Then we found out that there were the same amount of blues as greens. At first we thought there were two blues and two greens, but when we found out there were a quarter yellows (this had to be two yellows because that is a quarter of eight), we realised there had to be one green and one blue.
Then we found we needed an L shape yellow, red and blue, then a line of yellow, red and blue.
Finally we added the red and blue. Finished. It looks like this:
YRBR RBRY
RYRG or GRYR
Two groups with exciting names from St. James School sent in these pictures of their work. First we have the Flying Dolphins:

Then we have the Golden Terrifying Tigers who sent in this picture:

Thank you for all these solutions. Maybe others will be encouraged to try the same activity.
You may also like
Poly Plug Rectangles
The computer has made a rectangle and will tell you the number of spots it uses in total. Can you find out where the rectangle is?

