Charlie's Delightful Machine

Level 1
Jenna from Wootton Upper School in the UK made some observations about how often each light lit up:
The green light lights up after every 3 numbers. The red light lights up after every 4 numbers. The yellow light shows after every 5 numbers and the blue light lights up after every 6 numbers.

Arjun and Eddy from Levendale Primary School noticed something very similar:
Adding or subtracting 3 starting from 0 is green.
Adding or subtracting 7 starting from 6 is yellow.
Adding or subtracting 8 starting from 4 is red and yellow.

Sadaf, Anhaar and Vaneeza and Jonathan and Jayme from Greenacre Public School in Australia listed numbers that lit up the lights to help them find patterns. Sadaf, Anhaar and Vaneeza used a method like in Shifting Times Tables:
We found that the best way to find the pattern is to first look at Shifting Times Tables to understand the shifting pattern.

We recorded when each colour would light up, under each colour name we listed the numbers that caused it to light up. We found the pattern between the numbers and found how much it shifted, we then predicted the next number and checked whether this number would cause a light to light up.

An example of this solution:
Red: 5, 16, 27, 38
Therefore the pattern is increasing by 11 and it shifts down by 6 [because the numbers are 6 less than the numbers in the 11 times table].

Leo from Wootton Upper School and Rishika from Nonsuch High School for Girls in the UK found an expression for the term in the $n^\text{th}$ position. This is Rishika's work:
Firstly, I constructed a table, similar to the one shown below, to help mark and track which numbers satisfy the rule of the different lights.

	0	1	2	3	4	5	6	7	8	9	10	11	12	13	14	15	16	17	18	19	20
Yellow			X									X									X
Red	X			X			X			X			X			X			X
Green			X			X			X			X			X			X			X
Blue		X											X

Then, I wrote out the sequences for each light.
For example, Yellow: $2,11,20,29”¦$
We can notice that the sequence starts with $2$ and $9$ is added each time to find the next number. To generate a mathematical rule for this, I found the $n^\text{th}$ term of the sequence. This enables us to acquire the terms in the sequence without using the machine.

The common difference (number the sequence goes up in) of the sequence is $9$, and this can be found by subtracting any one term by the previous term (e.g. $11-2$ or $20-11$). 
I used this to find the '$0^\text{th}$ term' (the term preceding the $1^\text{st}$ term) by subtracting the $9$ from the first term, giving $-7$.

Therefore, the $n^\text{th}$ term of the sequence is $9n-7$. This tells us that the sequence is arithmetic and $9$ is added each time to find the next term.

We can then substitute the term positions to determine other numbers in the sequence.
$9(1)-7 = 2$
$9(2)-7 = 11$
$9(3)-7 = 20$
$9(4)-7=29$
$9(5)-7 = 38”¦$
These are the numbers for which the lightbulb would switch on.
We can also predict larger numbers for which the bulb would light - for example, the $100^\text{th}$ number would be:
$9(100)-7 = 893$

Four digit numbers in the sequence would include:
$9(112)-7=1001$
$1001,1110,1119”¦.$
The other sequences generated the rules/$n^\text{th}$ terms:
Red: $0,3,6,9”¦ \rightarrow 3n-3$
Blue: $1,12,23,34”¦ \rightarrow 11n-10$
Green: $2,5,8,11”¦ \rightarrow 3n-1$

These are all arithmetic sequences - there is a common difference that is added or subtracted (shown by the coefficient of $n$).


Pavi, Polina and Ayana from International School of Lausanne in Switzerland also generated rules and used these to help them find larger numbers that would turn on the lights. They recorded their results in a table:
We investigated a Level 1 challenge. And shown below are the rules we found in a table. At first, we listed the numbers where the light bulbs would turn on. Then, we tried to find a pattern, which we did, and recorded the rule in a table. After all the rules were found, we selected a random 4 digit number, and modified it to suit the rules.

Yellow	Red	Blue	Green
Rule: multiples of $4$	Rule: $(7\times(n-1))+2$	Rule: $(5\times(n-1))+3$	Rule: $(11\times(n-1))+8$
$4000$	$6995$	$4998$	$1097$

Levels 2 and 3
Sadaf, Anhaar and Vaneeza noticed that the patterns were different for some level 2 lights:
A new rule after refreshing and clicking on the level button:
2, 4, 8, 14
The pattern (difference/distance) between the numbers is:
2, 4, 6
And so on, it does not shift. Therefore the pattern (of the differences between the numbers) starts off at 2 and increases by 2 for every number.

Arjun and Eddy found the following patterns:
Yellow = all square numbers
Blue = start on 5 and add 8 and increase what you add every time by 2.
Red = start on 0 then add 2 then 4 then 6 etc.
Green = start on 7 then add 10 every time.

Leo and Rishika found an expression for the term in the $n^\text{th}$ position. This is Rishika's work:
For levels 2 and 3, I followed a similar method. However, I noticed that for some sequences, there was not a first common difference. 

For example, Level 2 Red generated: $3,4,7,12”¦$
The difference between the terms differed each time - but by the same amount:
2nd term $-$ 1st term $4-3=1$
3rd term $-$ 2nd term $7-4=3$
4th term $-$ 3rd term $12-7=5$

The common difference of these was $2$ ($5-3$ or $3-1$) - this is called the second common difference.

This is typical of a quadratic sequence, where the second common difference is the same. 
I then found the $n^\text{th}$ term of the quadratic sequence, which is expressed in the form $an^2+bn+c$.

This is Rishika's diagram showing how the second difference is constant for sequences of this type (where the term in the $n^\text{th}$ position is equal to $an^2+bn+c$).

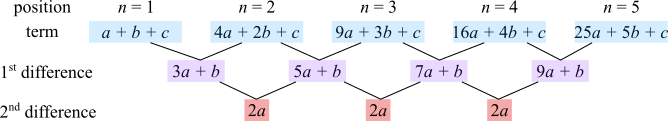
To find $a$, I used the formula $2a=$ second common difference
$2a=2$ (since the second common difference for this sequence was $2$) 
$\Rightarrow a=1$, which I substituted into the equation $3a+b=1$ (the first of the first differences was $1$)
$3(1)+b=1 \Rightarrow b=-2$
I then substituted both values into the formula $a+b+c=$ the first term (which was $3$)
$(1)+(-2)+c=3 \Rightarrow c=4$

Therefore, the $n^\text{th}$ term in this quadratic sequence is $n^2-2n+4$ (expressed in the form $an^2+bn+c$). 
We can use the same method to generate any terms in the sequence by substituting positions of numbers in the sequence. For example, the $33^\text{rd}$ term would be:
$(33)^2-2(33)+4=1027$

Other Level 2 and 3 rules I generated included:
Level 2:
Yellow: $1,4,7,10”¦$ (is arithmetic as the first difference is the same) $3n-2$
Green: $9,10,13,18,25”¦$ (quadratic as the second difference is the same) $ n^2+2n+10$
Blue: $7,9,13,19,27”¦:  n^2-n+7$

Level 3:
Red: $5,17,29,41”¦ $ (arithmetic, common difference$=12$) $12n-7$
Yellow: $10,11,14,19”¦ : n^2-2n+11$
Blue: $2,11,20,29”¦ : 9n-7$
Green: $6,7,9,12,16 : 0.5n^2-0.5n+6 $