Or search by topic
Number and algebra
Geometry and measure
Probability and statistics
Working mathematically
Advanced mathematics
For younger learners
Build it Up



- Problem
- Student Solutions
- Teachers' Resources
We had a very large number of solutions come in for this challenge. Here are a selection for you to consider.
Primary 6 at Victoria Primary School in Scotland sent in this very thorough solution
Firstly, we looked at the 4 numbers at the bottom and tried to work out how to get to 15. As a class we agreed that this would take too long for us to try and work out all the 4 digit numbers. So next we worked out a system...
We started at the top and worked out all the 2 digit numbers that make 15 which were
14 + 1; 13 + 2; 12 + 3; 11 + 4; 10 + 5; 9 + 6; 8 + 7; 7 + 8; 6 + 9; 5 + 10; 4 + 11; 3 + 12; 1+ 14;
Then we looked at next row down and worked out that we couldn't have 1 + 14 and 14 + 1 because we we couldn't use 0 so nothing adds up to 0. We took a pair of numbers each and worked out some of the group of 3 numbers which were:
| making 15 | making | the | two | totals | |||
| 9 + 6 | 8,1,5 | 7,2,3 | 6,3,2 | 5,4,3 | 4,5,1 | ||
| 5 + 10 | 4,1,9 | 3,2,8 | 2,3,7 | 1,4,6 | |||
| 12 + 3 | 11,1,2 | 10,2,1 | |||||
| 6 + 9 | 3,3,6 | 2,4,5 | 1,5,4 | 4,2,7 | |||
| 11 + 4 | 10,1,3 | 9,2,2, | 8,3,1 | ||||
| 8 + 7 | 7,1,6 | 6,2,5 | 5,3,4 | 4,4,3 | 3,5,2 | 2,6,1 | |
| 7 + 8 | 3,4,5 | 5,2,6 | 6,1,7 | ||||
| 10 + 5 | 9,1,4 | 8,2,3 | 7,3,2 | 6,4,1 | |||
| 3 + 12 | 1,2,10 | 2,1,11 | |||||
| 13 + 2 | 12,1,1 | ||||||
| 4 + 11 | 3,1,10 | 2,2,9 | 1,3,8 |
And from that we were able to do the exact same to work out the four
numbers which are:
2221; 8111; 4122; 4211; 5212; 3211; 5211; 4122; 7112; 5114; 3213; 3123; 7112; 2131; 7112; 7223; 5211; 2214; 2311; 8231; 1312; 6113; 1127; 1118; 4212;
We really enjoyed the challenge and our (something? brains? hands? minds ?) hurts after it but in a good way.
We really needed to keep a growth mindset.
This has been explained very well but now have a look at the ones I've put in italics (and underlined) what do you think about them?
We also had a number of solutions sent in from Peak School in Hong Kong
Alyssa wrote:
I found that all of my solutions always had a 1 in the bottom row, and the
total of all the numbers in the bottom row was always an odd number.
Victoria K & F wrote:
These are our solutions:
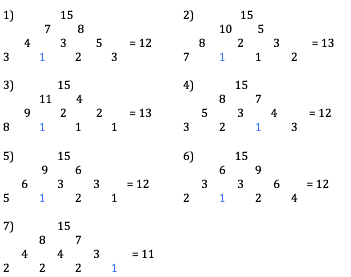
Rules:
There has to always be a 1 in the bottom row.
In the bottom row will always be a number repeated twice.
The bottom row must equal an odd number.
The second to bottom row must equal 11, 12 or 13.
There must be a combination of odd and even numbers in every row.
Mathias wrote:
I found out 9 different ways to get 15 using different numbers.

Sophie and Dorika wrote:
We think that the bottom line has to add up to an odd number.
We also found out that there has to be a one in in the bottom row.
The middle line can't have a one in it.
The second to bottom row will equal to 11, 12, 13.
On the bottom row a number will always be repeated twice or three times.
There are only 4 pairs of numbers that can be used in the second row.

Extension:
We combined your idea and we did our own version using decimal points
15
9 6
6 3 3
4 2 1 2
2.5 1.5 .5 .5 1.5
1.25 1.25 .25 .25 .25 1.25
.125 1.125 .125 .125 .125 .125 1.125
Chloé, Holly and Anna wrote:
We found these solutions by starting from the top row and working our way
to the bottom:

We found some rules after finding the solutions:
-The bottom row must equal an odd number (7, 9, 11)
-The second to bottom row equal 11,12 or 13
-On the bottom row there will always be a number repeated twice
-There has to be be one in the bottom row
Thanks for reading!
Jihun from Dong Sung Elementary School in South Korea sent in the workthat had been done:
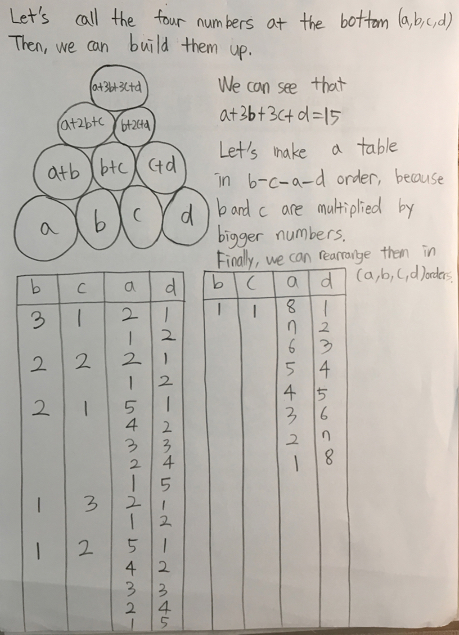
Well done all of you. It was very interesting reading about your methods and the things that you found out that had to be. Keep up your good work.
Related Collections
You may also like
Consecutive Numbers
An investigation involving adding and subtracting sets of consecutive numbers. Lots to find out, lots to explore.
Roll These Dice
Roll two red dice and a green dice. Add the two numbers on the red dice and take away the number on the green. What are all the different possible answers?

