Beach Huts

Beach Huts printable worksheet

A "beach hut" is generated from its two middle numbers.
Can you figure out the rule for working out the roof and floor numbers?


 

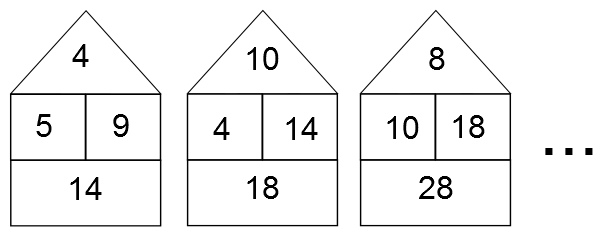
These beach huts form a sequence. Can you see how the second hut follows on from the first? And how the third hut follows on from the second?

Can you find the next huts in the sequence?
Do you notice any interesting patterns?

Try making some sequences of huts starting with your own numbers in the middle two boxes.
Do these sequences follow similar patterns?


If you have a beach hut from the middle of a sequence, is there a way to work out the beach huts that came before it?
Here's one to try:

 

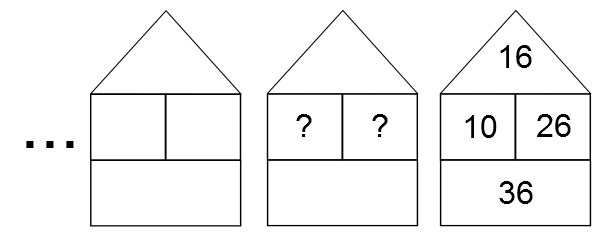
Here are some questions you might like to consider:

If you know any pair of numbers from a beach hut, can you work out the other two numbers?

Can you find a sequence of beach huts where two adjacent huts have the same roof number?

If you know the numbers in a hut, how can you work out the numbers of the fourth hut to the right of it? Or the 10th? Or the 100th...?

If you know the numbers in a hut, how can you work out the numbers of the sixth hut to the left of it? Or the 12th? Or the 100th...?

If you know the numbers in a hut, how can you work out the numbers of the seventh hut to the right of it? Or the fifth hut to the left of it? Or the 99th in either direction...?





With thanks to Don Steward, whose ideas formed the basis of this problem.