Skip over navigation
Well done to Vid from Osnovna Å¡ola Loka ÄŒrnomelj in Slovenia, who sent us the following solution to the problem:
Angle c = 45^\circ because it is the angle between the diagonal and the side of a square. To prove that a+b=c, I shall begin by adding points to the diagram to help with naming angles:
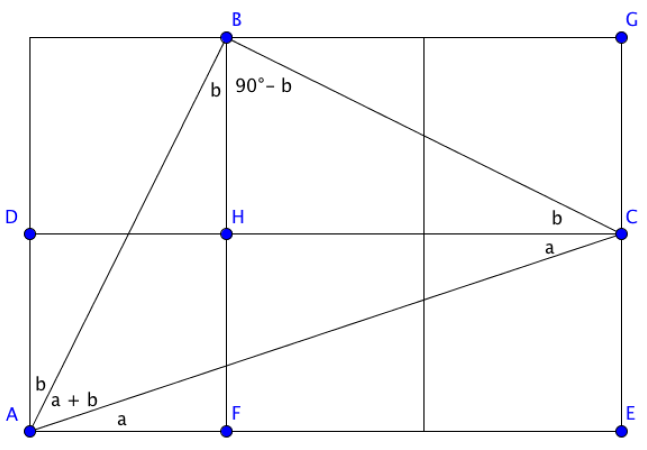
Now we can find several angles:
Therefore, adding the angles of \triangle ABC together, we have: \begin{align}\angle ABC + \angle BAC + \angle ACB &= 180^\circ \\ ((90^\circ - b) + b) + (a + b) + (a + b) &= 180^\circ \\ 90^\circ + (a + b) + (a + b) &= 180^\circ\\ 90^\circ + 2(a + b) &= 180^\circ\\ 2(a + b) &= 180^\circ - 90^\circ\\ 2(a + b) &= 90^\circ\\ a + b &= \frac{90^\circ}{2}\\ a + b &= 45^\circ\end{align}
Therefore, since c = 45^\circ, we have that a + b = c.

Or search by topic
Number and algebra
Geometry and measure
Probability and statistics
Working mathematically
Advanced mathematics
For younger learners
Angles in Three Squares
Age 14 to 16
Challenge Level 





- Problem
- Getting Started
- Student Solutions
- Teachers' Resources
Well done to Vid from Osnovna Å¡ola Loka ÄŒrnomelj in Slovenia, who sent us the following solution to the problem:
Angle c = 45^\circ because it is the angle between the diagonal and the side of a square. To prove that a+b=c, I shall begin by adding points to the diagram to help with naming angles:

Now we can find several angles:
- Firstly, \angle CAE = a and \angle BAD = b.
- Since DC is parallel to AE, by alternate angles, we know that \angle DCA = \angle CAE.
- Next, we can see that \angle BCD = b.
- Similarly, \angle ABF = \angle CBG = b.
- Since \angle BHC is a right angle and the sum of angles in a triangle is 180^\circ, then we have that \angle CBH = 180^\circ - 90^\circ - b = 90^\circ - b.
- Hence, we have that \angle BCA = a + b.
- In \triangle ABC, the line segments AB and BC have equal length, so we have that \triangle ABC is isosceles. Therefore, \angle BAC = \angle BCA = a+b.
Therefore, adding the angles of \triangle ABC together, we have: \begin{align}\angle ABC + \angle BAC + \angle ACB &= 180^\circ \\ ((90^\circ - b) + b) + (a + b) + (a + b) &= 180^\circ \\ 90^\circ + (a + b) + (a + b) &= 180^\circ\\ 90^\circ + 2(a + b) &= 180^\circ\\ 2(a + b) &= 180^\circ - 90^\circ\\ 2(a + b) &= 90^\circ\\ a + b &= \frac{90^\circ}{2}\\ a + b &= 45^\circ\end{align}
Therefore, since c = 45^\circ, we have that a + b = c.
You may also like
At a Glance
The area of a regular pentagon looks about twice as a big as the pentangle star drawn within it. Is it?
No Right Angle Here
Prove that the internal angle bisectors of a triangle will never be perpendicular to each other.

