Skip over navigation
Or search by topic
Number and algebra
Geometry and measure
Probability and statistics
Working mathematically
Advanced mathematics
For younger learners
Area L
Age 16 to 18
Challenge Level 





- Problem
- Getting Started
- Student Solutions
- Teachers' Resources
We received solutions from Amrit, Adithya, Guruvignesh and Agathiyan, all from Hymers College, and Pablo from Kings College Alicante. Here is Pablo's solution:
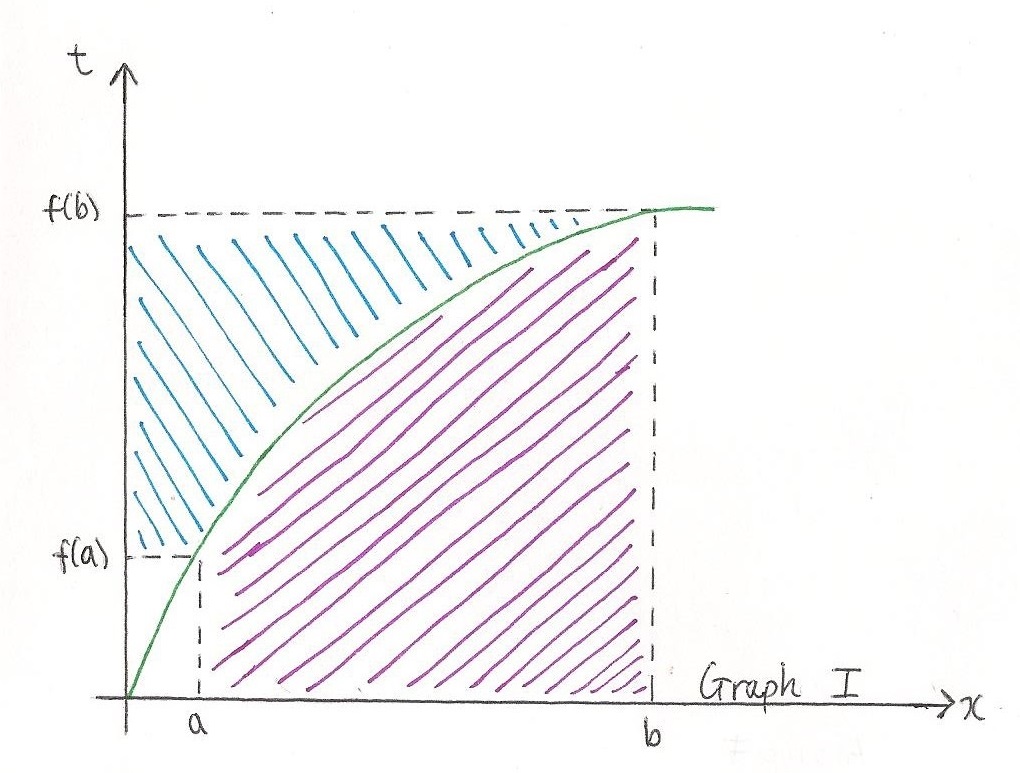
As you can see in Graph I, the areas in purple and blue add up to the big rectangle, bf(b), minus af(a), the small rectangle.
There is a change of variable in f^{-1}(t) because the area isn't taken
from the x-axis, but from the t-axis. f^{-1}(x) is the inverse of f(x). It is obtained either by switching variables and rearranging the equation again or by reflecting it in the line y=x.
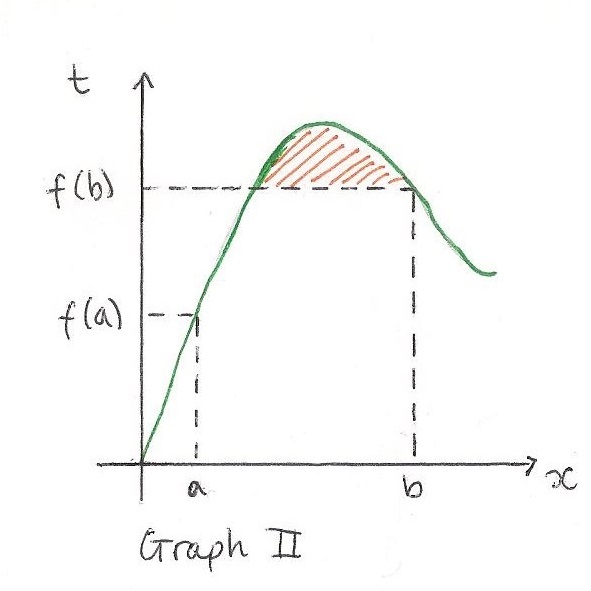
The reason why f(x) must be increasing in the interval a \leq x \leq b is shown in Graph II. If it weren't, if at some point it reached a turning point and began to decrease, the area (orange) at the top would not be accounted for.
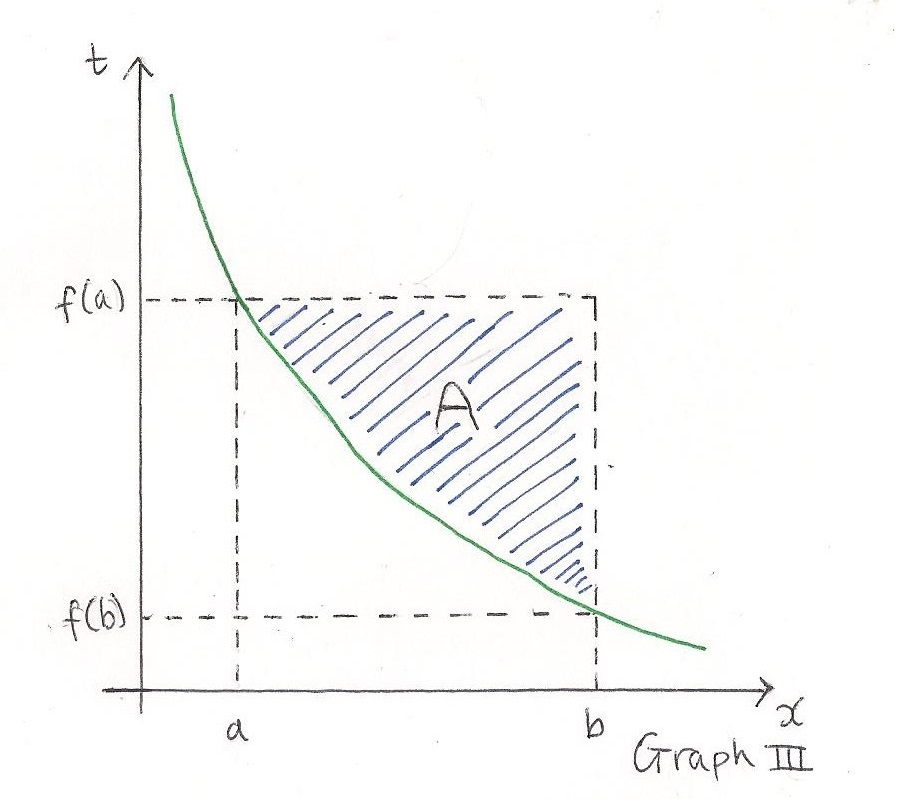
If f(x) is always decreasing in the interval a \leq x \leq b, a similar formula comes out, but through more algebraic manipulation. The area called A in Graph III is:
A = bf(a) - bf(b) - \int_{f(b)}^{f(a)} f^{-1}(t) d t
but also, A = bf(a) - af(a) \int_a^b f(x) d x
so bf(a) - bf(b) - \int_{f(b)}^{f(a)} f^{-1}(t) d = bf(a) - af(a) \int_a^b f(x) d x \int_a^b f(x) d x = bf(a) - af(a) - \left[bf(a) - bf(b) - \int_{f(b)}^{f(a)} f^{-1}(t) d t\right] \int_a^b f(x) d x = bf(b) - af(a) + \int_{f(b)}^{f(a)} f^{-1}(t) d t
The difference with the first formula is that \int_{f(b)}^{f(a)} f^{-1}(t) d t is added, not subtracted. Additionally, the boundaries are swapped, as f(b) is less than f(a).
First, we can find the value of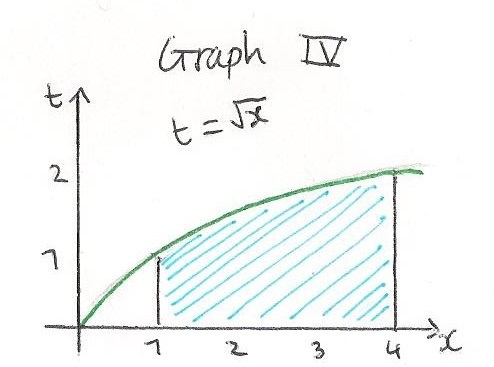 \int_1^4\sqrt t dt the conventional
\int_1^4\sqrt t dt the conventional
way:
\eqalign{\int_1^4 \sqrt x dx &= \left[\frac23 x^\frac32\right]_1^4 \\ &= \frac23 ( 4^\frac32 - 1^\frac32) \\ &= \frac{14}3}
Now using our formula:
\eqalign{\int_1^4 \sqrt x dx &= bf(b) - af(a) - \int_{f(a)}^{f(b)}f^{-1}(t)dt \\ &= 4\sqrt4 - 1\sqrt1 - \int_{\sqrt(1)}^{\sqrt(4)} t^2 dt \\ &= 8 - 1 - \left[\frac13 t^3 \right]_1^2 \\ &= 7 - \frac83 + \frac13 \\ &= \frac{14}3}
Which gives the same answer!
For \sin^{-1}(x), using Graph V: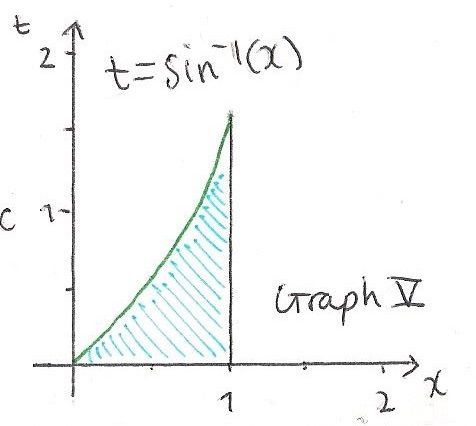
\eqalign{ \int_0^1 \sin^{-1}(x) dx &= bf(b) - af(a) - \int_{f(a)}^{f(b)}f^{-1}(t)dt \\ &= 1\times \sin^{-1}(1) - 0\times \sin^{-1}(0) - \int_{f(a)}^{f(b)} \sin(t) dt \\ &= \frac{\pi}2 - 0 - \int_0^{\frac{\pi}2} \sin(t) dt \\ &=\frac{\pi}2 - \left[-\cos(t)\right]_0^{\frac{\pi}2} \\ &= \frac{\pi}2 - \left[(-cos(\frac{\pi}2)-(-cos(0))\right]\\ &= \frac{\pi}2 - 1}

Another function that can be integrated using this method is ln(x):
\eqalign{\int_1^{e^2} ln(x) dx &= e^2ln(e^2) - 1ln(1) - \int_0^2 e^t dt \\ &= 2e^2 - 0 - \left[e^t\right]_0^2 \\ &= 2e^2 - (e^2 - e^0) \\ &= 2e^2 - e^2 + 1 \\ &= e^2 + 1}

As you can see in Graph I, the areas in purple and blue add up to the big rectangle, bf(b), minus af(a), the small rectangle.
There is a change of variable in f^{-1}(t) because the area isn't taken
from the x-axis, but from the t-axis. f^{-1}(x) is the inverse of f(x). It is obtained either by switching variables and rearranging the equation again or by reflecting it in the line y=x.

The reason why f(x) must be increasing in the interval a \leq x \leq b is shown in Graph II. If it weren't, if at some point it reached a turning point and began to decrease, the area (orange) at the top would not be accounted for.

If f(x) is always decreasing in the interval a \leq x \leq b, a similar formula comes out, but through more algebraic manipulation. The area called A in Graph III is:
A = bf(a) - bf(b) - \int_{f(b)}^{f(a)} f^{-1}(t) d t
but also, A = bf(a) - af(a) \int_a^b f(x) d x
so bf(a) - bf(b) - \int_{f(b)}^{f(a)} f^{-1}(t) d = bf(a) - af(a) \int_a^b f(x) d x \int_a^b f(x) d x = bf(a) - af(a) - \left[bf(a) - bf(b) - \int_{f(b)}^{f(a)} f^{-1}(t) d t\right] \int_a^b f(x) d x = bf(b) - af(a) + \int_{f(b)}^{f(a)} f^{-1}(t) d t
The difference with the first formula is that \int_{f(b)}^{f(a)} f^{-1}(t) d t is added, not subtracted. Additionally, the boundaries are swapped, as f(b) is less than f(a).
First, we can find the value of
 \int_1^4\sqrt t dt the conventional
\int_1^4\sqrt t dt the conventionalway:
\eqalign{\int_1^4 \sqrt x dx &= \left[\frac23 x^\frac32\right]_1^4 \\ &= \frac23 ( 4^\frac32 - 1^\frac32) \\ &= \frac{14}3}
Now using our formula:
\eqalign{\int_1^4 \sqrt x dx &= bf(b) - af(a) - \int_{f(a)}^{f(b)}f^{-1}(t)dt \\ &= 4\sqrt4 - 1\sqrt1 - \int_{\sqrt(1)}^{\sqrt(4)} t^2 dt \\ &= 8 - 1 - \left[\frac13 t^3 \right]_1^2 \\ &= 7 - \frac83 + \frac13 \\ &= \frac{14}3}
Which gives the same answer!
For \sin^{-1}(x), using Graph V:

\eqalign{ \int_0^1 \sin^{-1}(x) dx &= bf(b) - af(a) - \int_{f(a)}^{f(b)}f^{-1}(t)dt \\ &= 1\times \sin^{-1}(1) - 0\times \sin^{-1}(0) - \int_{f(a)}^{f(b)} \sin(t) dt \\ &= \frac{\pi}2 - 0 - \int_0^{\frac{\pi}2} \sin(t) dt \\ &=\frac{\pi}2 - \left[-\cos(t)\right]_0^{\frac{\pi}2} \\ &= \frac{\pi}2 - \left[(-cos(\frac{\pi}2)-(-cos(0))\right]\\ &= \frac{\pi}2 - 1}

Another function that can be integrated using this method is ln(x):
\eqalign{\int_1^{e^2} ln(x) dx &= e^2ln(e^2) - 1ln(1) - \int_0^2 e^t dt \\ &= 2e^2 - 0 - \left[e^t\right]_0^2 \\ &= 2e^2 - (e^2 - e^0) \\ &= 2e^2 - e^2 + 1 \\ &= e^2 + 1}

