Skip over navigation
Mean Geometrically
Age 16 to 18
Challenge Level 
$O$ is the centre of a circle with $A$ and $B$ two points NOT on a diameter. The tangents to $A$ and $B$ intersect at $C$. $CO$ cuts the circle at $D$ and a tangent through $D$ cuts $AC$ and $BC$ at $E$ and $F$.
What is the relationship between area of $ADBO$ and the areas of $ABO$ and $ACBO$?
You may also like
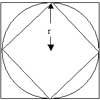
Approximating Pi
By inscribing a circle in a square and then a square in a circle find an approximation to pi. By using a hexagon, can you improve on the approximation?
Spokes
Draw three equal line segments in a unit circle to divide the circle into four parts of equal area.
