Skip over navigation
Well done Curt from Reigate College for this solution.
If G(x)=e^{f(x)}, by the chain rule:
G'(x)=e^{f(x)} \times f'(x).
As e is never zero, the gradient function of G(x) is zero when the gradient function of f(x) is zero and it always has the same sign as f'(x) so G(x) and f(x) have the same turning points.
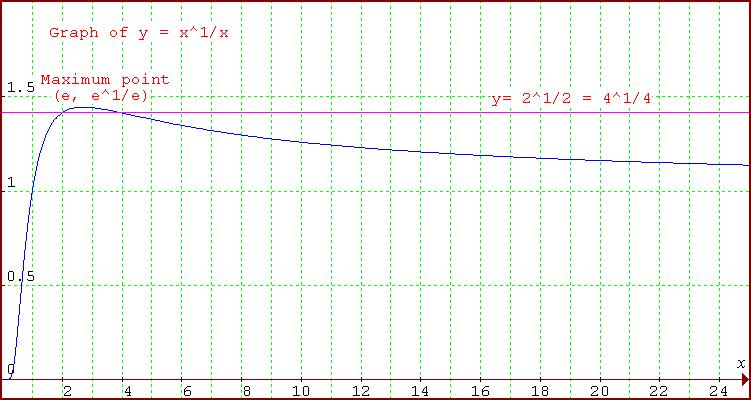
To answer the question of turning points of G(x)=x^{1\over x}= e^{1/x\log x} let's first find the derivative of {1\over x}\log x.
{d\over dx }f(x)={d\over dx }({1\over x}\log x) = {{1-\log x}\over x^2}.
The derivative is positive for \log x < 1, that is x< e, it is is zero when \log x = 1, x = e and it is negative for x> e. Hence the function is increasing for x< e and decreasing for x> e.
{d^2\over dx^2 }({1\over x}\log x)= {{-x-2x(1-\log x)}\over x^4 }= {{2\log x -3}\over x^3}.
At x=e the second derivative of f(x) is -1/e^3 which is negative so f(x) has a maximum and so G(x) has a maximum. Therefore (e, e^{1/e}) is a maximum point of G(x)=x^{1\over x}.
To prove \lim x^{1\over x} \rightarrow 1 as x\rightarrow \infty we may use the similar result for the discrete case n^{1\over n}. As the graph of f(x)=x^{1\over x} is decreasing as x\to \infty for each value of x there is a value of n for which n^{1/n}< x^{1/x} and vice versa so both tend to the same limit which is 1.
What if x\rightarrow 0? Well, as suggested in the question, letting 1/x=t in x^{1\over x}, one can note that as x\rightarrow 0, t\rightarrow \infty. Writing t=1/x, x^{1/x} = \left ({1\over t}\right )^t = {1\over t^t}. As x\to 0 we have t\to \infty and t^t \to \infty so x^{1/x} = {1\over t^t} \to 0.
Hence the graph of y=x^{1/x} is always above the line y=0 although the function is undefined at x=0.


Or search by topic
Number and algebra
Geometry and measure
Probability and statistics
Working mathematically
Advanced mathematics
For younger learners
Exponential Trend
Age 16 to 18
Challenge Level 





- Problem
- Getting Started
- Student Solutions
- Teachers' Resources
Well done Curt from Reigate College for this solution.
If G(x)=e^{f(x)}, by the chain rule:
G'(x)=e^{f(x)} \times f'(x).
As e is never zero, the gradient function of G(x) is zero when the gradient function of f(x) is zero and it always has the same sign as f'(x) so G(x) and f(x) have the same turning points.
To answer the question of turning points of G(x)=x^{1\over x}= e^{1/x\log x} let's first find the derivative of {1\over x}\log x.
{d\over dx }f(x)={d\over dx }({1\over x}\log x) = {{1-\log x}\over x^2}.
The derivative is positive for \log x < 1, that is x< e, it is is zero when \log x = 1, x = e and it is negative for x> e. Hence the function is increasing for x< e and decreasing for x> e.
{d^2\over dx^2 }({1\over x}\log x)= {{-x-2x(1-\log x)}\over x^4 }= {{2\log x -3}\over x^3}.
At x=e the second derivative of f(x) is -1/e^3 which is negative so f(x) has a maximum and so G(x) has a maximum. Therefore (e, e^{1/e}) is a maximum point of G(x)=x^{1\over x}.
To prove \lim x^{1\over x} \rightarrow 1 as x\rightarrow \infty we may use the similar result for the discrete case n^{1\over n}. As the graph of f(x)=x^{1\over x} is decreasing as x\to \infty for each value of x there is a value of n for which n^{1/n}< x^{1/x} and vice versa so both tend to the same limit which is 1.
What if x\rightarrow 0? Well, as suggested in the question, letting 1/x=t in x^{1\over x}, one can note that as x\rightarrow 0, t\rightarrow \infty. Writing t=1/x, x^{1/x} = \left ({1\over t}\right )^t = {1\over t^t}. As x\to 0 we have t\to \infty and t^t \to \infty so x^{1/x} = {1\over t^t} \to 0.
Hence the graph of y=x^{1/x} is always above the line y=0 although the function is undefined at x=0.
You may also like
Folium of Descartes
Investigate the family of graphs given by the equation x^3+y^3=3axy for different values of the constant a.
Witch of Agnesi
Sketch the members of the family of graphs given by y = a^3/(x^2+a^2) for a=1, 2 and 3.

