Skip over navigation
This solution is from Andrei from Tudor Vianu National College, Bucharest, Romania. Calculating F_1, F_2,. . . , F_7, I obtain: F_1 = 1;\ F_2 = 1;\ F_3 = 2;\ F_4 =3;\ F_5 = 5;\ F_6 = 8;\ F_7 = 13.
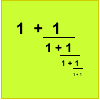

Or search by topic
Number and algebra
Geometry and measure
Probability and statistics
Working mathematically
Advanced mathematics
For younger learners
Plus or Minus
Age 16 to 18
Challenge Level 





- Problem
- Getting Started
- Student Solutions
- Teachers' Resources
This solution is from Andrei from Tudor Vianu National College, Bucharest, Romania. Calculating F_1, F_2,. . . , F_7, I obtain: F_1 = 1;\ F_2 = 1;\ F_3 = 2;\ F_4 =3;\ F_5 = 5;\ F_6 = 8;\ F_7 = 13.
Calculating F_{n-1}F_{n+1} for some values of n:
\begin{eqnarray} n = 2: F_1F_3 &= 2 = 1 + 1 = (F_2)^2 + 1 \\ n
= 3: F_2F_4 &= 3 = 4 - 1 = (F_3)^2 - 1 \\ n = 4: F_3F_5 &=
10 = 9 + 1 = (F_4)^2 + 1 \\ n = 5: F_4F_6 &= 24 = 25 - 1 =
(F_5)^2 - 1 \\ n = 6: F_5F_7 &=65 = 64 + 1 = (F_6)^2 + 1 .
\end{eqnarray}
Observing this, I try to prove the conjecture that:
F_{n-1}F_{n+1}= (F_n)^2 + (-1)^n.
Using the general formula for
F_{n-1} and F_{n+1} in terms of \alpha and \beta, the roots
of the equation x^2-x-1=0, I find: \begin{eqnarray}
F_{n-1}F_{n+1} &= {1\over
5}(\alpha^{n-1}-\beta^{n-1})(\alpha^{n+1}-\beta^{n+1}) \\ &=
{1\over 5}[ \alpha ^{2n} +
\beta^{2n}-(\alpha^2+\beta^2)(\alpha\beta)^{n-1}] \\ &= {1\over
5}[(\alpha^n-\beta^n)^2+ 2(\alpha\beta)^n
-(\alpha^2+\beta^2)(\alpha\beta)^{n-1}] \\ &= F_n^2 -{1\over
5}(\alpha\beta)^{n-1}(\alpha-\beta)^2 . \end{eqnarray}
At this
point we use the fact that \alpha\beta=-1 and \alpha-\beta=\sqrt
5 which gives the result F_{n+1}F_{n-1}=F_n^2 +(-1)^n
thus
proving the conjecture.
You may also like
Continued Fractions I
An article introducing continued fractions with some simple puzzles for the reader.
Fibonacci Factors
For which values of n is the Fibonacci number fn even? Which Fibonnaci numbers are divisible by 3?

