The Twelve Pointed Star Game
The Twelve Pointed Star Game
This game is for two or more players.
You will need a copy of the star board, counters and two 1-6 dice.
Each player chooses three numbers on the star. (If you play with more than four players, each player chooses two numbers.)
Players then take it in turns to roll two dice and add the scores.
The player who has chosen that number puts a counter on the appropriate circle.
The winner is the first player to have counters on all three circles belonging to one of their chosen numbers.
For example I'm playing with my friend Zac. I choose the numbers 2, 4 and 6; Zac chooses 7, 8 and 9.
Zac rolls the dice and it's a 4 and a 2, which makes a total of 6.
This means I can put a counter on one of the circles next to the 6.
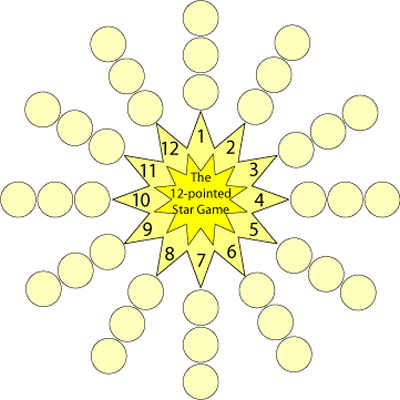
Play the game a few times.
Which are good numbers to choose? Why?
Which are poor numbers to choose? Why?
Which is the worst number to choose? Why?
Why do this problem?
This game offers a good context in which to explore possible outcomes and to think systematically about what scores are possible. It will be important for learners to develop a recording or listing system that they are happy with, in order to find all the possible ways in which the different totals can be made.
Possible approach
You could start by encouraging the group to try playing the game a few times and then pool their results of 'winning numbers'. It is not necessary to have the star or counters - you could just write the numbers $1$ to $12$ on a piece of paper and put ticks against the numbers that come up. However, it is more appealing to use the star. Here is a coloured copy of the board which could be printed off for pupils to use and here is one in black and white that can be photocopied.
Which are good numbers to choose? Why?
Which are poor numbers to choose? Why?
Which is the worst number to choose? Why?
Key questions
Possible extension
Pupils could be challenged to make a version of the game which was fairer.
Possible support
Some children will find it useful to manipulate dice as they work out the possible outcomes.
Related Collections
You may also like
Prompt Cards
These two group activities use mathematical reasoning - one is numerical, one geometric.
Consecutive Numbers
An investigation involving adding and subtracting sets of consecutive numbers. Lots to find out, lots to explore.
Exploring Wild & Wonderful Number Patterns
EWWNP means Exploring Wild and Wonderful Number Patterns Created by Yourself! Investigate what happens if we create number patterns using some simple rules.