Skip over navigation
Flight Path
Age 16 to 18
Challenge Level 
 |
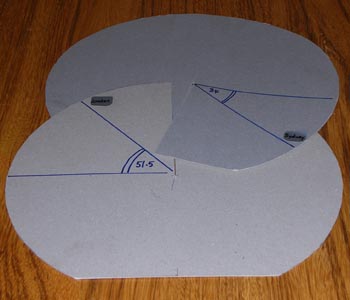A model to demonstrate
latitude and longitude
Cut 2 discs of cardboard.
Cut a radial slit in each so that they slot together as
shown.
Mark your chosen cities and their angles of latitude. In the
illustration London at $51.5^o$ North and Sydney at $34^o$ South
are shown.
Cut off a small segment at the South Pole so that the model
stands up.
Adjust the angle between the two planes in the model to show
the difference in their angles of longitude.
|
 |
Take the origin at the centre of the earth, the x-axis through
the Greenwich Meridian at the equator, the y-axis through longitude
$90^o$ East at the equator and the z-axis through the North
Pole.
London is approximately on the Greenwich Meridian so the 3-D
coordinates of London are $(R\cos 51.5^o, 0, R\sin 51.5^o$).
|
As an extension to this question you might like to calculate
the distance between Cape Town ($18^o$E, $34^o$S) and Sydney
($151^o$E, $34^o$S) along the great circle and find how much
shorter it is than the distance around the line of latitude
$34^o$S.
You may also like
2D-3D
Two circles of equal size intersect and the centre of each circle is on the circumference of the other. What is the area of the intersection? Now imagine that the diagram represents two spheres of equal volume with the centre of each sphere on the surface of the other. What is the volume of intersection?
The Dodecahedron Explained
What is the shortest distance through the middle of a dodecahedron between the centres of two opposite faces?
When the Angles of a Triangle Don't Add up to 180 Degrees
This article outlines the underlying axioms of spherical geometry giving a simple proof that the sum of the angles of a triangle on the surface of a unit sphere is equal to pi plus the area of the triangle.