Dotty Circle
Watch the film below.
Try to describe what happens in the film to someone else.
Imagine the dot starts at the point (1,0), turns through $20^\circ$ and then stops:
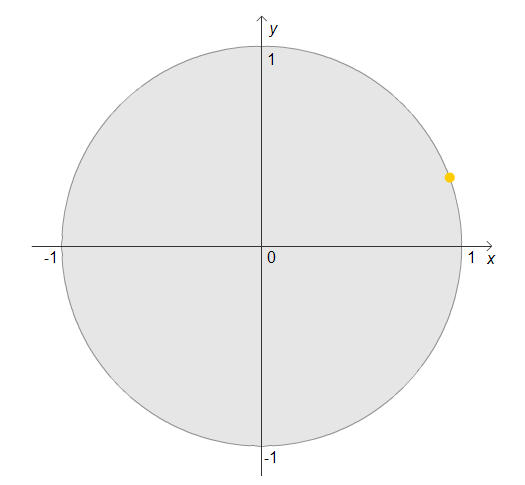
If the point now carries on, through how many degrees must it turn to finish the same height above or below the horizontal axis as it was when it had gone through $20^\circ$?
Can you find a general rule for explaining when the dot will be this same distance from the horizontal axis?
You might like to try Round and Round and Round after this problem.
You may also like
Counting Counters
Take a counter and surround it by a ring of other counters that MUST touch two others. How many are needed?
Cuisenaire Squares
These squares have been made from Cuisenaire rods. Can you describe the pattern? What would the next square look like?
Doplication
We can arrange dots in a similar way to the 5 on a dice and they usually sit quite well into a rectangular shape. How many altogether in this 3 by 5? What happens for other sizes?