Skip over navigation
We first encounter the function sin(x) when using right-angled triangles, where sine of an angle is defined to be the ratio of the length of the side opposite the angle to the length of the hypotenuse. Clearly this ratio must give a number which varies continuously between 0 and 1 as the angle varies from 0 to 90 degrees .
However, your calculator will give you a value of sin(x) for any value of x you care to choose, no matter how big or small. Calculators do not do this by 'drawing triangles'. Instead they approximate sin(x) by a polynomial function. In this problem we shall investigate the properties of these polynomials.
Look at an approximation by a cubic polynomial:
\sin(x)\approx a + b x + c x^2+ d x^3\quad\quad\mbox{where }\, a, b, c \,\mbox{ and } \, d \,\mbox{ are constants}
Extend your solution to 4th, 5th and higher order polynomials.
Test the accuracy of your final solution over a range 0 to \pi radians.
Why is it sufficient to work within this range?
Extension : Repeat this challenge to find approximations for \cos(x) and \log(1+x).
Discussion point : Why does it make sense to be able to approximate \sin(x) by a polynomial? What other functions do you think would have polynomial approximations? Do you think that all functions can be approximated by polynomials?
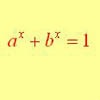
Or search by topic
Number and algebra
Geometry and measure
Probability and statistics
Working mathematically
Advanced mathematics
For younger learners
Building Approximations for Sin(x)
Age 16 to 18
Challenge Level 





We first encounter the function sin(x) when using right-angled triangles, where sine of an angle is defined to be the ratio of the length of the side opposite the angle to the length of the hypotenuse. Clearly this ratio must give a number which varies continuously between 0 and 1 as the angle varies from 0 to 90 degrees .
However, your calculator will give you a value of sin(x) for any value of x you care to choose, no matter how big or small. Calculators do not do this by 'drawing triangles'. Instead they approximate sin(x) by a polynomial function. In this problem we shall investigate the properties of these polynomials.
Look at an approximation by a cubic polynomial:
\sin(x)\approx a + b x + c x^2+ d x^3\quad\quad\mbox{where }\, a, b, c \,\mbox{ and } \, d \,\mbox{ are constants}
(where x
is in radians). By experimenting with the numerical values of
\sin(x) what values would you suggest for the constants?
[Aside: Why is it not
possible to 'solve' for these constants?]
Extend your solution to 4th, 5th and higher order polynomials.
Test the accuracy of your final solution over a range 0 to \pi radians.
Why is it sufficient to work within this range?
Extension : Repeat this challenge to find approximations for \cos(x) and \log(1+x).
Discussion point : Why does it make sense to be able to approximate \sin(x) by a polynomial? What other functions do you think would have polynomial approximations? Do you think that all functions can be approximated by polynomials?
You may also like
What Do Functions Do for Tiny X?
Looking at small values of functions. Motivating the existence of the Taylor expansion.
Equation Attack
The equation a^x + b^x = 1 can be solved algebraically in special cases but in general it can only be solved by numerical methods.

