Skip over navigation

Or search by topic
Number and algebra
Geometry and measure
Probability and statistics
Working mathematically
Advanced mathematics
For younger learners
ACE, TWO, THREE...
Age 11 to 14
Challenge Level 





- Problem
- Getting Started
- Student Solutions
- Teachers' Resources
Lots of people sent in correct solutions to this card problem. Many people developed Charlie's thoughts into a full solution. Well done to James from Chase Terrace Technology College, Evan from Muhlenberg Middle
School, Barnaby from Devonshire Primary School, Daisy, Bobby, Molly, Bethany, Anya and
Rosie from All Saints CofE Junior School Hessle and Richard from Worplesdon
School who did this! Here is their solution:
To represent the unknown cards write thirteen places on the page where the values will go:
_ _ _ _ _ _ _ _ _ _ _ _ _
Now count down three places for A-C-E and write 'A' for Ace in the third place, because this is where Charlie showed the Ace to be.
_ _ A _ _ _ _ _ _ _ _ _ _
Keep counting T-W-O (three places) for 2, T-H-R-E-E (five places)
_ _ A _ _ 2 _ _ _ _ 3 _ _
Now to count four places for F-O-U-R we need to go back to the beginning when we reach the end, this is because Charlie puts the cards he counts past on the bottom of the pack.
_ 4 A _ _ 2 _ _ _ _ 3 _ _
And for counting out F-I-V-E, skip the places already occupied by card values; this is because Charlie removes the cards when he finds them, they're not counted again.
_ 4 A _ _ 2 _ 5 _ _ 3 _ _
If you keep counting out the names of the cards you eventually get to the answer:
Q 4 A 8 K 2 7 5 10 J 3 6 9
Fantastic! Esperanza from Hamburg, Kendyl from Kelly Elementary School Wyoming, Michael from Cloverdale Catholic School Canada, Tayla from Farm Cove Intermediate and Milly from Grasmere Primary School found the correct answer with similar methods, and some people used letters to represent the missing card values - this is a great way to do it too.
Reiss from Burton Borough School had the excellent idea of working backwards from King to Ace. Here is his solution:
Have a group of cards on the table, at the bottom is an "Ace" all in order up to a "King" which should be at the top. Start off by putting the top card of the ordered pile in your hand and then pick up the second one "Queen" then put it on top of the "King" in your hand. Spelling "Queen" is important. The Queen is a "Q" then take the bottom card in your hand then place it above the top one in your hand and that is the "U." Keep doing that until you have completed the spelling "Queen." Then you pick up the next card in the ordered pile "Jack" and place it on top of the cards in your hand and do the same again and do the same again until you have completed the spelling "Jack." Do the same thing until all of the cards in the ordered pile are in your hand. Make sure you have done it using the correct spelling of each number, otherwise it might go wrong when you come to do it.
Can you see why his method works?
Patrick and H&H also submitted solutions for saying the card names in French and German. Brilliant. Can you see why this trick will work in any language provided you order the cards correctly? Try to find the right order for a language of your choice!
To represent the unknown cards write thirteen places on the page where the values will go:
_ _ _ _ _ _ _ _ _ _ _ _ _
Now count down three places for A-C-E and write 'A' for Ace in the third place, because this is where Charlie showed the Ace to be.
_ _ A _ _ _ _ _ _ _ _ _ _
Keep counting T-W-O (three places) for 2, T-H-R-E-E (five places)
_ _ A _ _ 2 _ _ _ _ 3 _ _
Now to count four places for F-O-U-R we need to go back to the beginning when we reach the end, this is because Charlie puts the cards he counts past on the bottom of the pack.
_ 4 A _ _ 2 _ _ _ _ 3 _ _
And for counting out F-I-V-E, skip the places already occupied by card values; this is because Charlie removes the cards when he finds them, they're not counted again.
_ 4 A _ _ 2 _ 5 _ _ 3 _ _
If you keep counting out the names of the cards you eventually get to the answer:
Q 4 A 8 K 2 7 5 10 J 3 6 9
Fantastic! Esperanza from Hamburg, Kendyl from Kelly Elementary School Wyoming, Michael from Cloverdale Catholic School Canada, Tayla from Farm Cove Intermediate and Milly from Grasmere Primary School found the correct answer with similar methods, and some people used letters to represent the missing card values - this is a great way to do it too.
Reiss from Burton Borough School had the excellent idea of working backwards from King to Ace. Here is his solution:
Have a group of cards on the table, at the bottom is an "Ace" all in order up to a "King" which should be at the top. Start off by putting the top card of the ordered pile in your hand and then pick up the second one "Queen" then put it on top of the "King" in your hand. Spelling "Queen" is important. The Queen is a "Q" then take the bottom card in your hand then place it above the top one in your hand and that is the "U." Keep doing that until you have completed the spelling "Queen." Then you pick up the next card in the ordered pile "Jack" and place it on top of the cards in your hand and do the same again and do the same again until you have completed the spelling "Jack." Do the same thing until all of the cards in the ordered pile are in your hand. Make sure you have done it using the correct spelling of each number, otherwise it might go wrong when you come to do it.
Can you see why his method works?
Patrick and H&H also submitted solutions for saying the card names in French and German. Brilliant. Can you see why this trick will work in any language provided you order the cards correctly? Try to find the right order for a language of your choice!
Related Collections
You may also like
All in the Mind
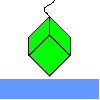
Imagine you are suspending a cube from one vertex and allowing it to hang freely. What shape does the surface of the water make around the cube?
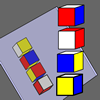
Instant Insanity
Given the nets of 4 cubes with the faces coloured in 4 colours, build a tower so that on each vertical wall no colour is repeated, that is all 4 colours appear.
Is There a Theorem?
Draw a square. A second square of the same size slides around the first always maintaining contact and keeping the same orientation. How far does the dot travel?

