Skip over navigation
Zin Obelisk
Age 11 to 14
Challenge Level 
Why do this problem?
This problem challenges students to make sense of a lot of random information and to apply their knowledge of proportionality and measures to answer the question.Possible approach
This is an ideal activity for students to carry out in small groups of 3 or 4. Some groups work better when its members have been allocated clear roles. A handout detailing possible roles that has been used successfully by some schools is available as a Word document or in pdf format.
Print and cut out sets of cards with the information and distribute to members of each group (they are available here as Word documents on in pdf format ).
The task for each "team" is to determine on which day of the week the obelisk was completed. There may be some disagreement between the groups so let them know that they will be expected to justify their answers.
Each group could be asked to set out their working/justifications on a large sheet of flipchart paper before being asked to talk to the whole class. How did they group the information to make it more manageable? How did they decide that some of the information was unnecessary?
Each group could be asked to set out their working/justifications on a large sheet of flipchart paper before being asked to talk to the whole class. How did they group the information to make it more manageable? How did they decide that some of the information was unnecessary?
Key questions
What behaviour helped the group accomplish the task?
What behaviour hindered the group in completing the task?
How did leadership emerge in the team?
Who participated most? Who participated least?
What feelings did you experience as the task progressed?
What suggestions would you make to improve team performance?
Possible extension
A possibly more difficult problem that again requires students to draw conclusions from information is Nutrition and Cycling.Possible support
Possible ways to simplify the problem could involve:
- offering students the information in an organised form
- removing information that is not required.
You may also like
Rule of Three
If it takes four men one day to build a wall, how long does it take 60,000 men to build a similar wall?
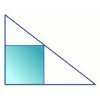
How Big?
If the sides of the triangle in the diagram are 3, 4 and 5, what is the area of the shaded square?