Counting Cogs

Counting Cogs

This problem has been designed to be worked on in a group. For more details about how you might go about doing this, please read the Teachers' Notes.

Here are nine different cogs:




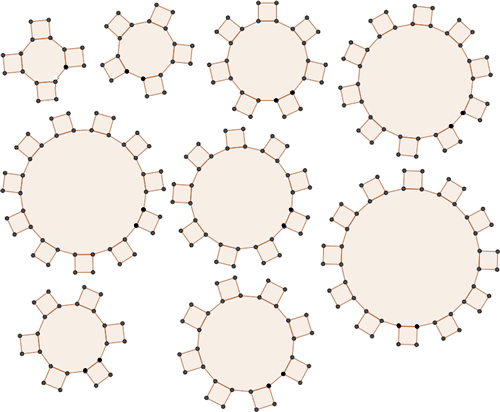
If you coloured one tooth on each cog, which pairs of cogs let the coloured tooth go into every 'gap' on the other cog?

Which pairs do not let this happen? Why?

Can you explain how to determine which pairs will work, and why?

You could cut out the cogs from these sheets to try out your ideas.

Why do this problem?

Possible approach

This is an ideal problem for learners to tackle in groups of four. Allocating these clear roles (Word, pdf) can help the group to work in a purposeful way - success on this task could be measured by how effectively members of the group work together as well as by the solutions they reach.

Introduce the four group roles to the class. It may be appropriate, if this is the first time the class has worked in this way, to allocate particular roles to particular children. If the class works in roles over a series of lessons, it is desirable to make sure everyone experiences each role over time.

For suggestions of team-building maths tasks for use with classes unfamiliar with group work, take a look at this article and the accompanying resources.

Give each group a copy of this sheet, which outlines the task. The idea is for them to read it together to find out what to do. Cut out a set of cogs for each group using this sheet and give them out so each person in a group has two or three cogs. Children should begin by working individually, investigating several pairs of cogs, then they will pool their findings as a group so that they have worked on all combinations of cogs.

Explain that each group will be expected to report back at the end of the session, showing the patterns they noticed, at least one conjecture they have and at least one question. Exploring the full potential of this task is likely to take more than one lesson, allowing time in each lesson for children to feed back ideas and share their thoughts and questions. Ask each group to record their reasoning, conjectures, explanations and any generalisations on a large sheet of paper (for example flipchart paper) in preparation for reporting back.

There are many ways that groups can report back. Here are just a few suggestions:

• Every group is given a couple of minutes to report back to the whole class. Learners can seek clarification and ask questions. After each presentation, children are invited to offer positive feedback. Finally, pupils can suggest how the group could have improved their work on the task.
• Everyone's posters are put on display at the front of the room, but only a couple of groups are selected to report back to the whole class. Feedback and suggestions can be given in the same way as above. Additionally, children from the groups which don't present can be invited to share at the end anything they did differently.
• Two children from each group move to join an adjacent group. The two "hosts" explain their findings to the two "visitors". The "visitors" act as critical friends, requiring clear mathematical explanations and justifications. The "visitors" then comment on anything they did differently in their own group.

Key questions

Which cogs have you found that work so far?
Which pairs didn't work? Can you explain why?
How could you predict whether a pair will work before you try them?
What questions would you like to ask?

Possible extension

Children could begin to work on a question that they have, or a question posed by another group.

Possible support

By working in groups with clearly assigned roles we are encouraging students to take responsibility for ensuring that everyone understands before the group moves on.