Skip over navigation
You need to be in a team of four for this task. The idea is to complete the challenge yourself but with support and advice from other members of your team.
A nearby adult can assess how well the team is good at:
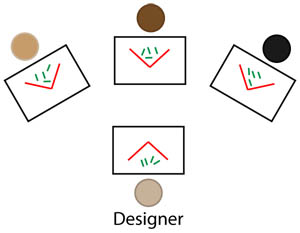
As you see above, you need to arrange the desks and some props so that everyone can see everyone else but no one can see what anyone else is building. One possibility is for the designer to face the other three team members and make the design inside a box whilst team members try to recreate the design behind books or folders used as screens.
Choose someone in the group to be the designer.
Stick Images
Age 7 to 14
Challenge Level 
You need to be in a team of four for this task. The idea is to complete the challenge yourself but with support and advice from other members of your team.
A nearby adult can assess how well the team is good at:
- helping others to do things for themselves
- responding to the needs of others - everybody helps everybody
- explaining by telling how.
As you see above, you need to arrange the desks and some props so that everyone can see everyone else but no one can see what anyone else is building. One possibility is for the designer to face the other three team members and make the design inside a box whilst team members try to recreate the design behind books or folders used as screens.
Choose someone in the group to be the designer.
You will need four sets of lolly sticks (or similar) - up to ten in each set. Give one set of lolly sticks to the designer and one set to each of the other team members.
The designer creates a lolly stick design so that it is hidden from the rest of the team but as s/he makes the design, s/he explains what it looks like so that the rest of the team can make a copy of the same design.
Team members can ask questions about the design at any time and the designer answers in as helpful a way as possible.
When a team member thinks s/he has a completed design, s/he asks the designer to check. If it is right s/he can then aid the designer in answering questions. If it is not correct, the task continues.
Remember that all help has to be given without the designer seeing the design of the person asking the question.
At any point the task can be brought to an end to discuss the success of the questioning and answering, and how it helped or hindered completion of the task.
You may also like
Summing Consecutive Numbers
15 = 7 + 8 and 10 = 1 + 2 + 3 + 4. Can you say which numbers can be expressed as the sum of two or more consecutive integers?
Where Can We Visit?
Charlie and Abi put a counter on 42. They wondered if they could visit all the other numbers on their 1-100 board, moving the counter using just these two operations: x2 and -5. What do you think?
Path to the Stars
Is it possible to draw a 5-pointed star without taking your pencil off the paper? Is it possible to draw a 6-pointed star in the same way without taking your pen off?