Skip over navigation
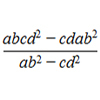

Or search by topic
Number and algebra
Geometry and measure
Probability and statistics
Working mathematically
Advanced mathematics
For younger learners
Number Rules - OK
Age 14 to 16
Challenge Level 





- Problem
- Getting Started
- Student Solutions
- Teachers' Resources
Try some numbers first - are you convinced?
Can you see how the pictorial and algebraic methods below can be used to explain why the sum of three consecutive numbers is always a multiple of three?
Method 1:

Method 2:
n+(n+1) + (n+2) = 3n + 3 = 3(n+1)
Try to use similar methods to explain the statements in the problem.
You may also like
DOTS Division
Take any pair of two digit numbers x=ab and y=cd where, without loss of generality, ab > cd . Form two 4 digit numbers r=abcd and s=cdab and calculate: {r^2 - s^2} /{x^2 - y^2}.
Novemberish
a) A four digit number (in base 10) aabb is a perfect square. Discuss ways of systematically finding this number. (b) Prove that 11^{10}-1 is divisible by 100.

