Or search by topic
Number and algebra
Geometry and measure
Probability and statistics
Working mathematically
Advanced mathematics
For younger learners
Going for Gold in 2012



- Problem
- Getting Started
- Student Solutions
- Teachers' Resources
Going for Gold in 2012
Here are the top ten nations in the table of medal winners for the 2012 Olympic Games:
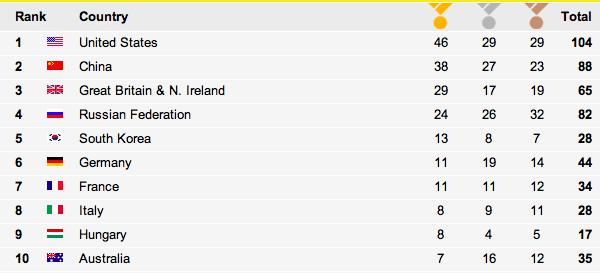
Is your own nation in the list?
If not find the data on the internet and compare it with the table.
How do you think the positions have been decided?
Could the results be presented differently to give another nation the top place?
How would this affect other results in the table?
Why do this problem?
This problem is intended to encourage children to develop their skills in data analysis and to be critical of the way in which data are presented to them.
Possible approach
Present the children with the table to look at and invite them to tell you about what it shows. Explain where it comes from and why it might have been made. Encourage the children to look at the variation in the numbers of medals of different types or the totals or both.
Key questions
How are the nations ordered?
Are there nations that have the same numbers of gold medals? Of silver medals? Of bronze medals?
Are they next to each other in the table? Why do you think this is so?
Do you think the system for ordering the nations is fair? Why?
Possible extension
Encourage the children to think creatively about scoring systems such as 3 points for a gold, 2 for a silver and 1 for a bronze and investigate the impact this would have on the order.
Further investigations might help the children to offer each nation advice about the sports they should focus on to maximise their position in the table. They would need to find and examine further data to do this.
Can you work out a system that is 'fair' but puts your favourite nation at the top?
It might be interesting to consider other factors that affect perception of performance at the Olympics, such as population and wealth - see this Plus article for further discussion.
Possible support
Get children to tell you and each other about the meaning of the data in the table. Ask straightforward questions such as:
How many gold medals does China have?
Which country is top of the table?
Once they have a good idea of the meaning of the data, they may be able to go on to consider the main task.
You may also like
Real Statistics
Have a look at this table of how children travel to school. How does it compare with children in your class?
Compare the Squares
In this problem you will do your own poll to find out whether your friends think two squares on a board are the same colour or not.
Three Spinners
These red, yellow and blue spinners were each spun 45 times in total. Can you work out which numbers are on each spinner?

