Factors and Multiples Game

Age 7 to 16

Challenge Level Yellow star

Many thanks to everyone who shared their solutions with our team. We were very impressed by your resilience and perseverence to produce the chains that you submitted to NRICH. Several of you sent in more than one solution, substituting an earlier chain with an even longer one after spending more time on the challenge. Well done to you all!

We've published below a selection of increasingly long chains which have a screenshot showing the full chain, or a list of its factors and multiples. We hope this list inspires you to keep working on your own solutions and perhaps consider the length of the longest possible chain, and the reasons for your answer.

Let's begin with Montsaye Community College in Northamptonshire where their students took on the challenge of generating the longest possible chain.

Gabrielle and Lauren reached a total of 50 numbers:

Aravindan, from GHS in India, shared this chain of 51 numbers:

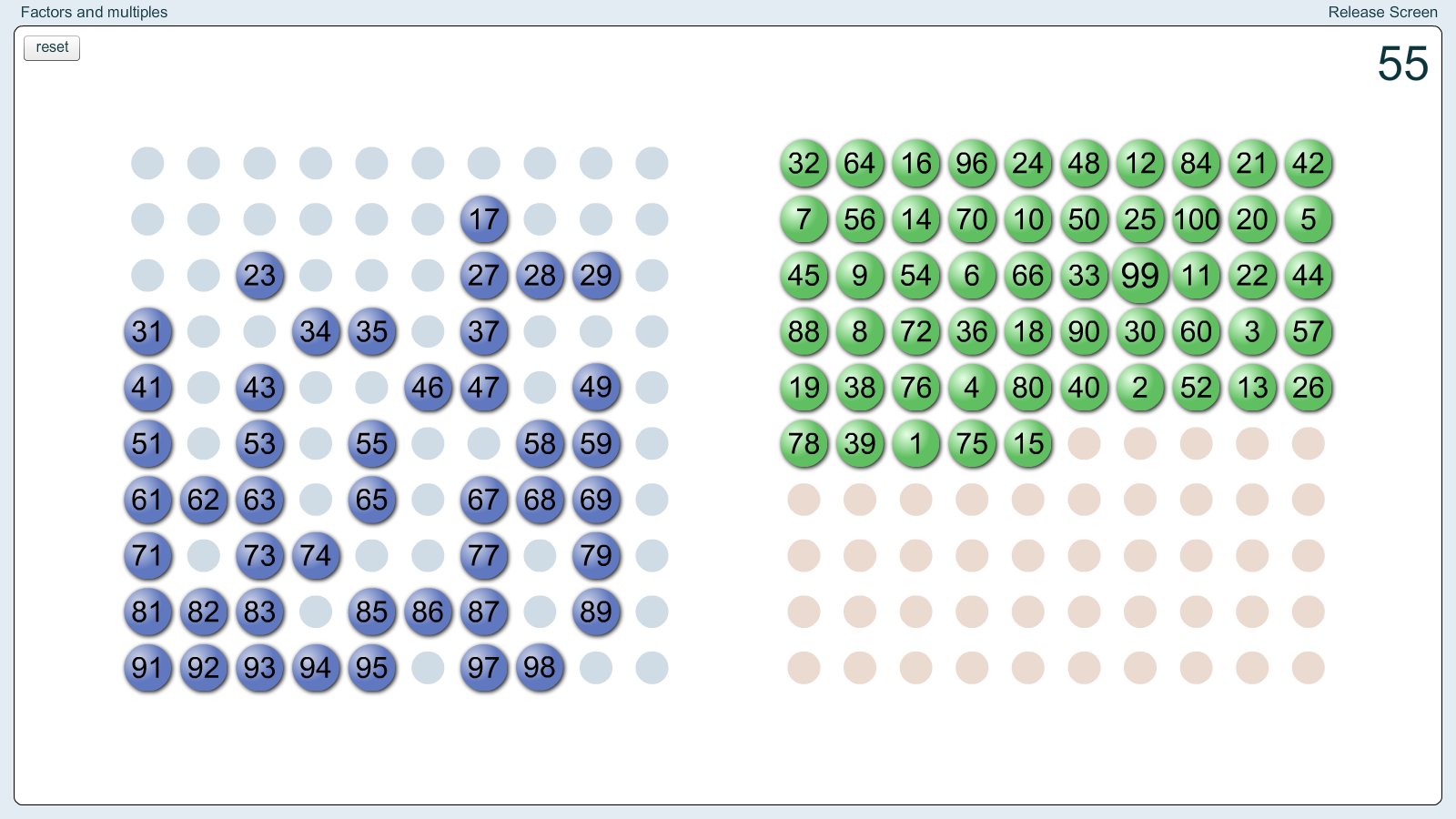
Makenzie, from Mountsaye Community College, managed to improve on this with a chain of 55 numbers:

Sophie and Tasmin, also from Mountsaye, managed to improve on that with this chain of 56 numbers:

Then, Gabrielle and Lauren managed to improve on their earlier effort with this new chain featuring 59 numbers:

Aravindan improved on his previous effort to produce this chain of 60 numbers:

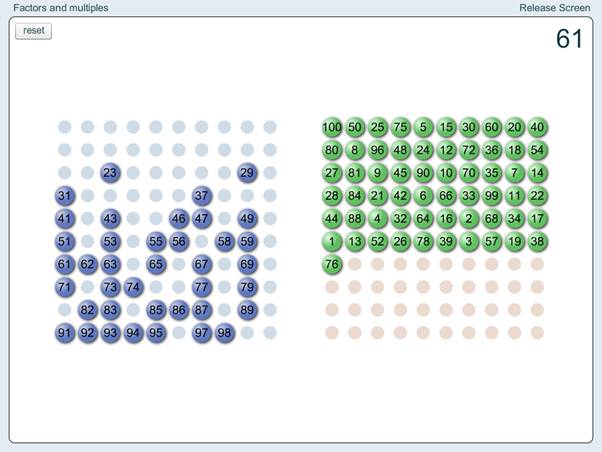
Abigail from Ridgewood School managed a chain of 61 numbers:

Abigail's solution

Alfie, Manuel, Jack and Emilio from Newhall School in Chelmsford, Essex, worked as a team to also produce a chain of 61:

Newhall solution

A.H. from Manorfield Primary School has improved on this by finding a chain of 63 numbers:

90-9-99-33-66-11-44-22-88-8-80-40-10-100-20-60-30-15-75-25-50-5-35-7-70-14-56-28-84-21-42-6-78-39-13-26-52-4-68-34-17-51-1-46-92-23-69-3-57-19-38-76-2-24-72-18-36-12-48-16-24-32-96

This solution came in from Ralph and Max at the Institut International de Lancy in Switzerland. Their teacher said they did not use a computer or calculator. They made a chain of 65, which seems to be a very popular answer.

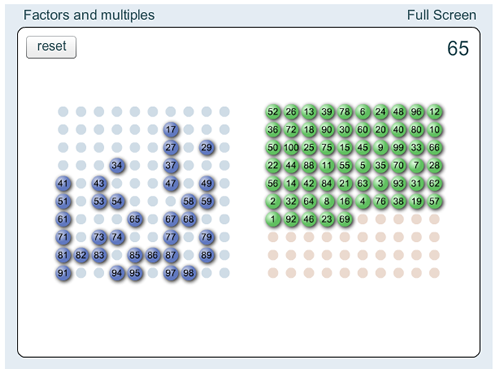
Evie from Deansfield Primary School created a chain of 65 numbers:

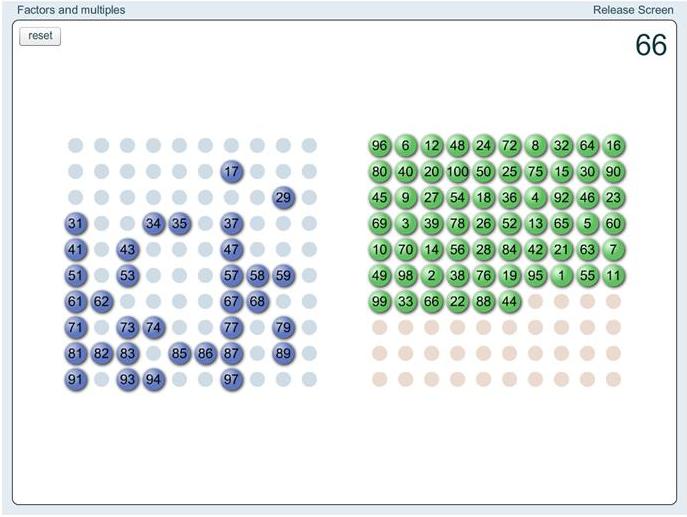
James from Ridgewood School went one better with this chain of 66 numbers:

solution

Linda from Bohunt School used 68 numbers:

link

A group of Year 9 students from The Perse School for Girls in Cambridge worked together and managed an even longer chain of 71 numbers:

Chain of 71

And Claire, of Blackheath High School in London, has managed to improve on that with this chain of 73 numbers:

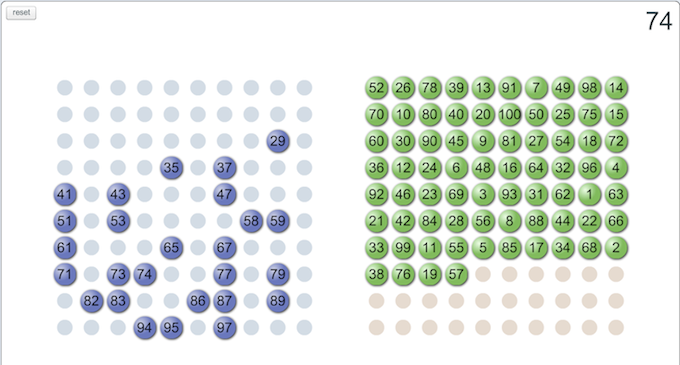
Jacky, from Princethorpe College in Rugby, has managed to go one better with a chain of 74:

Jesse from Moriah College in Sydney, Australia also managed a chain of 74 numbers:

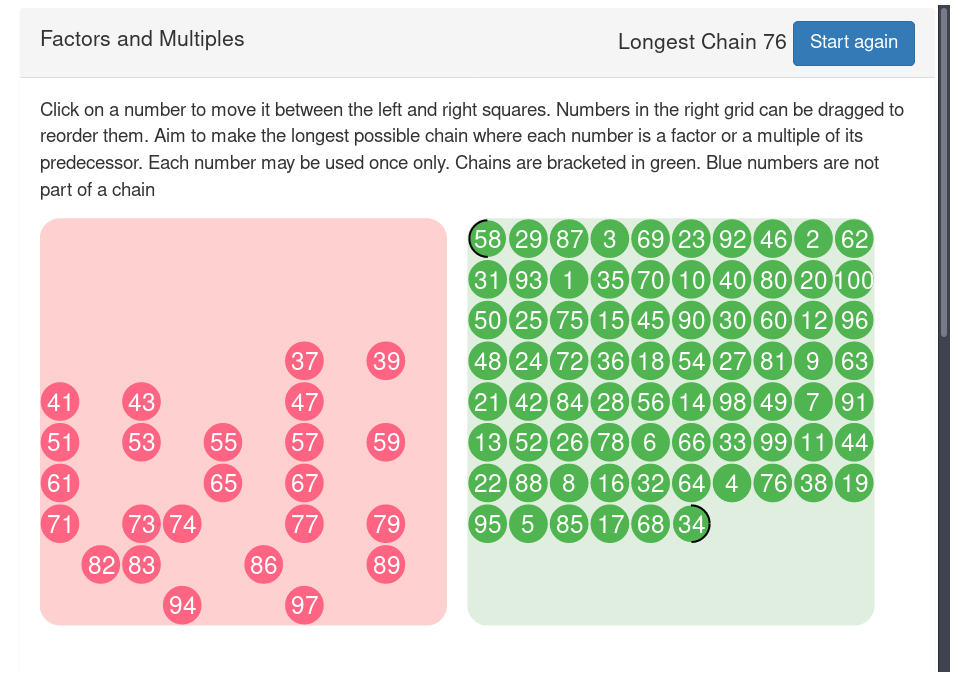
Izaak, from Hills Rd VI Form College, used some computer programming techniques to try to make the longest chain he could. Below is his 76 long chain. If you want to read about his program and see his code, he has shared it on Github.

We've also received a few chains using 77 numbers!

Millie from Hursthead Junior School in the UK sent in this solution:

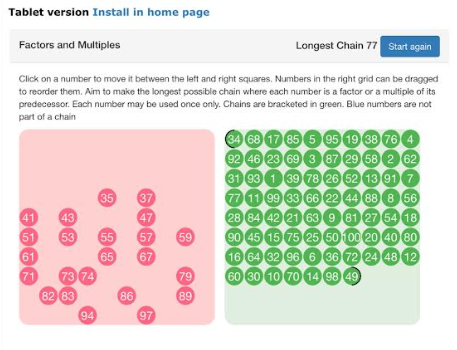
Well done Millie! Jakob from Haberdashers Adams School in the UK also found the same solution.

We received a different chain of 77 numbers from Michelle at The Coopers' Company and Coborn School in the UK:

Ahaan from Newcastle Under Lyme School found the same chain, and Sarisha from Newcastle Under Lyme School found this chain of 77 numbers:

Can you see how this is related to Michelle's chain above?

Miraya from Heckmondwike Grammar School shared these strategies for developing longer and longer chains:

First of all not use the numbers one to ten since they are the common multiples or factors of any given number between one to hundred. Next , the other thing you have to remember is to try not to use prime numbers as the prime numbers have factors that are only 1 you can use multiples but they will not last very long. Try to find out the common multiple factors as well. Also, try to use the bigger numbers since they will have the most factors. Finally , try to use the common numbers with the most multiples under 100 for example 10 or 11 since they have multiples in every ten numbers.

Well done to all of you, and to those of you who found chains of 77, congratulations, you have found the longest chain.

Thank you to Josh Tutin who sent us a link to The On-Line Encyclopedia of Integer Sequences, and the table below, which lists the longest possible chains using the numbers from 1 to n, where n is smaller than 200. Click to see the table. Can you produce some of these chains?

n	Longest chain
1	1
2	2
3	3
4	4
5	4
6	6
7	6
8	7
9	8
10	9
11	9
12	11
13	11
14	12
15	13
16	14
17	14
18	16
19	16
20	17
21	18
22	19
23	19
24	21
25	21
26	22
27	23
28	24
29	24
30	26
31	26
32	27
33	28
34	28
35	29
36	30
37	30
38	30
39	31
40	32
41	32
42	34
43	34
44	36
45	37
46	37
47	37
48	39
49	39
50	41
51	42
52	43
53	43
54	44
55	45
56	46
57	47
58	47
59	47
60	49
61	49
62	49
63	50
64	51
65	51
66	53
67	53
68	54
69	55
70	57
71	57
72	58
73	58
74	58
75	59
76	60
77	61
78	63
79	63
80	64
81	65
82	65
83	65
84	66
85	66
86	66
87	67
88	69
89	69
90	70
91	71
92	72
93	73
94	73
95	73
96	74
97	74
98	75
99	76
100	77
101	77
102	79
103	79
104	81
105	82
106	82
107	82
108	83
109	83
110	85
111	86
112	87
113	87
114	89
115	89
116	90
117	91
118	91
119	92
120	93
121	93
122	93
123	94
124	95
125	95
126	96
127	96
128	97
129	97
130	99
131	99
132	100
133	100
134	100
135	101
136	103
137	103
138	105
139	105
140	106
141	106
142	106
143	106
144	107
145	108
146	108
147	110
148	111
149	111
150	112
151	112
152	113
153	115
154	116
155	116
156	117
157	117
158	117
159	117
160	118
161	119
162	120
163	120
164	121
165	123
166	123
167	123
168	124
169	124
170	126
171	127
172	128
173	128
174	131
175	132
176	133
177	133
178	133
179	133
180	134
181	134
182	135
183	135
184	137
185	137
186	139
187	139
188	140
189	141
190	142
191	142
192	143
193	143
194	143
195	144
196	145
197	145
198	147
199	147
200	148

There are many different possible longest chains - at the OEIS you can see the 12 different possible chains, of length 11 (the longest chain - see below), which use numbers from 1 to 13.

Josh then investigated numbers which should not go in chains, and exploited these to develop some winning strategies for playing the game. Josh also created some longest-possible chains using numbers 1 to n, for several values of n. Click here to see Josh's work.

Number and algebra

Geometry and measure

Probability and statistics

Working mathematically

Advanced mathematics

For younger learners

Factors and Multiples Game

Related Collections

You may also like

Pebbles

Bracelets

Sweets in a Box