Skip over navigation
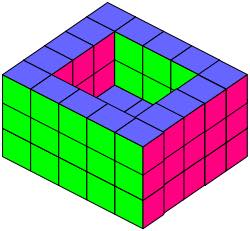
Here is a small open box made out of little cubes.
It is 5 cubes wide, 5 cubes long and 3 cubes high.
It is just 1 cube thick everywhere.
Work out how many little cubes were used to make the open box in the picture.
Challenge 2
Challenge 3
You have 112 little cubes.
Imagine that you are asked to make more open boxes which are 1 cube thick, always with a width between 3 and 5 cubes inclusive, the length between 10 and 20 cubes inclusive and the height of 2 or 3 cubes.
What size open boxes could you make if you have to use all 112 cubes?
Challenge 4
If open boxes have to be 1 cube thick, have a width of 3, 4 or 5 cubes inclusive, the length between 10 and 20 cubes inclusive and the height of 2 or 3 cubes (as in the previous challenge), what sizes use exactly 144, 145, 147 and 148 little cubes?
This activity was designed for the 2015 Young Mathematicians' Award so it might be a particularly useful activity for a small group of your highest-attaining pupils to work on. It is a useful vehicle for developing systematic approaches. It can be used as an activity to encourage children to explain in written or spoken words what it is they have done.
Working as a team of four pupils with a maximum of 20 cubes for them to use for the remaining challenges encourages a very thoughtful and systematic approach.
Tell me about how you are getting a solution for the challenge you are working on.
(The main thing when encouraging the pupils to use their skills is to avoid saying things about what you notice and directing them in your way of attempting a solution.)
The Rise and Fall of Curiosity - the extract from 23.50 to 37.15 on adult encouragement and teacher behaviour is particularly worth viewing
The Hungry Mind: The Origins of Curiosity - the extract from 8.22 to 12.29 on children asking questions is especially useful.
Open Boxes
Age 7 to 11
Challenge Level 
Open Boxes
Here is a small open box made out of little cubes.
It is 5 cubes wide, 5 cubes long and 3 cubes high.
It is just 1 cube thick everywhere.
Challenge 1
Work out how many little cubes were used to make the open box in the picture.
Challenge 2
If you were going to make a bigger box that is 5 cubes wide, 12 cubes long, 3 cubes high and just 1 cube thick, how many little cubes would you need?
Challenge 3
You have 112 little cubes.
Imagine that you are asked to make more open boxes which are 1 cube thick, always with a width between 3 and 5 cubes inclusive, the length between 10 and 20 cubes inclusive and the height of 2 or 3 cubes.
What size open boxes could you make if you have to use all 112 cubes?
Challenge 4
If open boxes have to be 1 cube thick, have a width of 3, 4 or 5 cubes inclusive, the length between 10 and 20 cubes inclusive and the height of 2 or 3 cubes (as in the previous challenge), what sizes use exactly 144, 145, 147 and 148 little cubes?
Why do this problem?
This activity was designed for the 2015 Young Mathematicians' Award so it might be a particularly useful activity for a small group of your highest-attaining pupils to work on. It is a useful vehicle for developing systematic approaches. It can be used as an activity to encourage children to explain in written or spoken words what it is they have done.This activity may be used to encourage pupils to ask "I wonder what would happen when . . ." and use their curiosity to take things further with cuboid shapes. If you are interested in pursuing Curiosity further then see the Further Note at the bottom of this page.
Possible approach
Since this activity is aimed at the most confident mathematicians, there will not be much that you have to do to introduce it apart, perhaps, from making the first open box and letting the pupils declare their different ways of answering the first challenge.Working as a team of four pupils with a maximum of 20 cubes for them to use for the remaining challenges encourages a very thoughtful and systematic approach.
Key questions
Tell me about how you are getting a solution for the challenge you are working on.(The main thing when encouraging the pupils to use their skills is to avoid saying things about what you notice and directing them in your way of attempting a solution.)
Possible extension
Learners might like to try the other two challenges that were part of the Young Mathematicians' Award 2015: Dice Stairs and Centred Squares.
Further note
You may be interested in the following talks given by Professor Susan Engels, which focus on encouraging curiosity and are available on YouTube:The Rise and Fall of Curiosity - the extract from 23.50 to 37.15 on adult encouragement and teacher behaviour is particularly worth viewing
The Hungry Mind: The Origins of Curiosity - the extract from 8.22 to 12.29 on children asking questions is especially useful.
You may also like
Geoboards
This practical challenge invites you to investigate the different squares you can make on a square geoboard or pegboard.
