Skip over navigation
Or search by topic
Number and algebra
Geometry and measure
Probability and statistics
Working mathematically
Advanced mathematics
For younger learners
Circular Area
Age 7 to 11
Challenge Level 





- Problem
- Getting Started
- Student Solutions
- Teachers' Resources
We had a few solutions sent in about this task, thank you.
Ayla from Woodlea Junior School wrote:
I think that Jay is right because he is using all of the circle and he's not using any of the space outside of the circle so it would probably end up more accurate. Even though he's splitting it up, it's still the same circle and it's not any of the outside space.
Isaac from Tockwith Primary Academy wrote:
You need to find the radius of the circle first. (The distance from the edge to the middle.)
Then you need to square the length of the radius.
(To square a number, you have to times it by itself, e.g. 4x4=16 5x5=25)
Then, you times your answer by 3.14 (scientists call it ð›‘, pi). Pi isn't just 3.14 it goes further, meaning it has more decimal places.
When you have done so, you will be left with the area.
Ben from Channel Christian School in Tasmania sent in the following:
I got the diameter of the circle which was 72mm then got the radius which was 36mm. After that, I multiplied the radius of the circle with pi and the answer was 406.9 mm ² then I converted mm into cm and it was 40.69cm ².
AREA EQUALS PI TIMES RADIUS SQUARED
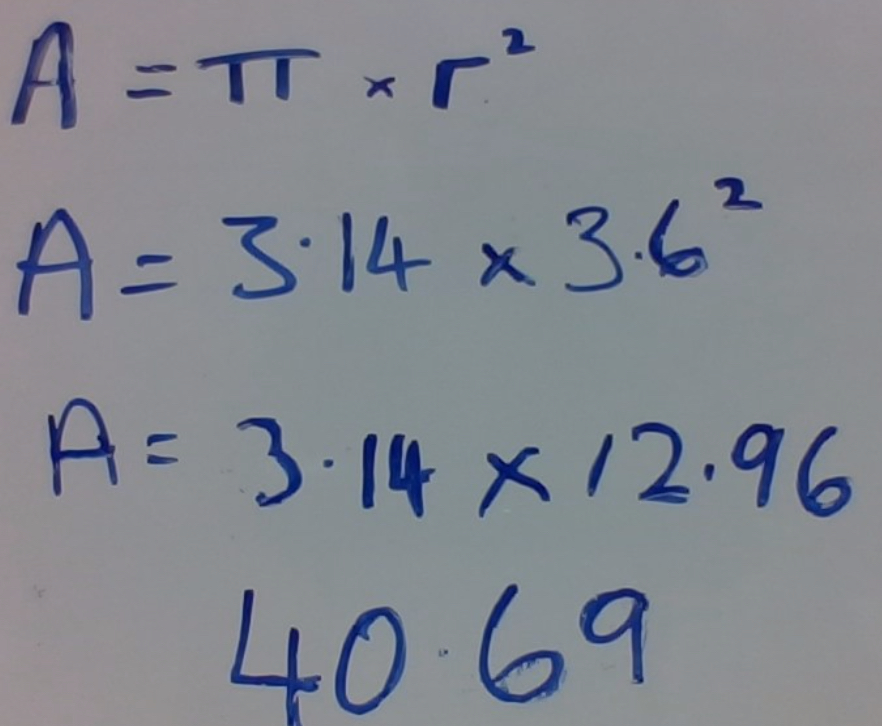
Thank you are your thoughts about circular areas.
Ayla from Woodlea Junior School wrote:
I think Fran's solution was better because it would probably give the most accurate answer.
Georgia from Woodlea Junior School explains:I think that Jay is right because he is using all of the circle and he's not using any of the space outside of the circle so it would probably end up more accurate. Even though he's splitting it up, it's still the same circle and it's not any of the outside space.
Isaac from Tockwith Primary Academy wrote:
You need to find the radius of the circle first. (The distance from the edge to the middle.)
Then you need to square the length of the radius.
(To square a number, you have to times it by itself, e.g. 4x4=16 5x5=25)
Then, you times your answer by 3.14 (scientists call it ð›‘, pi). Pi isn't just 3.14 it goes further, meaning it has more decimal places.
When you have done so, you will be left with the area.
Ben from Channel Christian School in Tasmania sent in the following:
I got the diameter of the circle which was 72mm then got the radius which was 36mm. After that, I multiplied the radius of the circle with pi and the answer was 406.9 mm ² then I converted mm into cm and it was 40.69cm ².
AREA EQUALS PI TIMES RADIUS SQUARED
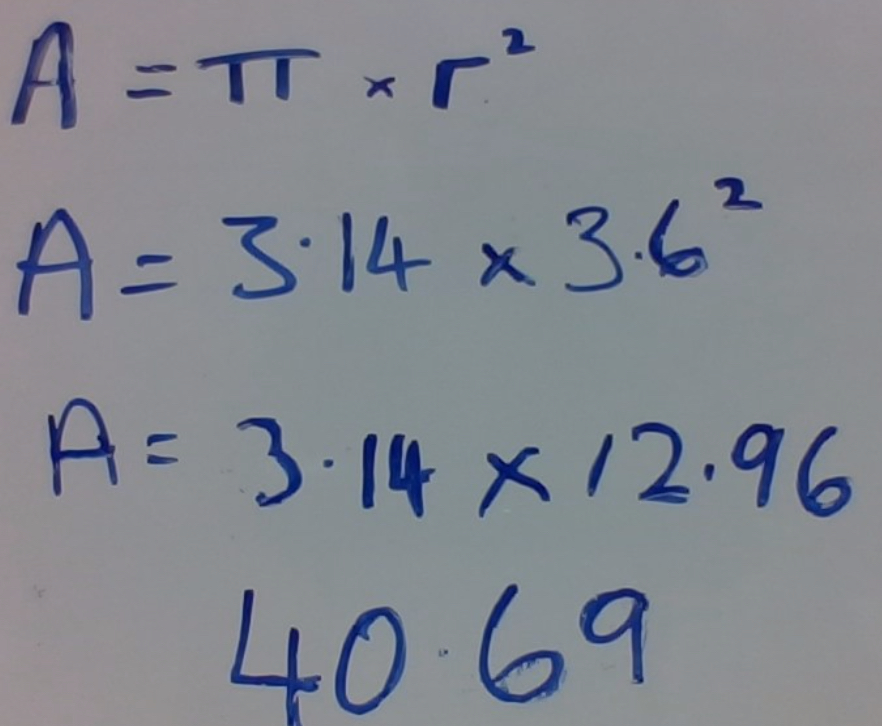
Thank you are your thoughts about circular areas.

