Cyclic Quadrilaterals Proof

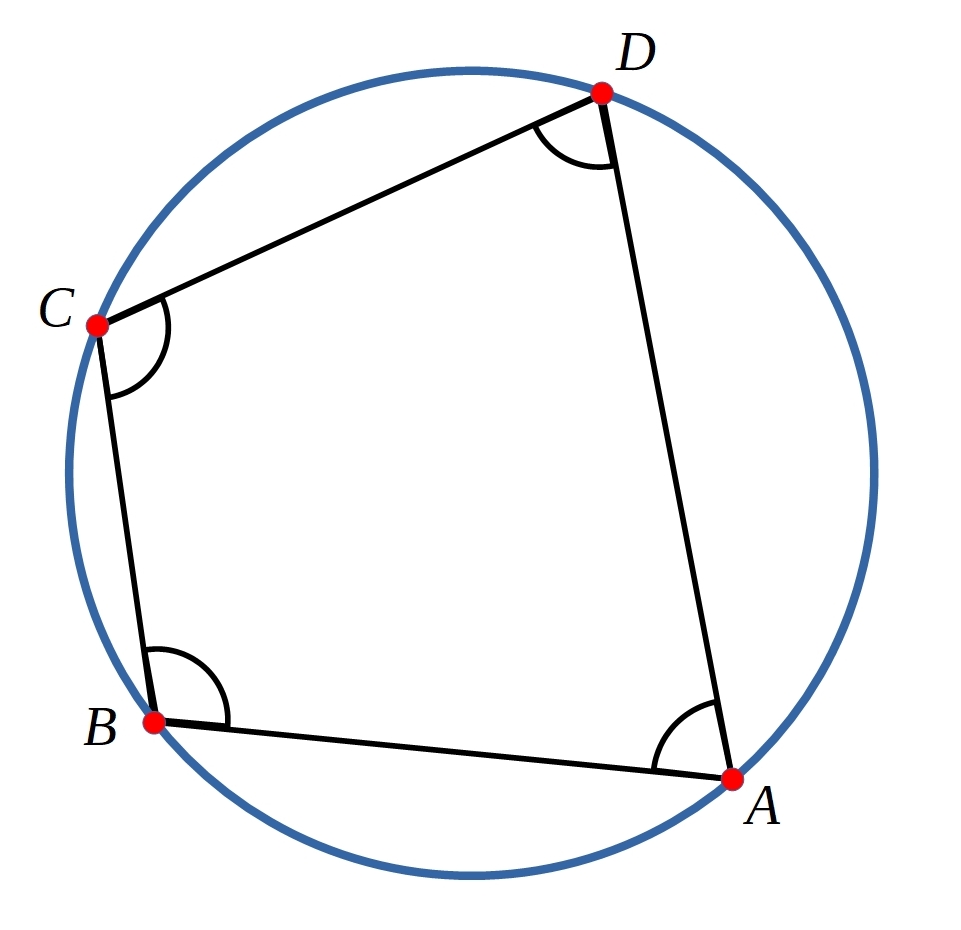This problem follows on from Cyclic Quadrilaterals

Sketch a circle and choose four points at random to form a quadrilateral. 


Can you prove that the opposite angles of your quadrilateral add to $180^\circ$?





Click below to see a diagram that might help you to prove it.

  

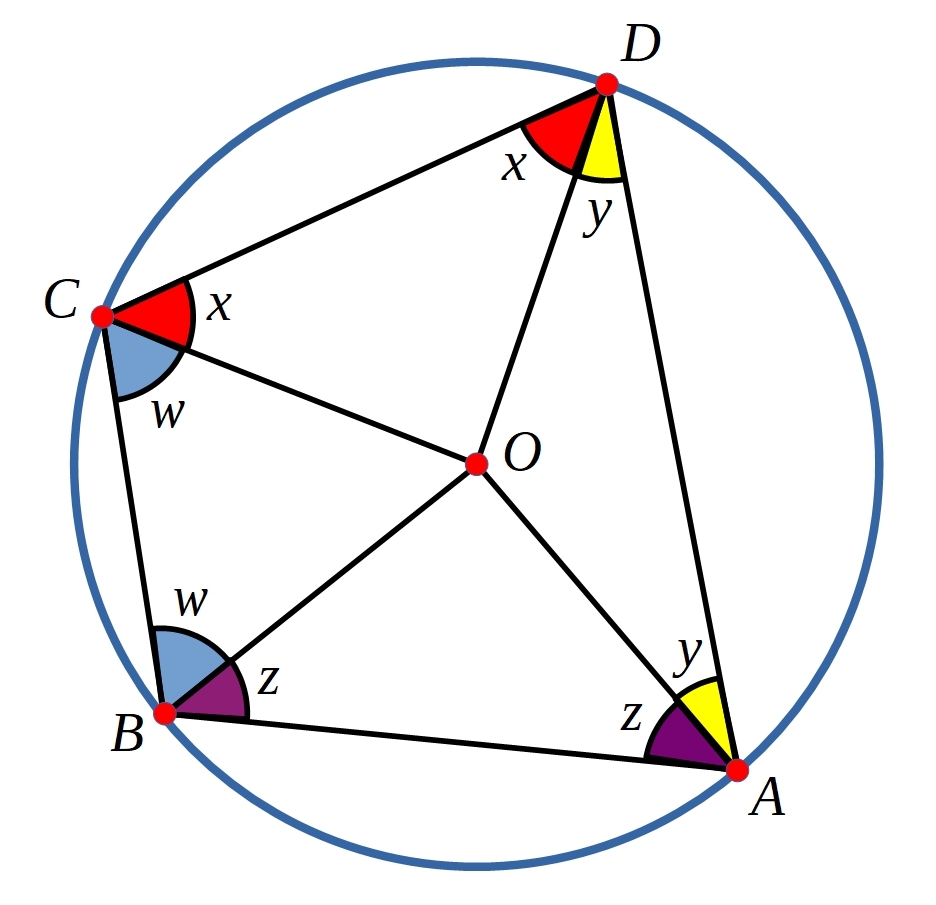
Quadrilaterals whose vertices lie on the edge of a circle are called Cyclic Quadrilaterals.





Extension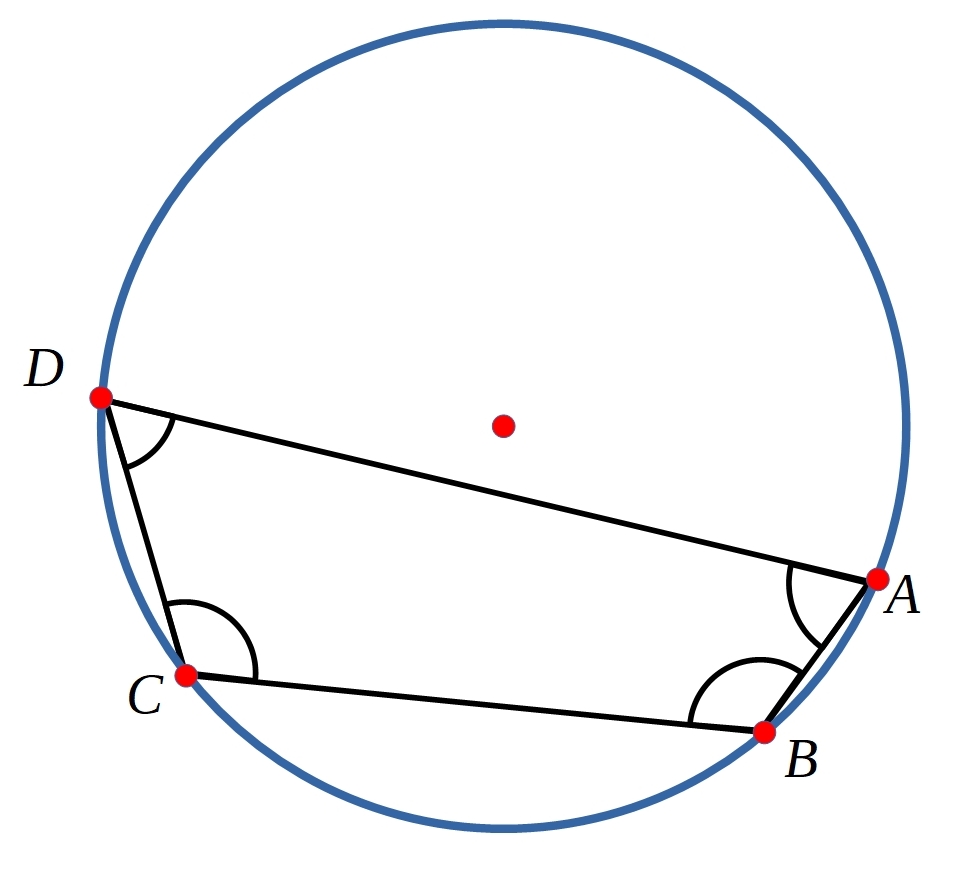

Can you prove that when the centre of the circle is outside the cyclic quadrilateral, the opposite angles also add to $180^\circ$?

You may wish to draw some examples on
9, 10, 12, 15 and 18 dot circles.







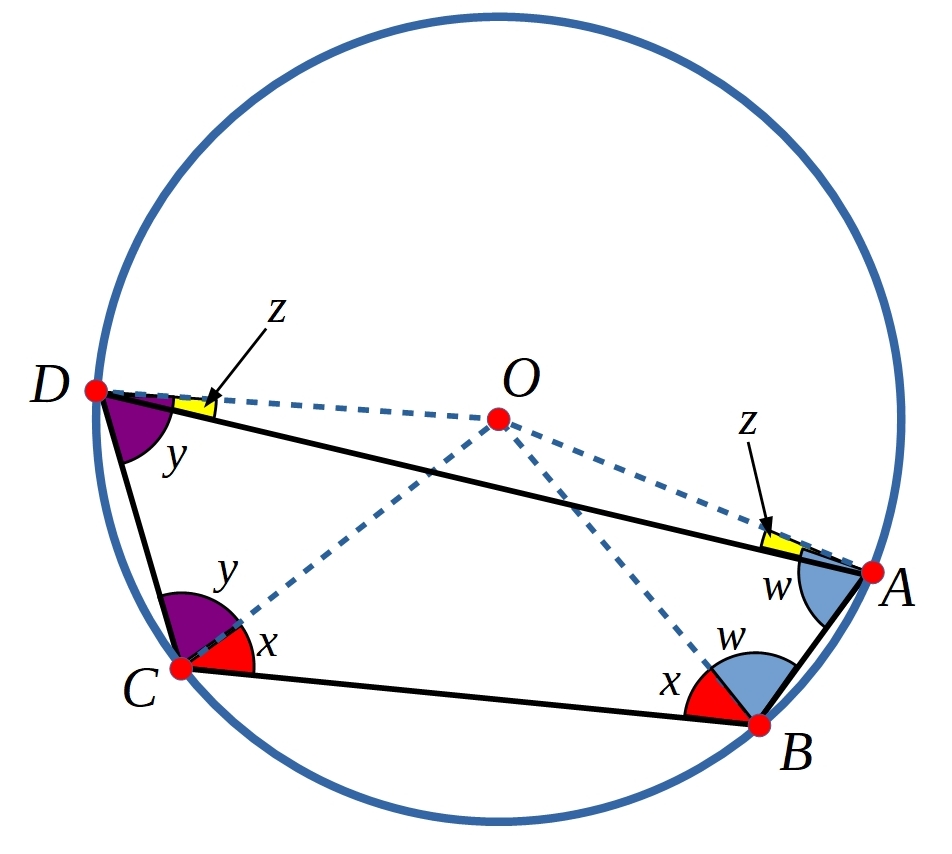
Click below to see a diagram that might help you to prove that the opposite angles of cyclic quadrilaterals add to $180^\circ$, when the centre of the circle is outside the cyclic quadrilateral.

 

You may be interested in the other problems in our Sharpen your Skills Feature.